Which of the following statement(s) is /are correct ?
(i) is negative, then the reaction is spontaneous and proceeds in the forward direction.
(ii) is positive, then reaction is non-spontancous
(iii) is 0, then reaction is at equilibrium
I, ii and iii are correct
I and ii
ii and iii are correct
only iii is correct
The correct Answer is:A
Topper's Solved these Questions
Similar Questions
Knowledge Check
If for a reaction ΔH is negative and ΔS is positive then the reaction is
Aspontaneous at all temperaturesBnon-spontaneous at all temperaturesCspontaneous only at high temperaturesDspontaneous only at low temperatureIf ΔH>0 and ΔS>0, the reaction proceeds spontaneously when :-
AΔH>0BΔH<TΔSCΔH=TΔSDNoneFor an exothermic reaction to be spontaneous (ΔS = negative)
ATemperature must be highBTemperature must be zeroCTemperature may have any magnitudeDTemperature must be low
If ΔH>0 and ΔS>0, the reaction proceeds spontaneously when :-
View SolutionFor an exothermic reaction to be spontaneous (ΔS = negative)
View SolutionFree enegry , G=H−TS, is state function that indicates whther a reaction is spontaneous or non-spontaneous. If you think of TS as the part of the system's enegry that is disordered already, then (H−TS) is the part of the system's energy that is still ordered and therefore free to cause spontaneous change by becoming disordered.
Also, ΔG=ΔH−TΔS From the second law of thermodynamics, a reaction is spontaneous if ΔtotalS is positive, non-spontaneous if ΔtotalS is negative, and at equilibrium if Δ'→talS is zero.
Since, −TΔS=ΔG and since ΔG and ΔS have opposite sings, we can restate the thermodynamic criterion for the spontaneity of a reaction carried out a constant temperature and pressure.
IF ΔG<0, the reaction is spontaneous.
If ΔG>0, the reaction is non-spontaneous.
If ΔG=0, the reaction is at equilibrium.
Read the above paragraph carefully and answer the following questions based on the above comprehension.
For the spontaneity of a reaction, which statement is true?
View SolutionFree enegry , G=H−TS, is state function that indicates whther a reaction is spontaneous or non-spontaneous. If you think of TS as the part of the system's enegry that is disordered already, then (H−TS) is the part of the system's energy that is still ordered and therefore free to cause spontaneous change by becoming disordered.
Also, ΔG=ΔH−TΔS From the second law of thermodynamics, a reaction is spontaneous if ΔtotalS is positive, non-spontaneous if ΔtotalS is negative, and at equilibrium if Δ'→talS is zero.
Since, −TΔS=ΔG and since ΔG and ΔS have opposite sings, we can restate the thermodynamic criterion for the spontaneity of a reaction carried out a constant temperature and pressure.
IF ΔG<0, the reaction is spontaneous.
If ΔG>0, the reaction is non-spontaneous.
If ΔG=0, the reaction is at equilibrium.
Read the above paragraph carefully and answer the following questions based on the above comprehension.
For the spontaneity of a reaction, which statement is true?
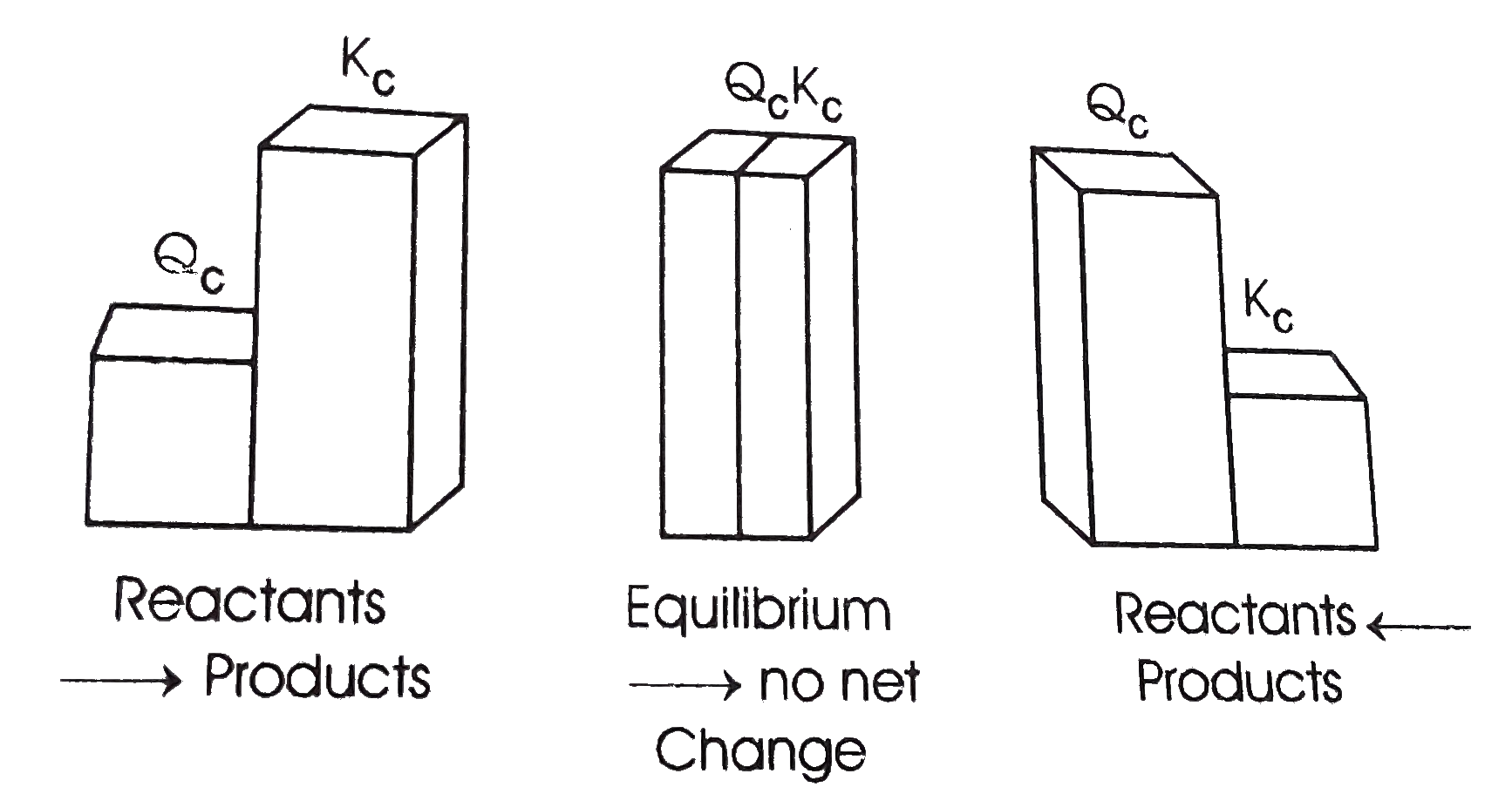
View SolutionThe reaction which is in dynamic equilibrium, ensured us, that the reaction is reversible . But if that the reaction is in equilibrium. The reaction quotient predict either the reversible reaction is in equilibrium or tries to achieve equilibrium. In those reactions which have not achieved equilibrium, we obtain reaction quotient Qc in place of equilibrium constant (Kc) by substituting the concentration of reactant and product at the time, at whih we have to calculate the value of Qc . To determine the direction at which the net reaction will proceed to achieve equilibrium, we compare values of Qc and Kc. The three possible cases are shown as comparison of Kc and Qc in the following figures.
Change in Gibbs free energy, i.e., ΔG is the driving force of any reaction.
For spontaneous reaction , ΔG=−ve
For non-spontaneous reaction , ΔG=+ve
For reaction at equilibrium , ΔG=0
Thermodynamically, we know that
ΔG=ΔG∘+RTlnQ, where Q is reaction quotient and ΔG∘= change in Gibbs energy at standard condition.
For equilibrium A(g)⇔B(g)(Keq=1.732) If the pressure of the system [varied by introducing a stream of A(g) and B (g) is represented by the curve at constant temperature T.
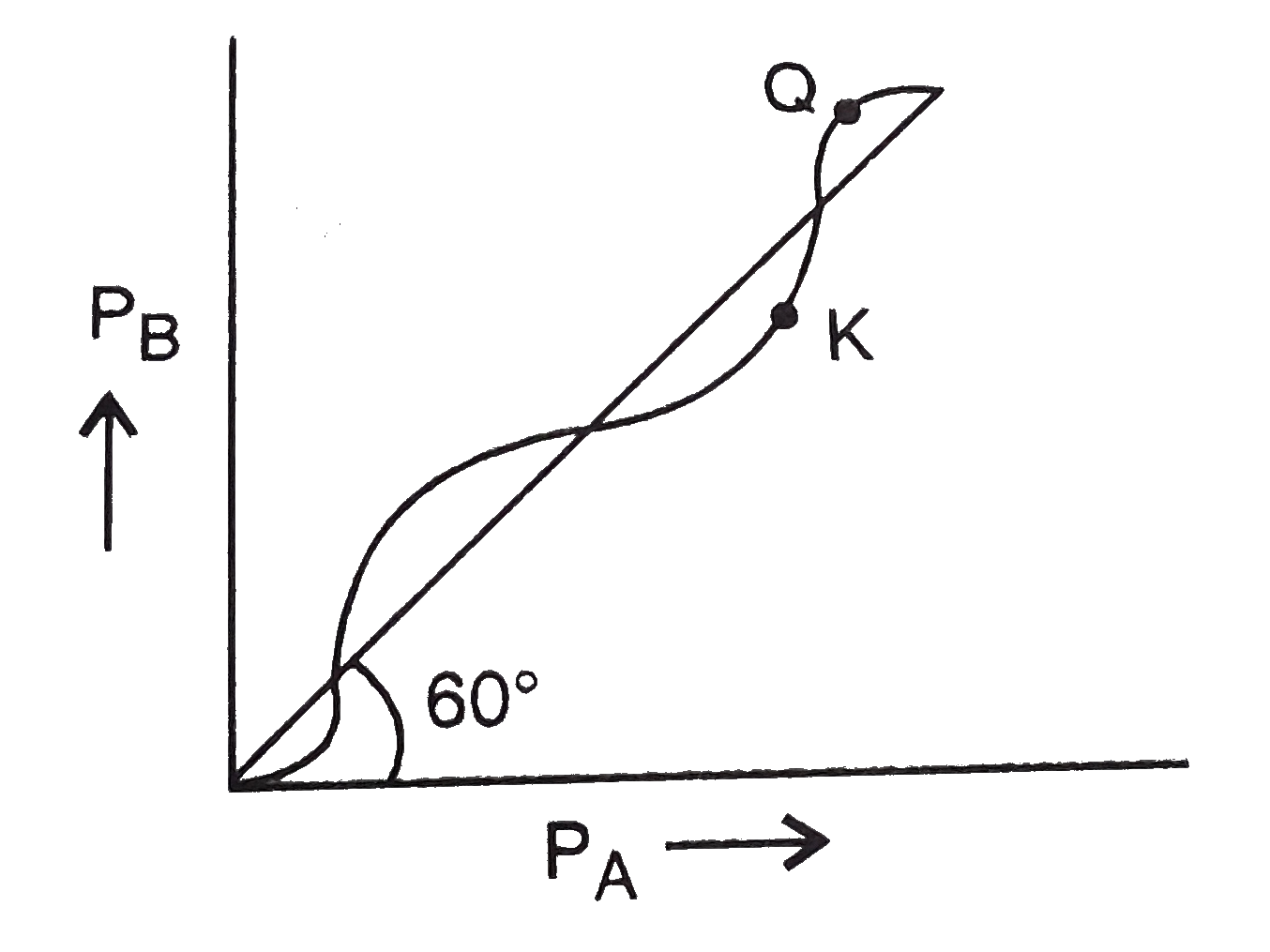
Which of the following is the correct statement at point R in the figure above ?
View Solution
