In the given figure,AB=4cmandBD=4√3cm. Then the relation between Δ[areaof△ADC]andS(shaded area bounded by three semicircles) is:
Step by step video & image solution for In the given figure, AB =4 cm and BD=4sqrt3 cm. Then the relation between Delta[area of triangle ADC] and S(shaded area bounded by three semicircles) is: by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
If the sides of a triangle are 5 cm, 4 cm and √41 cm, then the area of the triangle is
A20 cm2B(5+4+√41)cm2C5+4+√412cm2D10 cm2In the adjoining figure there are three semicircles in which BC=6 cm and BD=6√3 cm. What is the area of the shaded region ( in cm) :
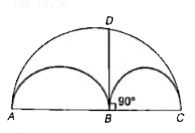 A12πB9πC27πD28π
A12πB9πC27πD28πIf the angle A, B and C of a triangle ABC are in A.P and a:b=1: √3 If c=4 cm then the area (in sq. cm) of this triangle is :
A4√3B2√3C2√3D4√3
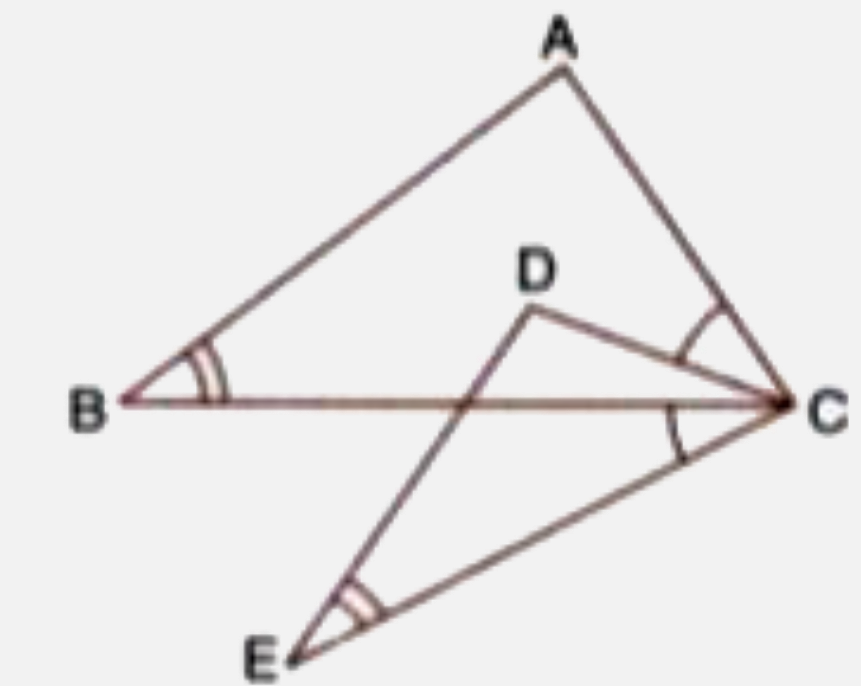
In the given figure, ∠B=∠E,∠ACD=∠BCE,AB=10.4 cm and DE=7.8cm. Find the ratio between areas of the ΔABCandΔDEC.
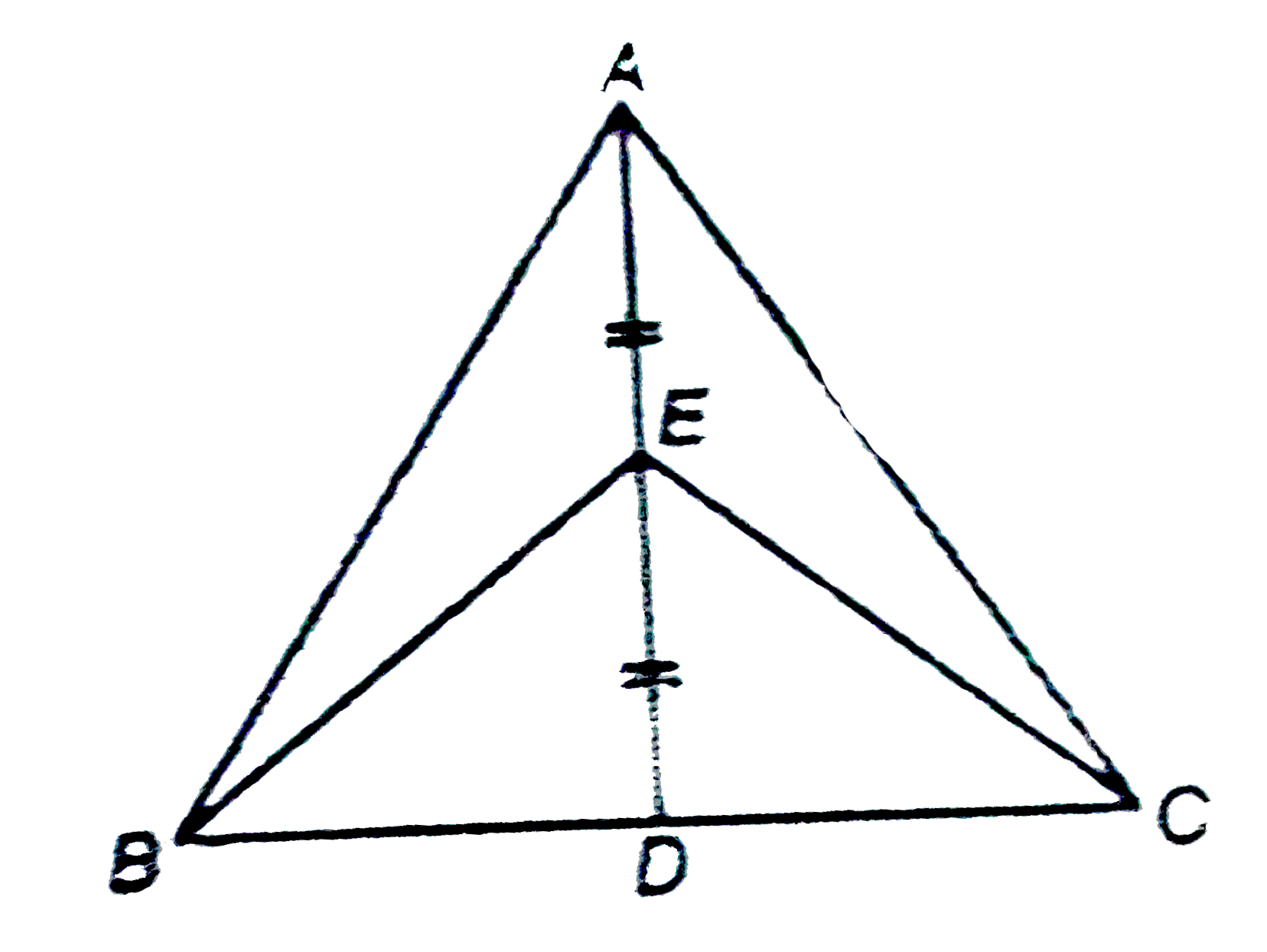
View SolutionIn the adjoining figure, BD = DC and AE = ED. Prove that
area of ΔACE=14 area of ΔABC
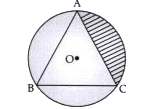
View SolutionIn the given figure, ABC is an equilateral triangle inscribed in a circle of radius 4 cm with centre O, then the area of the shaded region is :
View SolutionIf the angle A, B and C of a triangle ABC are in A.P and a:b=1: √3 If c=4 cm then the area (in sq. cm) of this triangle is :
View SolutionIf the angle A, B and C of a triangle ABC are in A.P and a:b=1: √3 If c=4 cm then the area (in sq. cm) of this triangle is :
View Solution