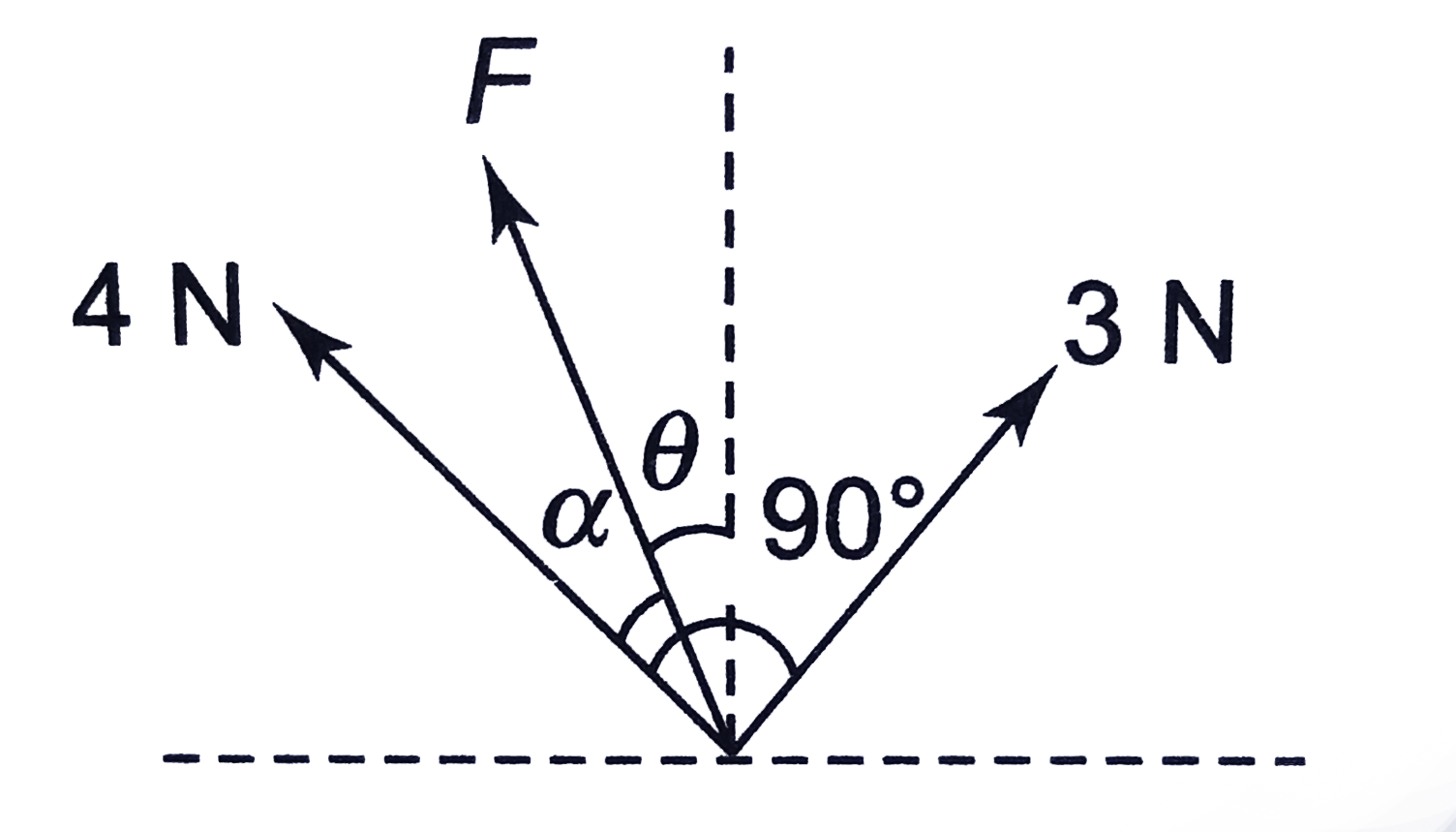
A force 3N is acting along the NE and another force 4N is acting the NW. Find the angle made by the resultant force with north.
Method I (component approach) :
Fx=3cos45∘−4cos45∘=−cos45∘=−1√2
Fy=3sin45∘+4sin45∘=7sin45∘=7√2
tanθ=FxFy=17
θ=tan−1(17)
The resultant force makes an angle tan−1(17) with north, left of it.
Method II : Since there are only two forces , we can use the law of parallelogram
Since 4>3 , the resultant will be towards 4N.
tanα=34⇒α=tan−1(34)
θ+α=45∘
θ=45∘−tan−1(34)
(If the value of tan−1(3/4)=37∘ given)
θ=45∘−37∘=8∘
The angle between by the resultant force with north (left of it ) is 8∘.

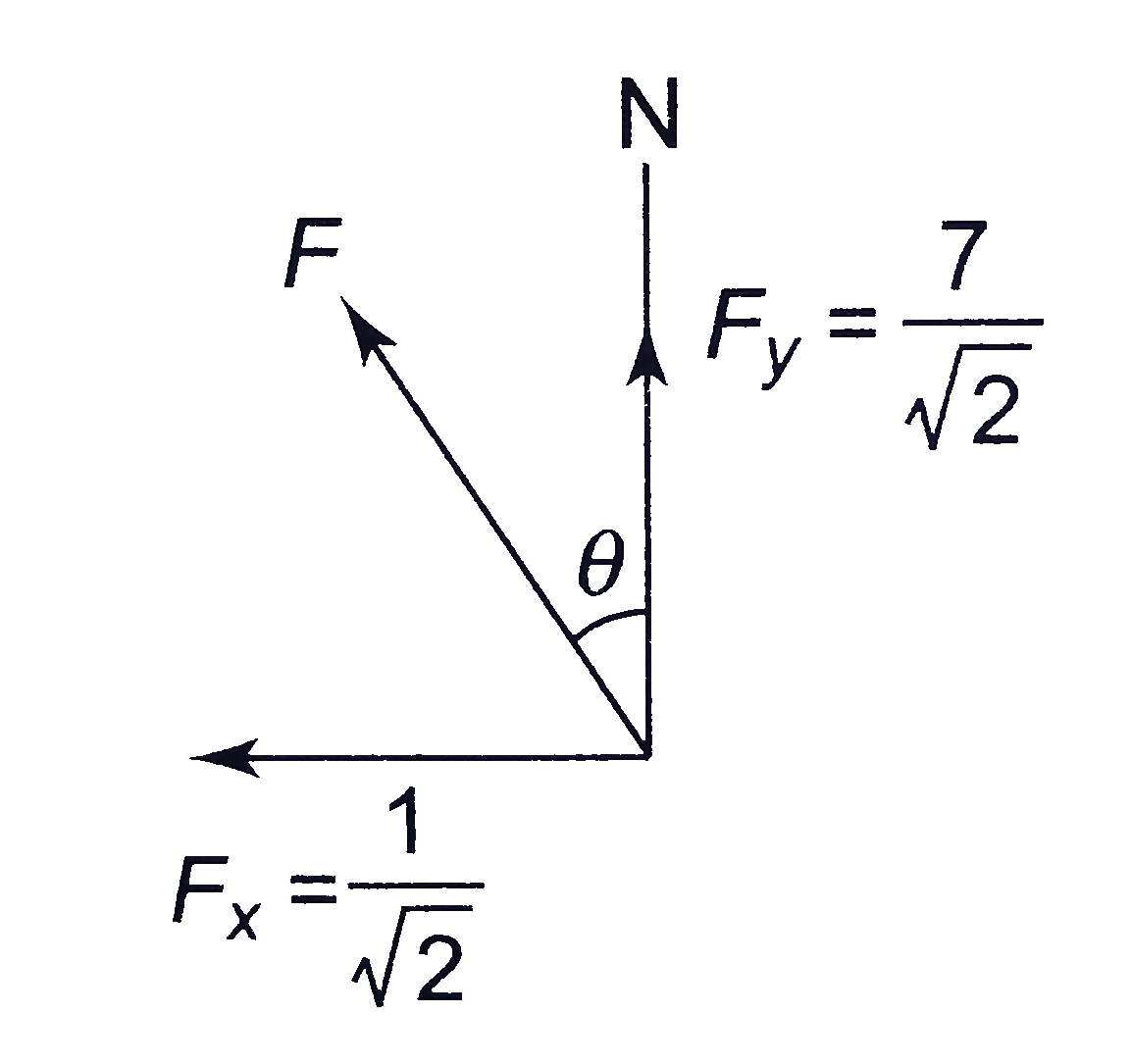
Topper's Solved these Questions
Similar Questions
A magnetic compass needle is subjected to a force of 0.02 N acting due north and a force of 0.04 N acting due west. Calculate the resultant force on the needle and the direction in which it rests.
View SolutionTwo constant forces 4 N and 5 N act on a body in opposite directions .Find the resultant force on the body
View Solution
Knowledge Check
Two equal forces acting at 60∘ to each other. Find the forces if resultant force is 300 N.
A155.3 NB173.2 NC162.4 NDNone of theseTwo equal forces acting at 60∘ to each other. Find the forces if resultant force is 300 N.
A155.3NB173.2 NC162.4 NDNone of theseTwo non-collinear forces, one of 10 N and another of 6 N act upon a body. The directions of the forces are unknown. The resultant force on the body is :
Abetween 6 and 10 NBbetween 4 and 16 NCmore than 6 NDmore than 10 N
Two constant forces 4 N and 5 N act on a body in opposite directions .Find the resultant force on the body
View SolutionTwo constant forces 4 N and 5 N act on a body in opposite directions. Find the resultant force on the body.
View SolutionA force of 4 N is acting at an angle of 30∘ to the horizontal. Find its component along axeses.
View SolutionIf two forces are acting at a point such that the magnitude of each force is 2 N and the magnitude of their resultant is also 2 N, then the angle between the two forces is
View SolutionIf two forces are acting at a point such that the magnitude of each force is 2N and the magnitude of their resultant is also 2N, then the angle between the two forces is
View Solution
