If a particle moves from the point A(1,2,3) to the point B(4,6,9), its displacement vector be
ˆi+ˆj+ˆk
2ˆi+3ˆj+4ˆk
3ˆi+4ˆj+6ˆk
3ˆi+4ˆj+5ˆk
The correct Answer is:C
→r1=I+2j+3k,→–––(r2)=4i+6j+9k
→d=→r2−→r1=3I+4j+6k
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
If a particle moves from point P(2,3,5) to point Q(3,4,5). Its displacement vector be
Aˆi+ˆj+10ˆkBˆi+ˆj+5ˆkCˆi+ˆjD2ˆi+4ˆj+6ˆk - Question 2 - Select One
If a particle is displaced from the point A(5, -5, -7) to the point B(6, 2, -2) under the influence of the forces →P1=10ˆi−ˆj+11ˆk,→P2=4ˆi+5ˆj+6ˆk,→P3=−2ˆi+ˆj−9ˆk, then the work done is :
A87B85C81Dnone of these - Question 3 - Select One
If a particle is displaced from the point A(5, -5, -7) to the point B(6, 2, -2) under the influence of the forces →P1=10ˆi−ˆj+11ˆk,→P2=4ˆi+5ˆj+6ˆk,→P3=−2ˆi+ˆj−9ˆk, then the work done is :
A87B85C81Dnone of these
A force ¯¯¯F=(3 hati-4 hatj +b hat k)N is acting on the particle which is moving from point A ( 0-1, 1) m to the point B(2, 2 3) m If net work done by the force on the particle is zero then value of b is
View SolutionIn the diagram shown, a particle is moving from the point A to the point B. Length of each arrow is 1 m and the particle is moving at a constant speed of 1ms−1 . What is the displacement of the particle?
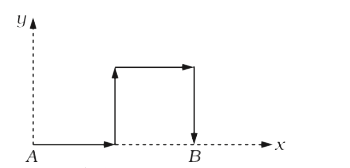 View Solution
View SolutionIn a two diamensional motion of a particle, the particle moves from point A, with position vector →r1 to point B, with position vector →r2. If the magnitudes of these vectors are, respectively, r1=3 and r2=4 and the angles they make with the x-axis are θ1=75∘ and θ2=15∘, respectively, then find the magnitude of the displacement vector.
 View Solution
View SolutionIf the constant due to which it is displaced from a point on a particle due to which it is displaced from a point A(4,−3,−2) to a point B(6,1,−3) then the work done by the forces is
View SolutionIn a two-dimensional motion of a particle, the particle moves from point A of position vector →r1, to point B of vectors are , respectively, r1=3andr2=4 and the angles they make with the x axis are θ1=75∘andθ2=15∘, respectively, then find the magnitude of the displacement vector.
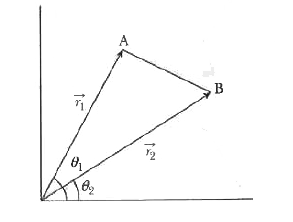 View Solution
View Solution

