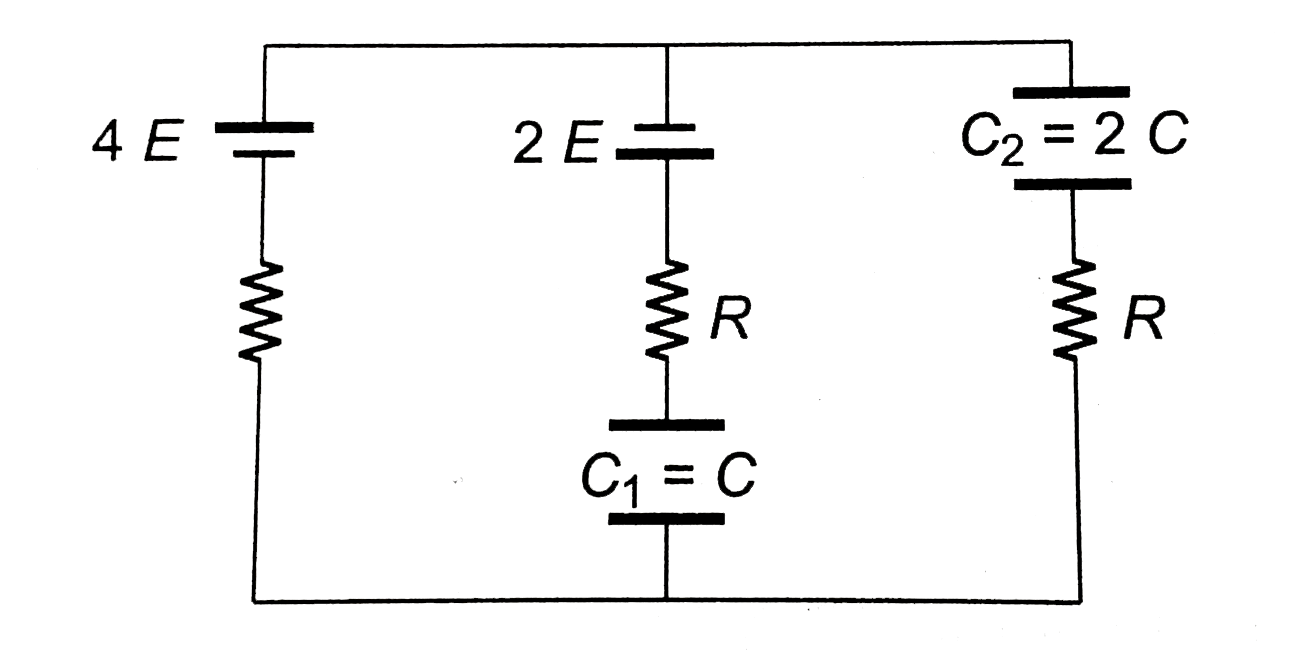
The ratio of energy stored in capacitors C1 and C2
98
89
56
65
The correct Answer is:A
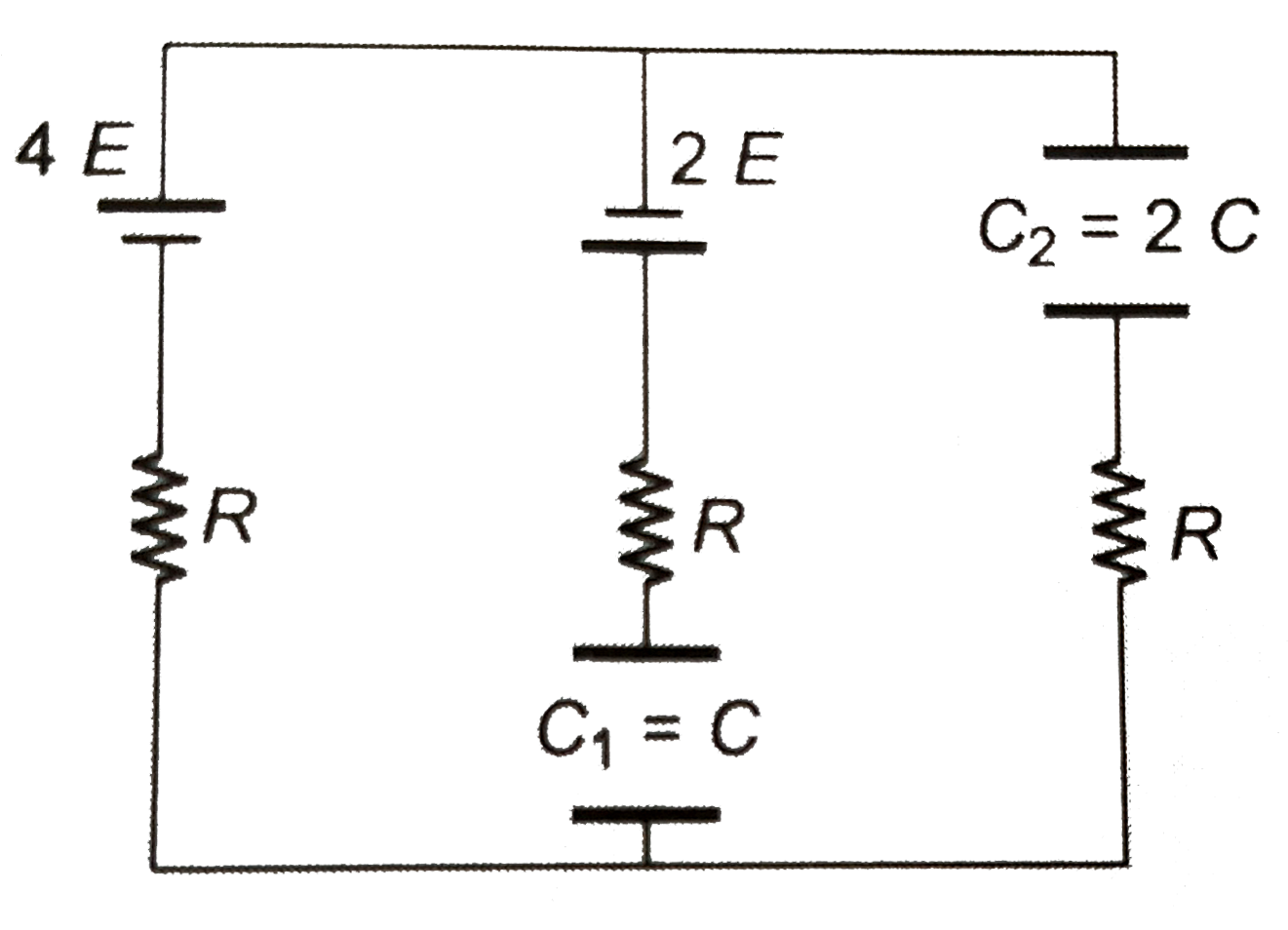
The resistances each R are redundant, no current flows through them.
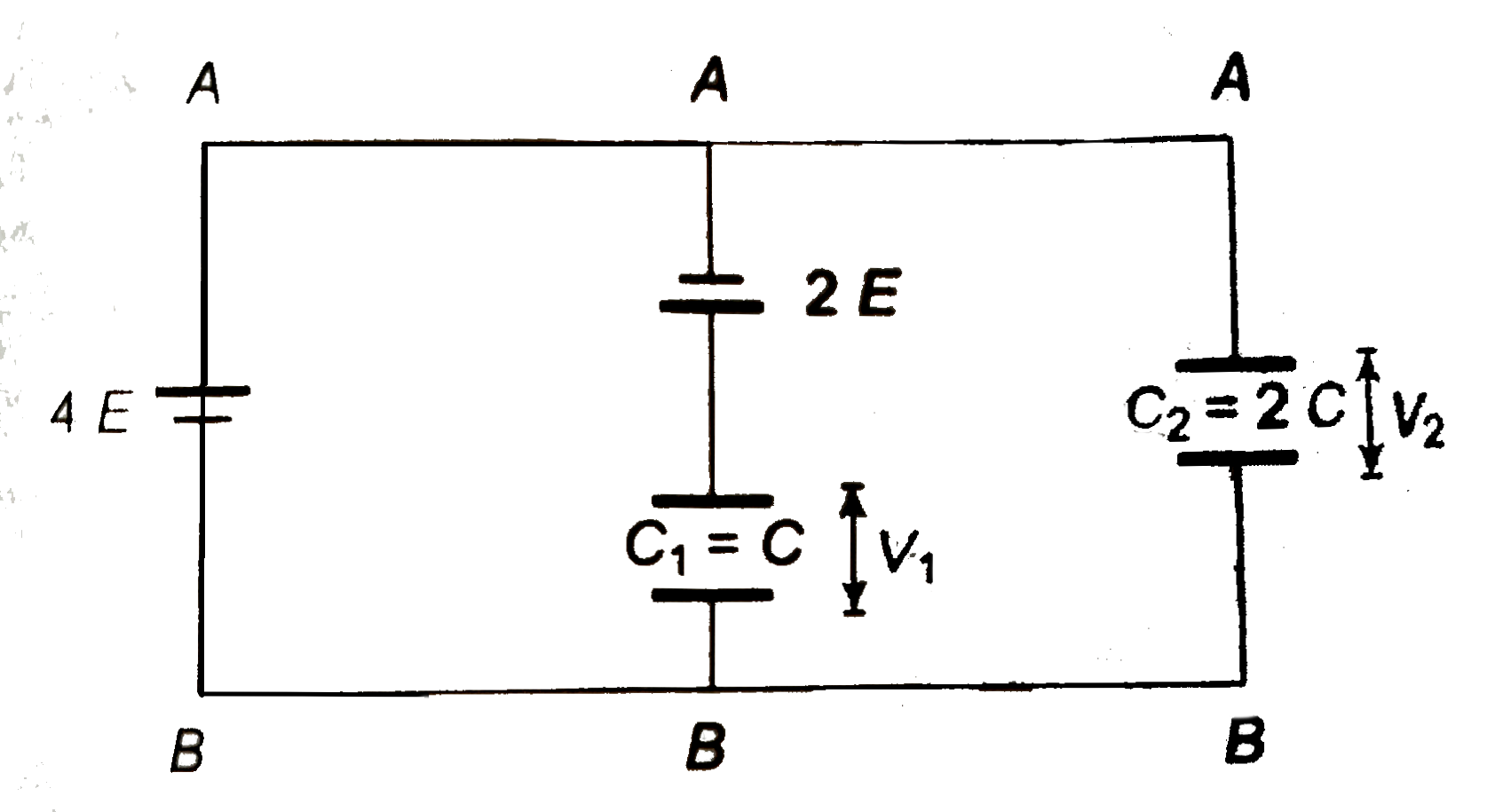
p.d. across = C2:V2=4E
p.d.acrossC1:VA+2E−V1=VB⇒VA−VB+2E=V1
4E+2E=V1⇒V1=6E
U1U2=12C1V2112C2V22=(6)22(4)2=98
Total charge on capacitors Qi=C1V1+C2V2=14CE.
Topper's Solved these Questions
- THERMAL AND ELECTRIC EFFECT oF CURRENTBook:CP SINGHChapter:THERMAL AND ELECTRIC EFFECT oF CURRENTExercise:Exercises
Explore 101 Videos
- SEMICONDUCTORSBook:CP SINGHChapter:SEMICONDUCTORSExercise:Exercises
Explore 140 Videos
- WAVE NATURE OF LIGHTBook:CP SINGHChapter:WAVE NATURE OF LIGHTExercise:EXERCISES
Explore 140 Videos
Similar Questions
(a) Find potential difference between A and B and C.

(b) Find potential difference across capacitor C.

(i) Find ratio of energy stored in capacitors, C1 and (C2)
(ii) If battery of emf of 2E is short -circuited, find charge flown in battery of emf 4E.
View SolutionWhere is energy stored in a capacitor?
View Solution
Knowledge Check
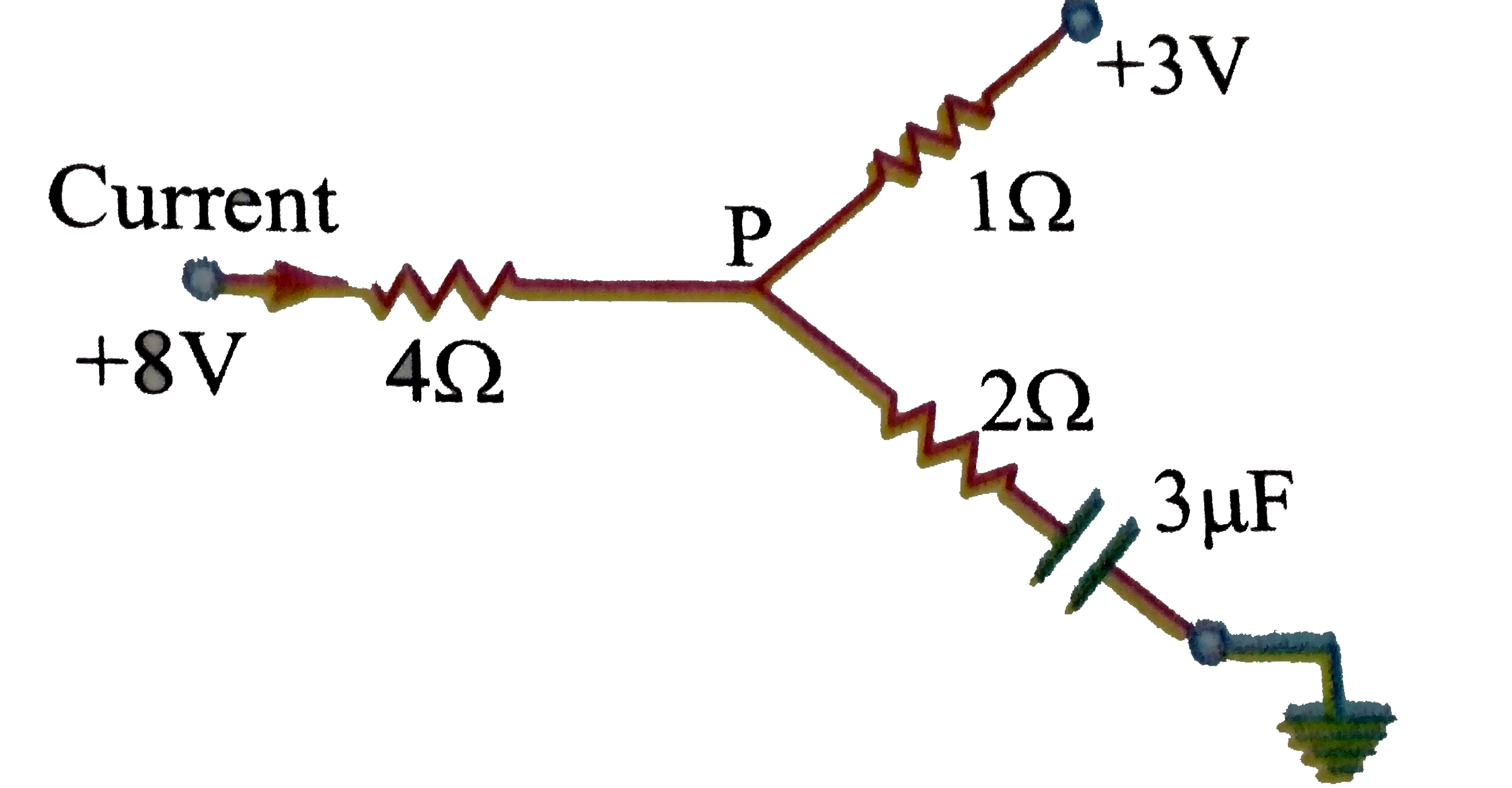
the energy stored in the capacitor isA12μJB24μJC36μJD48μJEnergy Stored in the capacitor is
Aε0E2×AdB(12)ε0E2×AdCε0E2×AdDε0E×AdAn uncharged capacitor is fully charged with a battery . The ratio of energy stored in the capacitor to the work done by the battery in this process is
A1:2B2:1C1:1D2:3
Capacitor C3 in the circuit is variable capacitor (its capacitance can be varied). Graph is plotted between potential difference V1 (across capacitor C1) versus C3.
Electric potential V1 approaches on asymptote of 10 volts as C3→∞
The ratio of energy stored in capacitorC1 to that of total energy whenC3→∞ is
View Solution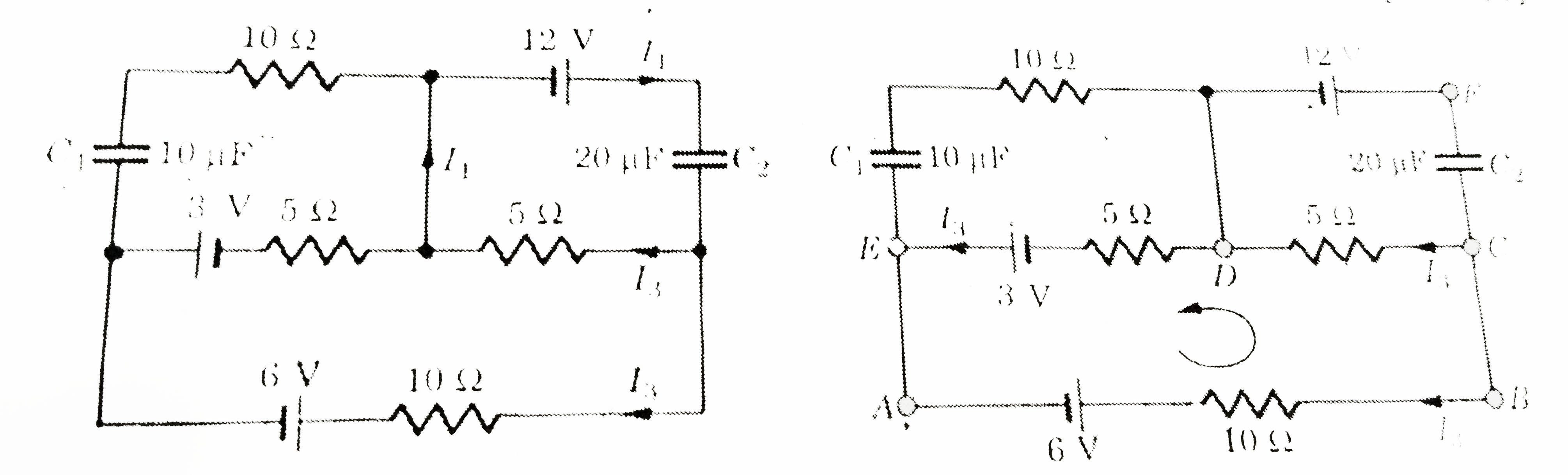
Find currents I1,I2 and I3 and the energy stored in the capacitors C1andC2 in the circuit shown in figure.
View Solution(i) Use Gauss's law to find the electric field due to a uniformly charged infinite plane sheet. What is the direction of field for positive and negative charge densities?
(ii) Find the ratio of the potential differences that must be applied across the parallel and eries combination of two capacitors C1 and C2 with their capacitances in the ratio 1:2 so that the energy stored in the two of two capacitors C1 and C2 with their capacitances in the ratio 1:2 so that the energy stored in the two cases becomes the same.
View SolutionTwo capacitors of equal capacitance (C1=C2) are as shown in the figure. Initially, while the switch is open (as shown) one of the capacitors is uncharged and the other carries charge Q0. The energy stored in the charged capacitor is U0. Sometime after the switch is closed, the capacitors C1andC2 carry charged Q1andQ2 respectively, the energy stored in the capacitors are U1andU2 respectively. Which of the following expression is correct?

View SolutionIn a series circuit C=2μF,L=1mH and R=10Ω, when the current in the circuit is maximum, at that time the ratio of the energies stored in the capacitor and the inductor will be
View Solution