An isotropic popint source emits light with wavelength 589 nm. The radiation power of the source is P = 10 W.
(a) Find the number of photons passing through unit area per second at a distance of 2 m from the source
(b) Also calculate the distance between the source and the point at which the mean concentration of the photons is $100 / c m^{3}$ .

Text Solution

Verified by Experts

(a) $P = n_{0} \frac{h c}{λ}$
$n_{0} = \frac{P λ}{h c}$ ,
where $n_{0}$ : number of photons/sec,

At distance r from point source, number of photons / area / time
$n' = \frac{n_{0}}{4 π r^{2}} = \frac{P λ}{h c .4 π r^{2}}$
$= \frac{10 \times 589 \times 10^{- 9}}{6.6 \times 10^{- 34} \times 3 \times 10^{8} \times 4 π \times {(2)}^{2}}$
$= 5.92 \times 10^{18} / m^{2}$ . sec
(b) Consider a spherical shell of radius r and thickness dr

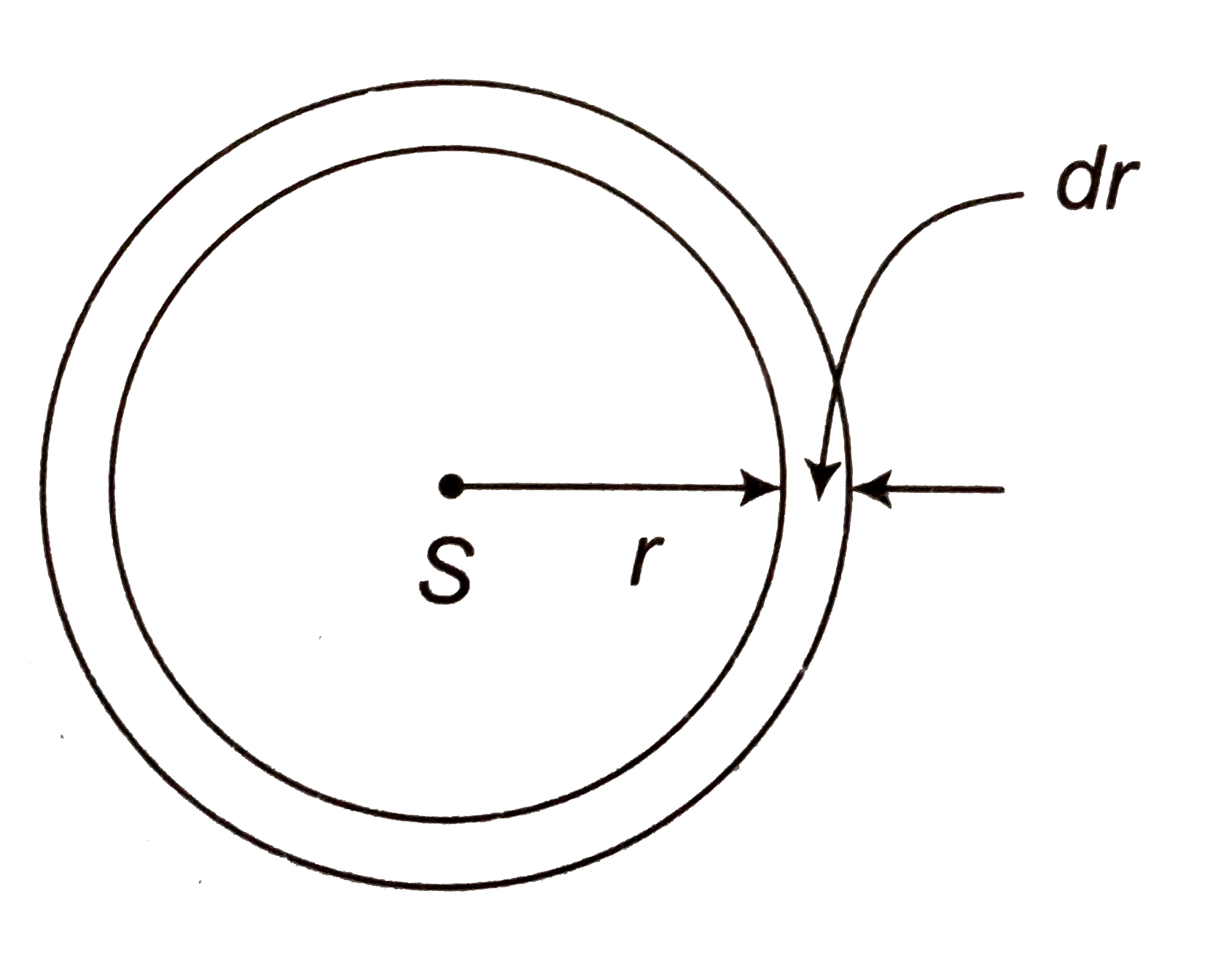
Volume of this shell $d V = 4 π r^{2} d r$
If n: number of photons/volume
Number of photons crossing this shell per unit time
$n_{0} = n (4 π r^{2} \frac{d r}{d t})$ $[w h e r e \frac{d r}{d t} = c]$
$n = \frac{n_{0}}{4 π r^{2} c} = \frac{P λ / h c}{4 π r^{2} c}$
$r = \sqrt{\frac{P λ}{4 π h c^{2} n}}$
$= \sqrt{\frac{10 \times 589 \times 10^{- 9}}{4 \times 3.14 \times 6.6 \times 10^{- 34} \times (3 \times 10^{8}) \times 100 \times 10^{6}}}$
$= 8.88 m$

Updated on:21/07/2023

|

Class 12 PHYSICS DUAL NATURE OF RADIATION AND MATTER

Topper's Solved these Questions

Similar Questions

Knowledge Check

The number of photons emitted per second by a $62 W$ source of monochromatic light of wavelength $4800 A^{\circ}$ is
A $1.5 \times 10^{19}$
B $1.5 \times 10^{20}$
C $2.5 \times 10^{20}$
D $4 \times 10^{20}$

CP SINGH-PHOTOELECTRIC EFFECT-EXERCISES

Home
Ask Doubt
Profile