The line joining the centre of a circle to the midpoint of a chord is perpendicular to the chord.
Step by step video & image solution for The line joining the centre of a circle to the midpoint of a chord is perpendicular to the chord. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
The measure of the line segment joining the centre of a circle to the mid-point of a chord is :
Atwice the measure of the chordBhalf the measure of the chordCequal to the measure of the chordDNone of the aboveThe straight line drawn from the centre of a circle perpendicular to a chord P–– the chord.
A straight line will not cut a circle in more than O–– distinct points.
R–– chords of a circle are equidistant from the centre of the circle.APQRTrisectThreePerpendicularBPQRDefinesTwoEqualCPQRBisectsTwoEqualDPQRBisectsThreeParallelIn the given figure, AC is the diameter of the circle with centre O. Chord BD is perpendicular to AC. Express p in terms of x.
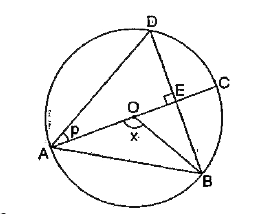 Ax/2B90∘+x/2C90∘−x/2D180∘−x
Ax/2B90∘+x/2C90∘−x/2D180∘−x
Theorem :-2 The perpendicular from centre of a circle to the chord bisects the chord and Perpendicular bisectors of two chords of a circle intersects at the centre.
View SolutionThe perpendicular from the centre of a circle to a chord bisects the chord.
View SolutionThe perpendicular from the centre of a circle to a chord bisects the chord.
View SolutionIf two equal chords of a circle in intersect within the circle, prove that : the segments of the chord are equal to the corresponding segments of the other chord. the line joining the point of intersection to the centre makes equal angles with the chords.
View SolutionIf two equal chords of a circle in intersect within the circle, prove that: the segments of the chord are equal to the corresponding segments of the other chord. the line joining the point of intersection to the centre makes equal angles with the chords.
View Solution
