From the top of the hill; the angle of depressions of two consecutive kilometre stones due east are found to be and . Find the height of the hill.
To solve the problem, we will use the properties of angles of depression and some trigonometric ratios.
1. Understanding the Problem:
- We have a hill from which the angles of depression to two consecutive kilometer stones are given as 30∘ and 45∘.
- Let the height of the hill be h.
- Let the distance from the base of the hill to the first kilometer stone (the one at 45∘ angle) be x kilometers. Therefore, the distance to the second kilometer stone (the one at 30∘ angle) will be x+1 kilometers.
2. Setting Up the Right Triangles:
- From the top of the hill to the first stone (at 45∘):
tan(45∘)=hx
Since tan(45∘)=1, we have:
h=x(1)
- From the top of the hill to the second stone (at 30∘):
tan(30∘)=hx+1
Since tan(30∘)=1√3, we have:
1√3=hx+1
Rearranging gives:
h=x+1√3(2)
3. Equating the Two Expressions for Height:
- From equations (1) and (2):
x=x+1√3
4. Solving for x:
- Multiply both sides by √3:
x√3=x+1
- Rearranging gives:
x√3−x=1
x(√3−1)=1
x=1√3−1
5. Rationalizing the Denominator:
- Multiply the numerator and denominator by √3+1:
x=1(√3+1)(√3−1)(√3+1)=√3+13−1=√3+12
6. Finding the Height h:
- Substitute x back into equation (1):
h=x=√3+12
Final Answer:
The height of the hill is:
h=√3+12 kilometers
- We have a hill from which the angles of depression to two consecutive kilometer stones are given as 30∘ and 45∘.
- Let the height of the hill be h.
- Let the distance from the base of the hill to the first kilometer stone (the one at 45∘ angle) be x kilometers. Therefore, the distance to the second kilometer stone (the one at 30∘ angle) will be x+1 kilometers.
- From the top of the hill to the first stone (at 45∘):
tan(45∘)=hx
Since tan(45∘)=1, we have:
h=x(1)
tan(30∘)=hx+1
Since tan(30∘)=1√3, we have:
1√3=hx+1
Rearranging gives:
h=x+1√3(2)
- From equations (1) and (2):
x=x+1√3
- Multiply both sides by √3:
x√3=x+1
- Rearranging gives:
x√3−x=1
x(√3−1)=1
x=1√3−1
- Multiply the numerator and denominator by √3+1:
x=1(√3+1)(√3−1)(√3+1)=√3+13−1=√3+12
- Substitute x back into equation (1):
h=x=√3+12
The height of the hill is:
h=√3+12 kilometers
Similar Questions
From the top of a hill, the angles of depression of two consecutive kilometre stones due east are found to be 300and450 . Find the height of the hill.
View SolutionFrom the top of a building 60m high the angles of depression of the top and the bottom of a tower are observed to be 300and600 . Find the height of the tower.
View Solution
Knowledge Check
From the top of a cliff 50 m high, the angles of depression of the top and bottom of a tower are observed to be 30∘ and 45∘. The height of tower is
A50mB50√3mC50(√3−1)mD50(1−√33)mFrom the top of a cliff 50m high, the angles of depression of the top and bottom of a tower are observed to be 30∘ and 45∘ The height of tower is
A50 mB50√3C50(√3−1)D50(1−√33)mFrom the top of a cliff 50m high, the angles of depression of the top and bottom of a tower are observed to be 30∘ and 45∘ The height of tower is
A50 mB50√3C50(√3−1)D50(1−√33)m
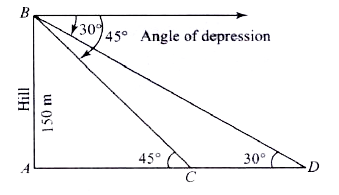
The height of a hill is 150 m. From the top of the hill the angles of depression of two objects lying towards east to the hill are 45∘ and 30∘. Find the distance btween the objects.
View SolutionFrom the top of a cliff, 200m high, the angle of depression of the top and bottom of a tower are observed to be 30∘ and 60∘, find the height of the tower.
View SolutionFrom the top of a building 60m high the angles of depression of the top and the bottom of a tower are observed to be 30o and 60o . Find the height of the tower.
View SolutionFrom an aeroplane vertically over a straight horizontal road, the angles of depression of two consecutive kilometre-stones on the opposite sides of the aeroplane are observed to be αandβ. The height of the aeroplane above the road is
View SolutionFrom an aeroplane just over a straight road, the angles of depression of two consecutive kilometre is stones situated at opposite sides of the aeroplane were found to be 60∘ and 30∘ respectively. The height (in km) of the aerophlane from the road at that instant was
(Given √3=1.732)
View Solution
