From the foot of a tower 80m high, a stone is thrown up so as to reach the top of a tower. After 2s, another stone is dropped from the top of the tower. When and where the two stones meet?
First, we have to calculate the intial velocity of the projected stone. When it reaches the top of the tower, i.e. at height 80m, its velocity becomes zero.
0=u2−2g×80⇒u=40m/s
let the stones meet t seconds after projection of the first. The dropped stone or second stone will take (t−2) seconds. Let the stones meet at height h.
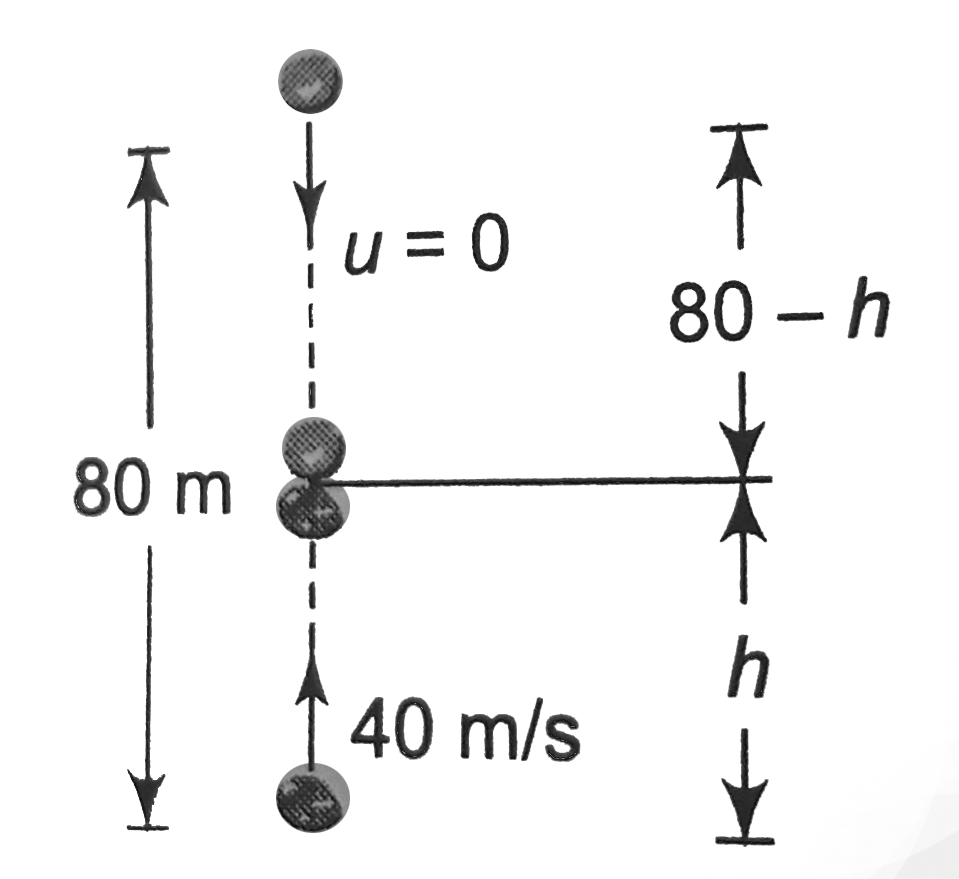
First stone: h=40t−12gt2...(i)
Second stone: 80−h=12g(t−2)2...(ii)
Adding (i) and (ii), we get
80=40t−12g[t2−(t−2)2]
=40t−5(2t−2)(2)=40t−20t+20
20t=60⇒t=3s
h=40t−12gt2=40×3−5×(3)2
=120−45=75m
The stones meet at height 75m and after 3s from the projection of the first stone.
Note: Till now we have taken those problem in which the partical is moving in one direction (either upward or downward). For these problems, we have assumed vertically downward//upward direction positive according to our convenience.
In some of the following problems, the particle is moving in both the directions, upward direction followed by downward direction. For these type of problems, we will assume vertically upward direction positive, displacement above origin positive and below origin negative.
Topper's Solved these Questions
Similar Questions
From the foot of a tower 90m high, a stone is thrown up so as to reach the top of the tower. Two second later another stone is dropped from the top of the tower. Find when and where two stones meet (Take g=10m/s2)
View SolutionFrom the foot of a tower 90 cm. high, a stone is thrown up so as to reach the top of the tower. Two seconds later another stone is dropped from the top of the tower. The two stones meet at a height...... after time..... of the start of 1st stone.
View Solution
Knowledge Check
- Question 1 - Select One
A stone dropped from the top of a tower reaches the ground in 3 s. The height of the tower is
A18.6 mB39.2 mC44.1 mD98 m - Question 2 - Select One
A stone is dropped from the top of a tower and travels 24.5 m in the last second of its journey. The height of the tower is
A44.1 mB49 mC78.4 mD72 m - Question 3 - Select One
A stone from the top of a tower, travels 35 m in the last second of its journey. The height of the tower is
A20mB40mC60mD80m
A stone is allowed to fall from the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25m/s. Calculate when and where the two stone will meet.
View SolutionA stone is allowed to fall from the top of a tower 100m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25ms.Calculate when and where the two stones will meet.
View Solution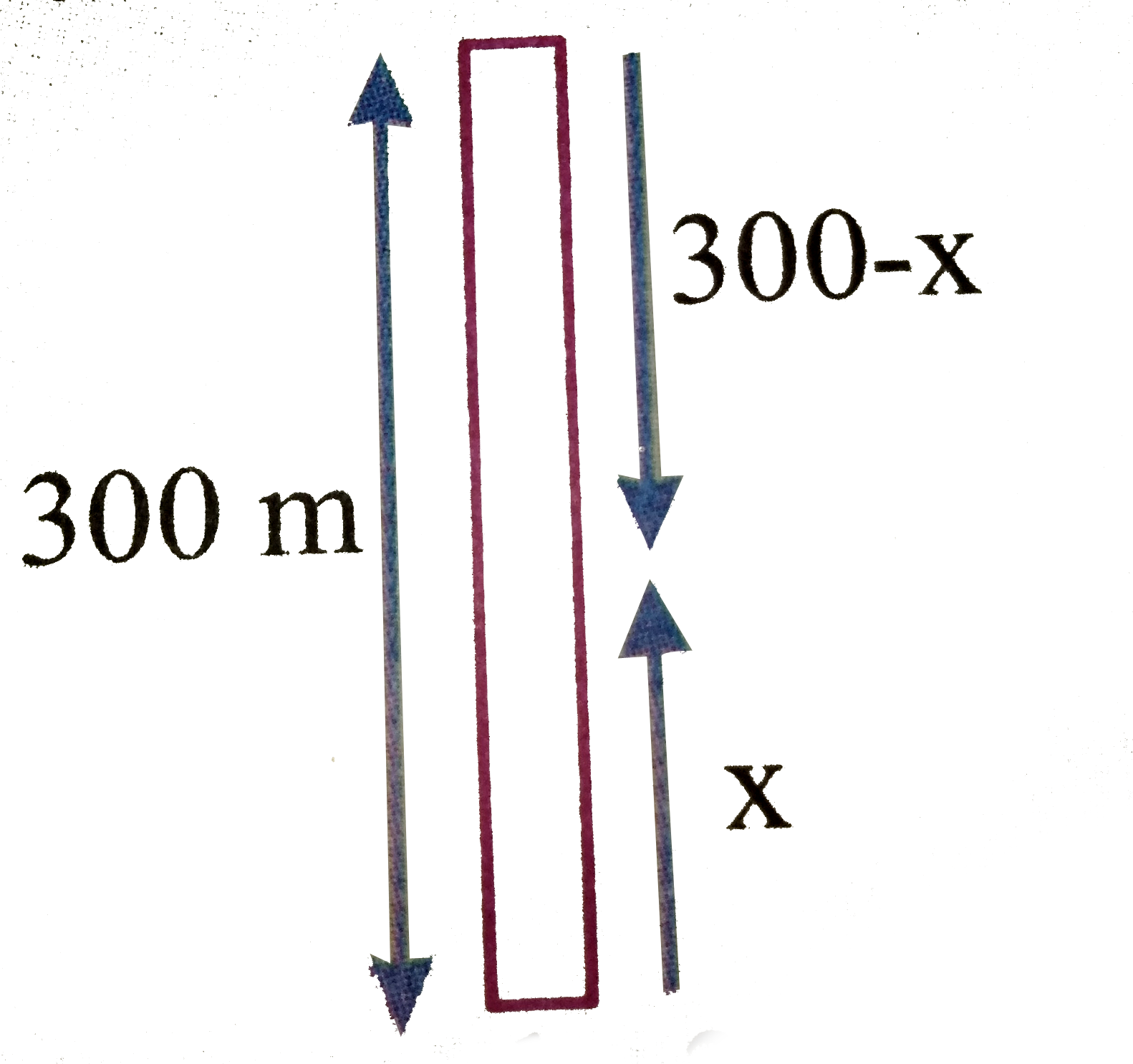
A stone is allowed to fall from the top of a tower 300 m height and at the same time another stone is projected vertically up from the ground with a velocity 100m/s. Find when and where the two stones meet?View SolutionFrom top of a tower of hight 80m, person drops a stone. And after one second another stone is thrown down from the top of the same tower with some initial velocity, such that both the stones reach the ground at the same instant. Calculate the magnitude of the initial velociy (in m/s) of the second stone. (Take g=10m/s2)
View SolutionFrom the top of the house 18m high, if the angle of elevation of the top of a tower is 45° and the angle of depression of the foot of the tower is 30°, then the height of the tower is
View Solution

