From the top of a toward of height $60 m$ , a ball is thrown vertically upward with speed $20 m / s$ . After some time, the ball strikes the ground. Sketch the v-t and s-t graph.

Video Solution

Text Solution

Verified by Experts

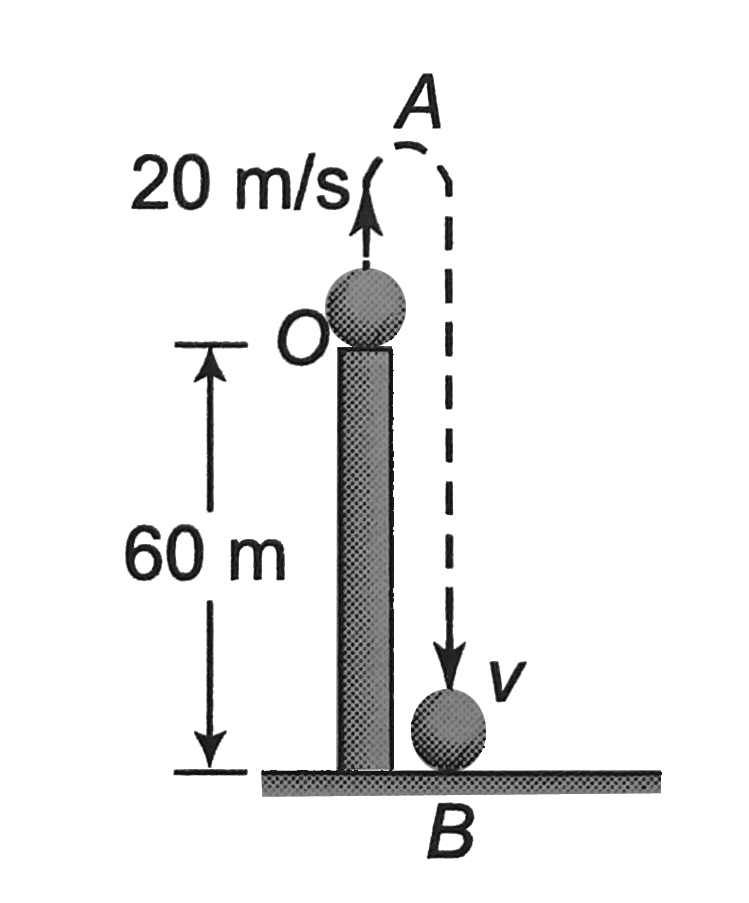
Assume $O$ as the origin and upward direction +ve.
$O$ to B throught $A$ :
Displacement $s = - 60 m$
$s = u t - \frac{1}{2} g t^{2}$
$- 60 = 20 t - 5 t^{2}$
$t^{2} - 4 t - 12 = 0$
$(t - 6) (t + 2) = 0$
$t = 6 s$
The ball will strike the ground after time $t = 6 s$ .
v-t graph: $v = u - g t$
$v = 20 - 10 t$
$t = 0, v = 20 m / s$
$t = 6 s, v = - 40 m / s$
$v = 0 \Rightarrow 20 - 10 t \Rightarrow t = 2 s$
Shape: $y = 20 - 10 x$ (straight line)
$y = c + m x$
$c = 20$ ,+ve
$m = - 10$ , -ve

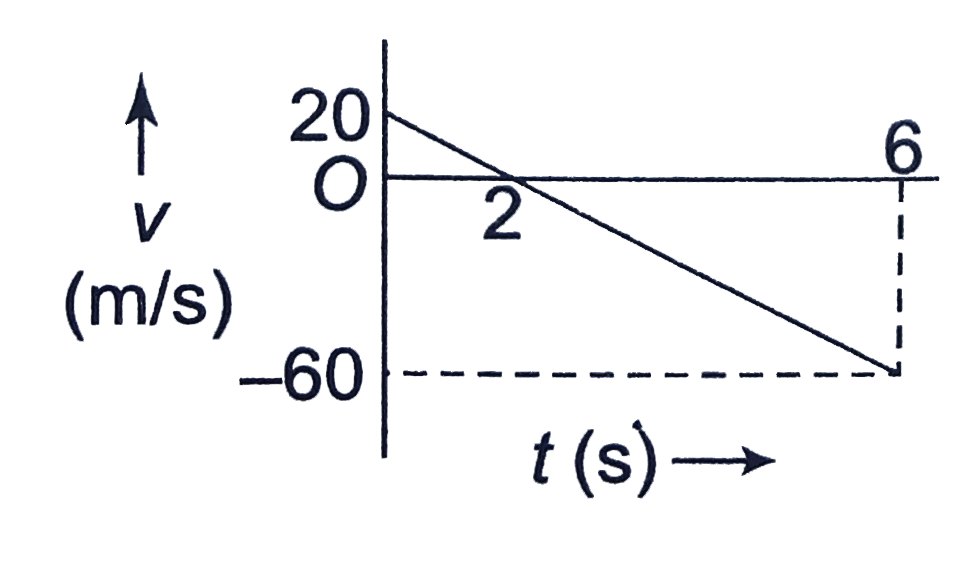
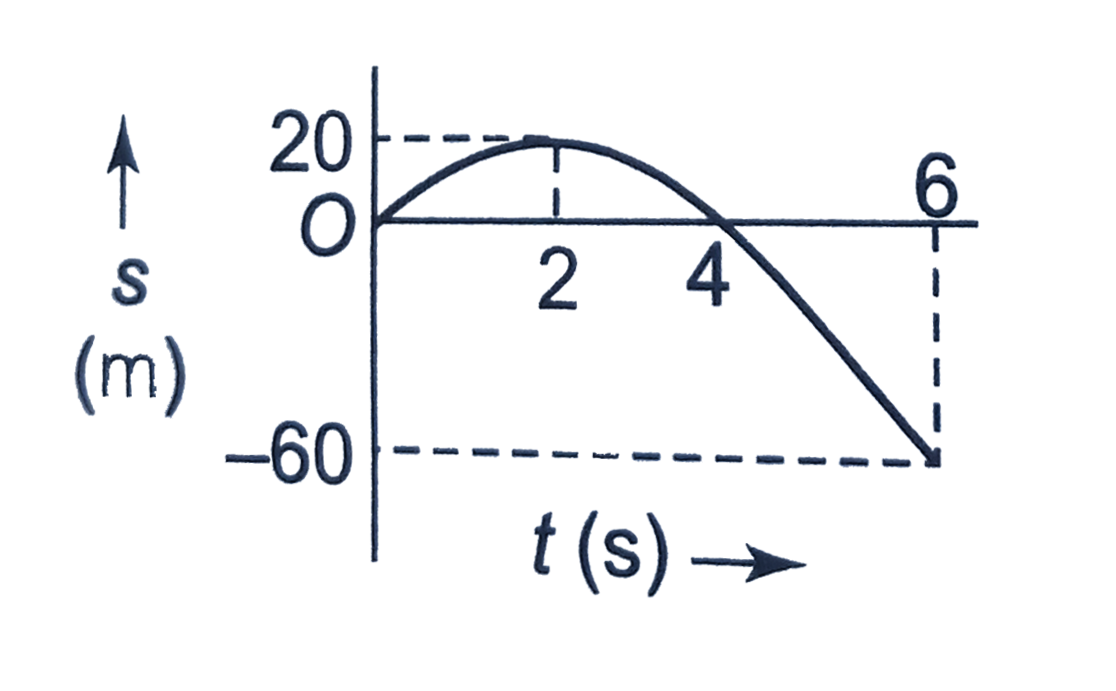
s-t graph: $s = u t - \frac{1}{2} g t^{2} = 20 t - 5 t^{2}$
$t = 0, s = 0$
$t = 6 s, s = - 60 m$
$s = 0 \Rightarrow 20 t - 5 t^{2} = 0 \Rightarrow t (20 - 5 t) \Rightarrow t = 0, t = 4 s$
s will be maximum, when $v (= d s / d t) = 0 \Rightarrow t = 2 s$
At $t = 2 s, s = 20 m$
A part from the initial and final values, we required values at critical points, where change takes place.
Shape: $y = 20 x - 5 x^{2}$ (parabola)
The coefficient of $x^{2}$ is -ve, i.e. the parabola will be open downward.

Updated on:21/07/2023