Concyclic points on ellipse
Step by step video & image solution for Concyclic points on ellipse by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
Q is a point on the auxiliary circle of an ellipse. P is the corresponding point on ellipse. N is the foot of perpendicular from focus S, to the tangent of auxiliary circle at Q. Then
ASP=SNBSP=PQCPN=SPDNQ=SPIf the coordinates of four concyclic point on the rectangular hyperbola xy =c2 are i=1,2,3,4 then
At1t2t3t4=−1Bt1t2t3t4=1Ct1t3=t2t4Dt1t2t3t4=c2
Prove that product of parameters of four concyclic points on the hyperbola xy=c2 is 1. Also, prove that the mean of these four concyclic points bisects the distance between the centres of the hyperbola and the circle.
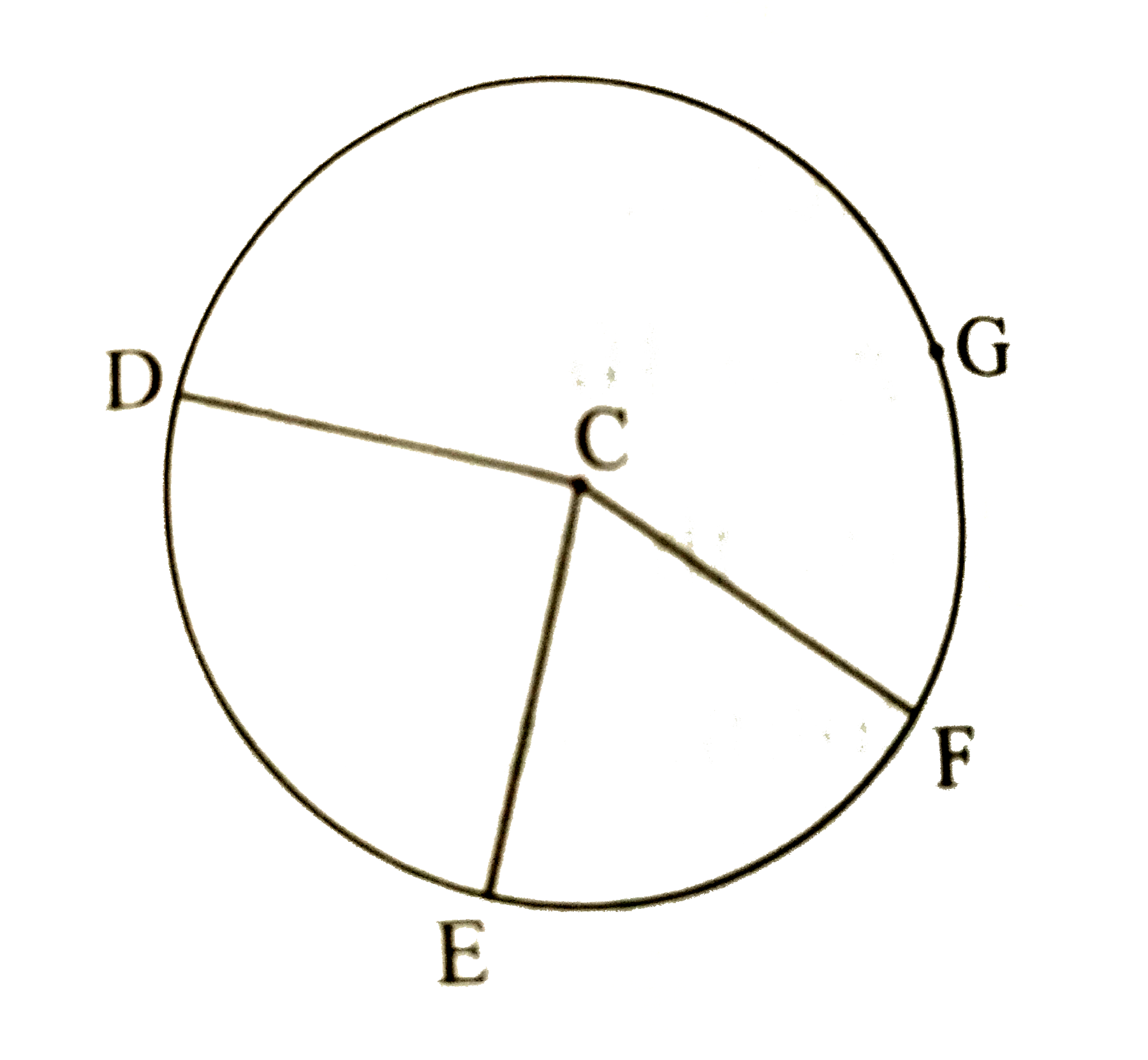
View SolutionIn the figure , points G,D,E,F are concyclic points of a cicle with centreC.
∠ECF=70∘
m ( arc DGF ) =200∘,
find m(arc DE) and m(arc DEF ) .
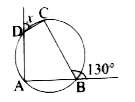
View SolutionIn the adjoining figure A, B, C, D are the concyclic points. The value of 'x' is :
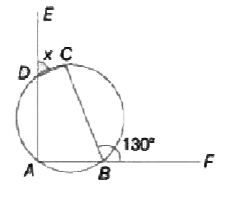
View SolutionIn the following figure,A, B, Cand D are the concyclic points. Find the value of x.
View SolutionDerive an equation to find distance of a point on ellipse to the focus of ellipse.
View SolutionNumber of points on the ellipse x2a2+y2b2=1 at which the normal to the ellipse passes through at least one of the foci of the ellipse is
View Solution
