A body is moved from rest along a straight line by a machine delivering constant power. The ratio of displacement and velocity (s/v) varies with time t as
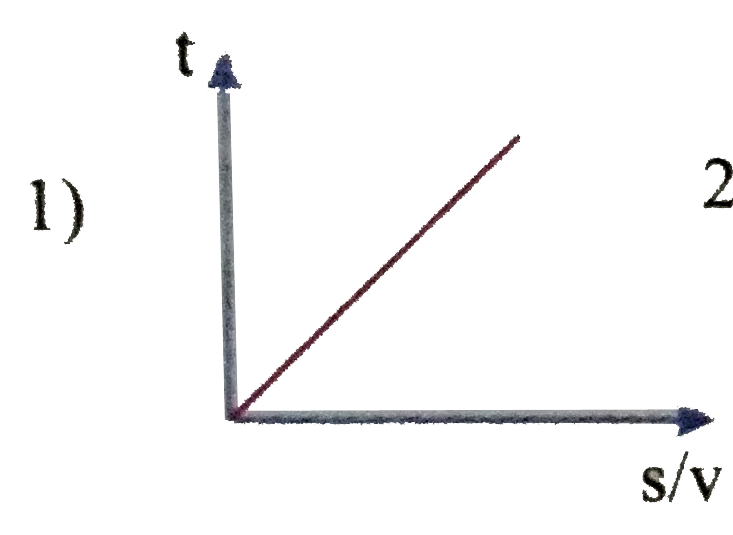

The correct Answer is:A
To solve the problem step by step, we need to analyze the relationship between displacement (s), velocity (v), and time (t) when a body is moved from rest by a machine delivering constant power.
Step 1: Understand the relationship between power, work, and time
Given that the power (P) is constant, we can express the work done (W) as:
W=P⋅t
where t is the time.
Step 2: Relate work done to kinetic energy
The work done on the body is equal to the change in kinetic energy (KE). Since the body starts from rest, the kinetic energy can be expressed as:
KE=12mv2
Thus, we have:
P⋅t=12mv2
Step 3: Solve for velocity (v)
Rearranging the equation gives us:
v2=2Ptm
Taking the square root, we find:
v=√2Ptm
Step 4: Relate displacement (s) and velocity (v)
Since velocity is defined as the rate of change of displacement with respect to time, we can write:
v=dsdt
Substituting our expression for v gives:
dsdt=√2Ptm
Step 5: Separate variables and integrate
We can rearrange this to separate variables:
ds=√2Pm⋅t1/2dt
Now we will integrate both sides. The left side integrates from 0 to s and the right side from 0 to t:
∫ds=√2Pm∫t1/2dt
Step 6: Perform the integration
The integral of t1/2 is:
∫t1/2dt=23t3/2
Thus, we have:
s=√2Pm⋅23t3/2
Step 7: Find the ratio sv
Now, we need to find the ratio sv:
Using our expressions for s and v:
sv=s√2Ptm
Substituting for s:
sv=√2Pm⋅23t3/2√2Ptm
Step 8: Simplify the ratio
This simplifies to:
sv=23t
This shows that the ratio of displacement to velocity varies linearly with time.
Conclusion
Thus, the final answer is:
sv=23t
Given that the power (P) is constant, we can express the work done (W) as:
W=P⋅t
where t is the time.
The work done on the body is equal to the change in kinetic energy (KE). Since the body starts from rest, the kinetic energy can be expressed as:
KE=12mv2
Thus, we have:
P⋅t=12mv2
Rearranging the equation gives us:
v2=2Ptm
Taking the square root, we find:
v=√2Ptm
Since velocity is defined as the rate of change of displacement with respect to time, we can write:
v=dsdt
Substituting our expression for v gives:
dsdt=√2Ptm
We can rearrange this to separate variables:
ds=√2Pm⋅t1/2dt
Now we will integrate both sides. The left side integrates from 0 to s and the right side from 0 to t:
∫ds=√2Pm∫t1/2dt
The integral of t1/2 is:
∫t1/2dt=23t3/2
Thus, we have:
s=√2Pm⋅23t3/2
Now, we need to find the ratio sv:
Using our expressions for s and v:
sv=s√2Ptm
Substituting for s:
sv=√2Pm⋅23t3/2√2Ptm
This simplifies to:
sv=23t
This shows that the ratio of displacement to velocity varies linearly with time.
Thus, the final answer is:
sv=23t
Topper's Solved these Questions
- WORK POWER AND ENERGYBOOK - NARAYNACHAPTER - WORK POWER AND ENERGYEXERCISE - NCERT (Based Questions)
45 Videos
- WORK POWER AND ENERGYBOOK - NARAYNACHAPTER - WORK POWER AND ENERGYEXERCISE - Level-V (Single Answer)
24 Videos
- WORK POWER AND ENERGYBOOK - NARAYNACHAPTER - WORK POWER AND ENERGYEXERCISE - Level- II (C.W)
68 Videos
- WORK , ENERGY & POWER BOOK - NARAYNACHAPTER - WORK , ENERGY & POWER EXERCISE - EXERCISE IV
43 Videos
Similar Questions
A body is moved from rest along straight line by a machine delievering constant power.Time taken by body to travel a distance 'S' is proportional to
View SolutionA body is moved from rest along a straight line by a machine delivering constant power .Calculate the velo0city and distance moved by the body as a function of time .
View Solution
Knowledge Check
A body is being moved from rest along a straight line by a machine delivering constant power. The distance covered by body in time t is proportional to
A√tBt3/2Ct3/4Dt2A body is moved along a straight line by a machine delivering constant power . The distance moved by the body in time t is proportional to
At1/2Bt3/4Ct3/2Dt2A body is moved along a straight line by a machine delivering a constant power. The distance moved by the body in time t is proportional to
At3/4Bt1/2Ct1/4Dt1/2
A body is moved along a straight line by a machine delivering a power proportional to time (P∝t). Then, match the following.
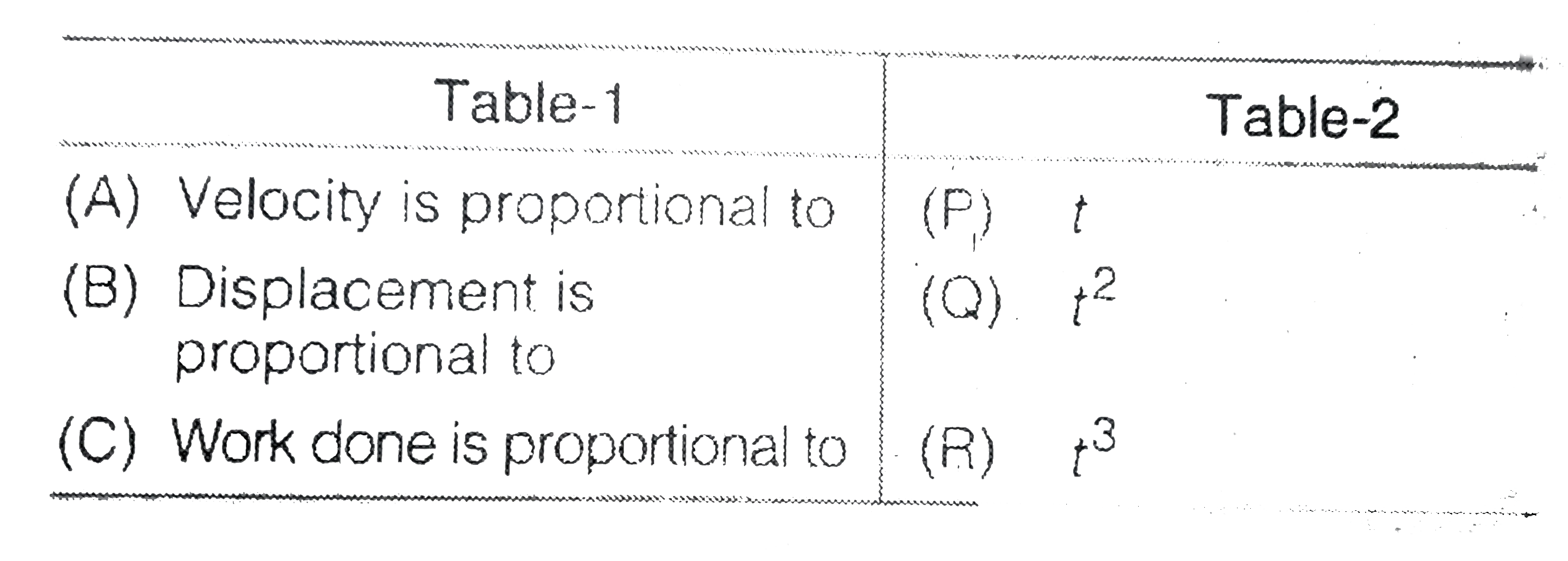
View SolutionA body at rest is moved along a horizontal straight line by a machine delivering a constant power. The distance moved by the body in time ‘t’ is proportional to:
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moves along a straight line by a machine dellvering a constant power . The distance moved by the body in time t is propertional as
View SolutionA body is moved along a straight line by a machine delivering a power proportional to time (p∝t) Then, match the following.
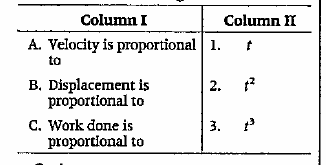
View Solution
