A man squatting on the ground gets straight up and stand. The force of reaction of ground on the man during the process is.
constant and equal to mg in magnitude
constant and greater than mg in magnitude
variable but always greater than mg.
at first greater than mg, and later becomes equal to mg.
The correct Answer is:D
To solve the problem of the force of reaction of the ground on a man who is squatting and then stands up, we can analyze the situation step by step.
Step 1: Understand the Initial Position
When the man is squatting, he is exerting a force on the ground equal to his weight (mg, where m is mass and g is acceleration due to gravity). The ground exerts an equal and opposite force (normal reaction, N) back on him.
Hint: Remember Newton's Third Law: for every action, there is an equal and opposite reaction.
Step 2: Analyze the Transition to Standing
As the man begins to stand up, he needs to exert a force greater than his weight to accelerate upwards. This means that the normal reaction force (N) from the ground must be greater than his weight (mg) during the initial phase of standing.
Hint: Consider the forces acting on the man when he is accelerating upwards.
Step 3: Apply Newton's Second Law
Using Newton's Second Law, we can express the forces when the man is transitioning from squatting to standing:
- The net force acting on the man is given by N−mg=ma, where a is the upward acceleration.
- Rearranging gives us N=mg+ma. Here, N must be greater than mg because a is positive (the man is accelerating upwards).
Hint: Identify that the net force must be greater than zero for the man to accelerate upwards.
Step 4: Analyze the Final Position
Once the man stands up completely, he is in a state of rest again. At this point, the forces acting on him are balanced:
- The normal force (N) from the ground is equal to his weight (mg), so N=mg.
Hint: When at rest, the forces acting on an object are balanced.
Conclusion
In summary, during the process of standing up, the force of reaction (normal force) from the ground on the man is greater than his weight while he is accelerating upwards and equals his weight when he is standing still. Therefore, we can conclude:
- While squatting and beginning to stand: N>mg
- While standing still: N=mg
Thus, the correct option is that the force of reaction of the ground on the man during the process is greater than his weight when he is standing up.
Final Answer: The force of reaction of the ground on the man during the process is greater than his weight (N > mg) when he is standing up.
When the man is squatting, he is exerting a force on the ground equal to his weight (mg, where m is mass and g is acceleration due to gravity). The ground exerts an equal and opposite force (normal reaction, N) back on him.
As the man begins to stand up, he needs to exert a force greater than his weight to accelerate upwards. This means that the normal reaction force (N) from the ground must be greater than his weight (mg) during the initial phase of standing.
Using Newton's Second Law, we can express the forces when the man is transitioning from squatting to standing:
- The net force acting on the man is given by N−mg=ma, where a is the upward acceleration.
- Rearranging gives us N=mg+ma. Here, N must be greater than mg because a is positive (the man is accelerating upwards).
Once the man stands up completely, he is in a state of rest again. At this point, the forces acting on him are balanced:
- The normal force (N) from the ground is equal to his weight (mg), so N=mg.
In summary, during the process of standing up, the force of reaction (normal force) from the ground on the man is greater than his weight while he is accelerating upwards and equals his weight when he is standing still. Therefore, we can conclude:
- While standing still: N=mg
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - WORK POWER AND ENERGYEXERCISE - Level-V (Single Answer)24videos
WORK POWER AND ENERGY
- BOOK - NARAYNACHAPTER - WORK POWER AND ENERGYEXERCISE - Level-V (Multiple Answer)13videos
WORK POWER AND ENERGY
- BOOK - NARAYNACHAPTER - WORK POWER AND ENERGYEXERCISE - Level- III (C.W)50videos
WORK POWER AND ENERGY
- BOOK - NARAYNACHAPTER - WORK , ENERGY & POWER EXERCISE - EXERCISE IV43videos
WORK , ENERGY & POWER
Similar Questions
Knowledge Check
- Question 1 - Select One
A man squatting on the ground gets straight up and stand. The force of reaction of ground on the man during the process is.
Aconstant and equal to mg in magnitudeBconstant and greater than mg in magnitude.Cvariable but always greater then mgDat first greater than mg and later becomes equal to mg - Question 2 - Select One
A man is running on the ground .It is known that the coefficient of friction between the man and the ground is μ . Then which of the following statements is correct
ANormal reaction between man and ground is equal to weight of manBThe direction of friction on man is in the direction of normal reaction on the manCDirection of friction on man is opposite to the direction of motion of manDMaximum acceleration of man can be 2μg - Question 3 - Select One
A boy of mass 50 kg standing on ground exerts a force of 500 N on the ground. The force exerted by the ground on the boy will be :
A100 NB200 NC50000 ND500 N
The pressure exerted on the ground by a man is greatest
View SolutionA main stands on the ground at a fixed distance from a siren which emits sound of fixed amplitude. The man hears the sound to be on a clear night than on a clear day.
View SolutionName the state of equilibrium in the following:
(i) A man lying on the ground
(ii) A man standing on one leg
(iii) A ball on the ground
(iv) A cone resting on its base
(v) A cone resting on its apexView SolutionA man of mass m is standing on a plank kept on ground having mass m. There is no friction between the plank and the ground. If the man walks with an acceleration a w.r.t. the plank when calculate the acceleration of the plank w.r.t. the ground. Also calculate frictional force exerted by the plank on man.
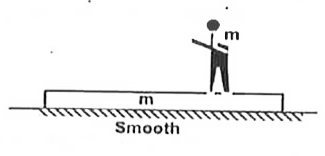 View Solution
View SolutionA man of mass 80kg pushes box of mass 20kg horizontaly. The man moves the box with a constant acceleration of 2m/s2 but his foot does not slip on the ground. There is no friction between the box and the ground. There is no friction between the box and the ground. whereas there is sufficient friction between the man's foot and the ground to prevent him from slipping.
Assertion:- The force applied by the man on the box is equal and opposite to the force applied by the force applid by the box on the man.
Reason:- Friction force applied by the ground on the man is 200N.View Solution

