A body projected from a point O at an angle θ, just crosses a wall y m high at a distance. x m from the point of projection and strikes the ground at Q beyond the wall as shown, then find height of the wall
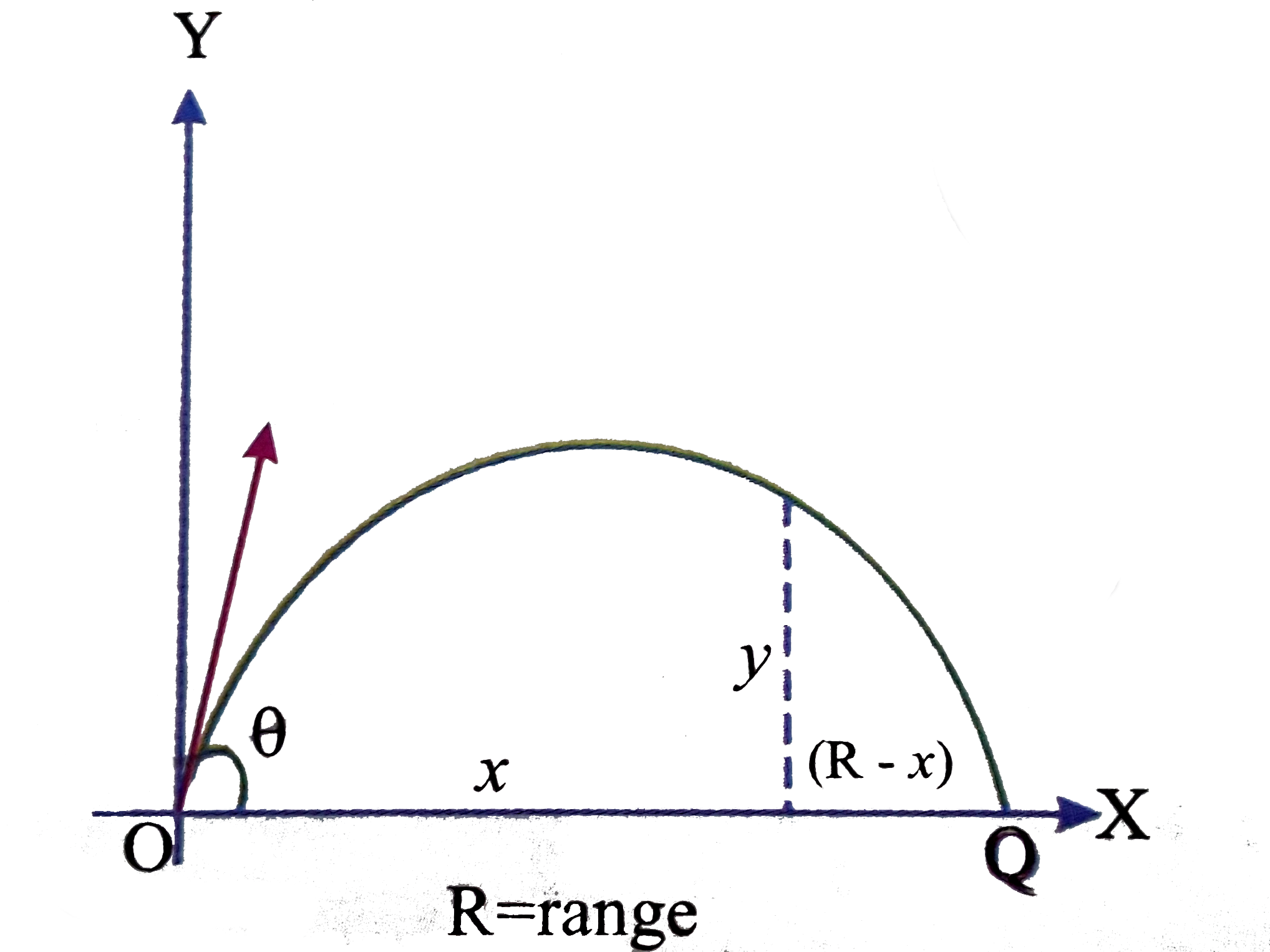
We know that the equation of the trajectory is y=xtanθ−gx22u2cos2θcan be written as
y=xtanθ−(gx22u2cos2θ)sinθsinθ
y=xtanθ−gx2tanθu2(2sinθcosθ)⇒y=xtanθ−x2tanθu2sin2θg
⇒=xtanθ[1−(xR)][∵R=u2sin2θg]
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - C.U.Q
36 Videos
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-I(C.W)
43 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LEVEL-II (H.W)
24 Videos
- MOTION IN A STRAIGHT LINEBOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 2 H.W
29 Videos
Similar Questions
A body projected just crosses a wall of 4m height,at 6m distance from the point of projection and it hits the ground at 12m away from the wall. The angle of projection is nearly
View SolutionA ball projected from ground at an angle of 45∘ just clears a wall infront. If point of projection is 4m from the foot of wall and ball strikes the ground at a distance of 6m on the other side of the wall, the height of the wall is
View Solution
Knowledge Check
A ball projected from ground at an angle of 45∘ just clears a wall infront. If point of projection is 4m from the foot of wall and ball strikes the ground at a distance of 6m on the other side of the wall, the height of the wall is
A4.4mB2.4mC3.6mD1.6mA ball is projected at an angle 45∘ with horizontal. It passes through a wall of height h at horizontal distance d1 from the point of projection and strikes the ground at a horizontal distance (d1+d2) from the point of projection, then
Ah=2dd_(2)d+d2Bh=d1d2d1+d2Ch=√2d1d2d1+d2Dh=d1d22(d1+d2)A ball is projected from the ground with speed u at an angle α with horizontal. It collides with a wall at some distance from the point of projection and returns on the same level. Coefficient of restitution between the ball and the wall is e, then time taken in path AB and maximum height attained by the ball is
 Aaeucosα,u2sin2α2gBaucosα,u2sin2α2gCaeucosα,u2sin2α2gDaucosα,u2sin2α2g
Aaeucosα,u2sin2α2gBaucosα,u2sin2α2gCaeucosα,u2sin2α2gDaucosα,u2sin2α2g
A ball projected from the ground with speed 10m/s at an angle of 45∘ with horizontal. It collides with a wall at a distance 2 m from the point of projection and returns to its original position. If the coefficient of restitution between the ball and wall is 1/x, find x.
View SolutionA ball is projected from ground with a speed of 20ms−1 at an angle of 45∘ with horizontal.There is a wall of 25m height at a distance of 10m from the projection point. The ball will hit the wall at a height of
View SolutionA ball is projected from the ground with speed u at an angle α with horizontal. It collides with a wall at a distance a from the point of projection and returns to its original position. Find the coefficient of restitution between the ball and the wall.
View SolutionA small ball is projected with a velocity u at some angle with horizontal towards a smooth vertical wall at a distance d from the point of projection after rebounding from the wall . If e is the coefficient of restitution then find maximum distance d for which ball may return to point of projection .
View SolutionA particle is projected with a velocity u from a point at ground level. Show that it cannot clear a wall of height h at a distance x from the point of projection if u2<g(h+√h2+x2).
View Solution
