When we analyse the projectile motion from any accelerated frame $O$ as ${\to r}_{o}, {\to u}_{o}$ and ${\to a}_{o}$ respectively, express the following terms, ${\to r}_{p O} + {\to a}_{p} - {\to r}_{O}, {\to u}_{p O} = {\to u}_{p} - {\to u}_{O}$ and ${\to a}_{p O} = {\to a}_{p} - {\to a}_{O}$
where $P$ stands for projectile. Then using the following kinematical equations of the projectile (For constant acceleration) relative to the accelerating frame ,we have ${\to S}_{p O} = {\to u}_{p O} t + \frac{1}{2} {\to a}_{p O} t^{2}, {\to v}_{p O}$
$= {\to u}_{p O} + {\to a}_{p O} t$ and $v_{p O}^{2} = u_{p O}^{2} + 2 \to a . {\to s}_{p} O$
Using the above expressions, anwer the following
question:A projectile has initial velocity $v_{0}$ realtive to the large plate which is moving with a constant upward acceleration a.
Refering to Q.1, velocity of the projectile relative to $B$ ofter some time

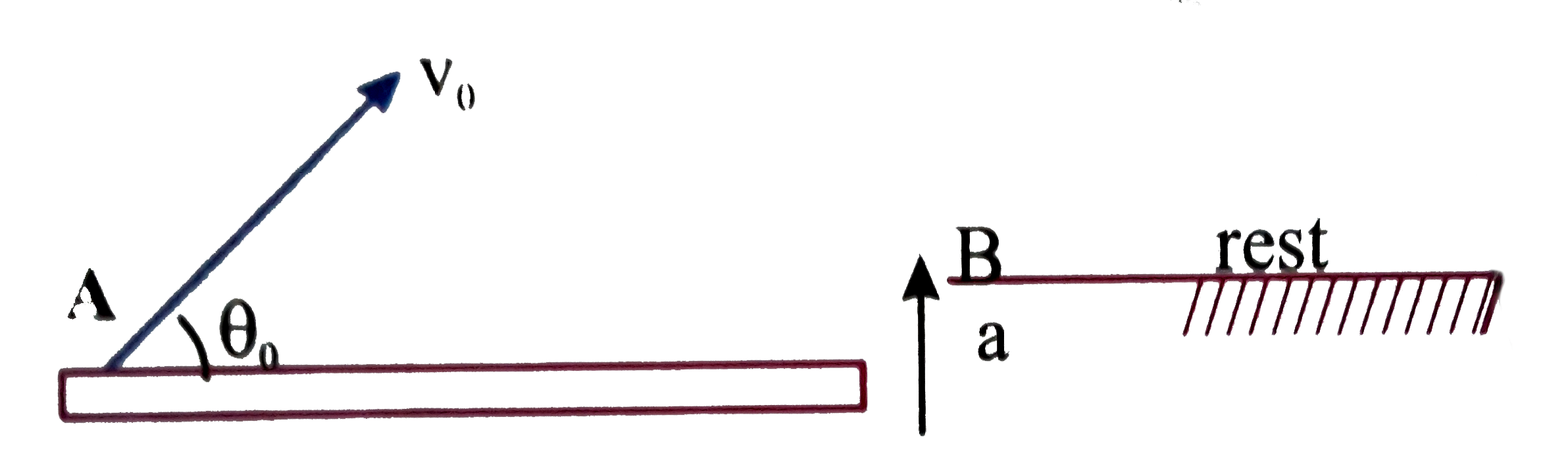
$< v_{0} at an angle θ < θ_{0}$

$> v_{0} at an angle θ > θ_{0}$

$> v_{0} at an angle θ = θ_{0}$

$v_{0} at an angle θ = θ_{0}$

Text Solution

Verified by Experts

The correct Answer is:D

Here $B$ is ground frame, w.r.t $B$ projection velocity will be greater than $V_{0}$ . Hence when $θ > θ_{0}$ projectile velocity will be greater than $V_{0}$ .

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Topper's Solved these Questions

Knowledge Check

When we analyse the projectile motion from any accelerated frame $O$ as ${\to r}_{o}, {\to u}_{o}$ and ${\to a}_{o}$ respectively, express the following terms, ${\to r}_{p O} + {\to a}_{p} - {\to r}_{O}, {\to u}_{p O} = {\to u}_{p} - {\to u}_{O}$ and ${\to a}_{p O} = {\to a}_{p} - {\to a}_{O}$
where $P$ stands for projectile. Then using the following kinematical equations of the projectile (For constant acceleration) relative to the accelerating frame ,we have ${\to S}_{p O} = {\to u}_{p O} t + \frac{1}{2} {\to a}_{p O} t^{2}, {\to v}_{p O}$
$= {\to u}_{p O} + {\to a}_{p O} t$ and $v_{p O}^{2} = u_{p O}^{2} + 2 \to a . {\to s}_{p} O$
Using the above expressions, anwer the following
question:A projectile has initial velocity $v_{0}$ realtive to the large plate which is moving with a constant upward acceleration a.
Which of the following remains equal for the observers $A$ and $B$ ?

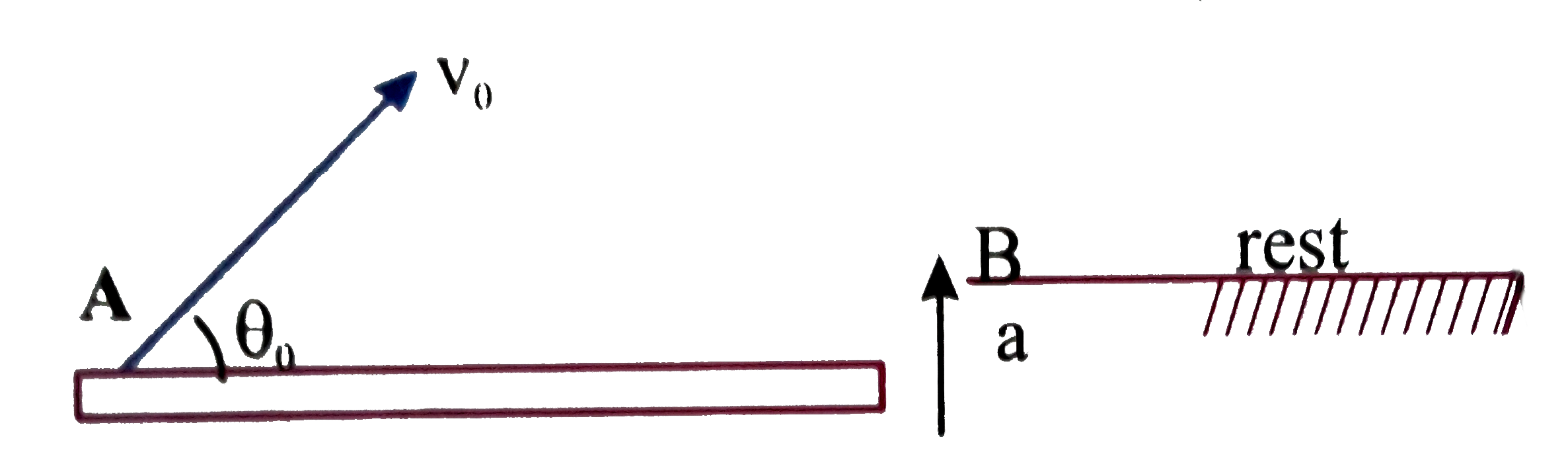AMaximum height
BRange
CTime of flight
DAngle of projection
Let $\to a$ and $\to b$ be two non-collinear unit vectors. If $\to u = \to a - (\to a . \to b) \to b$ and $\to v = \to a \times \to b$ , then $| \to v |$ is
A $| \to u | + ∣ ∣ \to u . (\to a \times \to b) ∣ ∣$
B $| \to u | + | \to u . \to a |$
C $| \to u | + ∣ ∣ \to u . \to b ∣ ∣$
D $| \to u | + \to u . (\to a + \to b)$
Consider $H_{3} P O_{2}, H_{3} P O_{3}, H_{3} P O_{4} and H_{4} P_{2} O_{7}$ . Which of the above oxoacids results into two series of salts ?
A $H_{3} P O_{2}$
B $H e_{3} P O_{3}$
C $H_{3} P O_{4}$
D $H_{4} P_{2} O_{7}$

NARAYNA-MOTION IN A PLANE-Level-VI Passage answer

Home
Ask Doubt
Profile

The correct Answer is:D

HereB is ground frame, w.r.t B projection velocity will be greater than V0. Hence when θ>θ0 projectile velocity will be greater than V0.

Topper's Solved these Questions

Similar Questions

Knowledge Check

Let →a and →b be two non-collinear unit vectors. If →u=→a−(→a.→b)→b and →v=→a×→b, then |→v| is

Consider H3PO2,H3PO3,H3PO4andH4P2O7 . Which of the above oxoacids results into two series of salts ?

NARAYNA-MOTION IN A PLANE-Level-VI Passage answer

Here $B$ is ground frame, w.r.t $B$ projection velocity will be greater than $V_{0}$ . Hence when $θ > θ_{0}$ projectile velocity will be greater than $V_{0}$ .

Let $\to a$ and $\to b$ be two non-collinear unit vectors. If $\to u = \to a - (\to a . \to b) \to b$ and $\to v = \to a \times \to b$ , then $| \to v |$ is

Consider $H_{3} P O_{2}, H_{3} P O_{3}, H_{3} P O_{4} and H_{4} P_{2} O_{7}$ . Which of the above oxoacids results into two series of salts ?