A polynomial function is everyehere continuous.
Step by step video & image solution for A polynomial function is everyehere continuous. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
Assertion (A) : A continuous function is always differentiable.
Reason (R ) : A differentiable function is always continuous.ABoth A and R are true and R is the correct explanation of ABBoth A and R are true but R is NOT the correct explanation of ACA is true but R is falseDA is false and R is true
Which of the following is not true?
(A) A polynomial function is always continuous
(B) A continuous function is always differentiable
(C) A differentiable function is always continuous
(D) None of these
View SolutionA constant and identity function is everywhere continuous.
View SolutionThe graph of a polynomial function is a smooth continuous curve. By looking at graph, we can find the number of zeros of the polynomial. Graphs are the geometrical meaning of the polynomials. They help us to understand their type, nature of its zeroes and coefficients of its various terms.
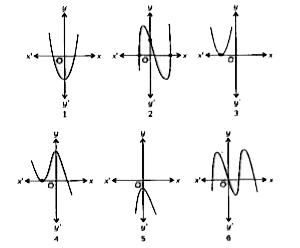
Which of the above graph represents quadratic polynomials?
View SolutionThe exponential function ax;a>0 is everywhere continuous.
View SolutionProve that every rational function is continuous.
View SolutionDifferentiation OF polynomial function and Inverse function , Illustration
View SolutionThe composition of two continuous functions is a continuous function.
View Solution
