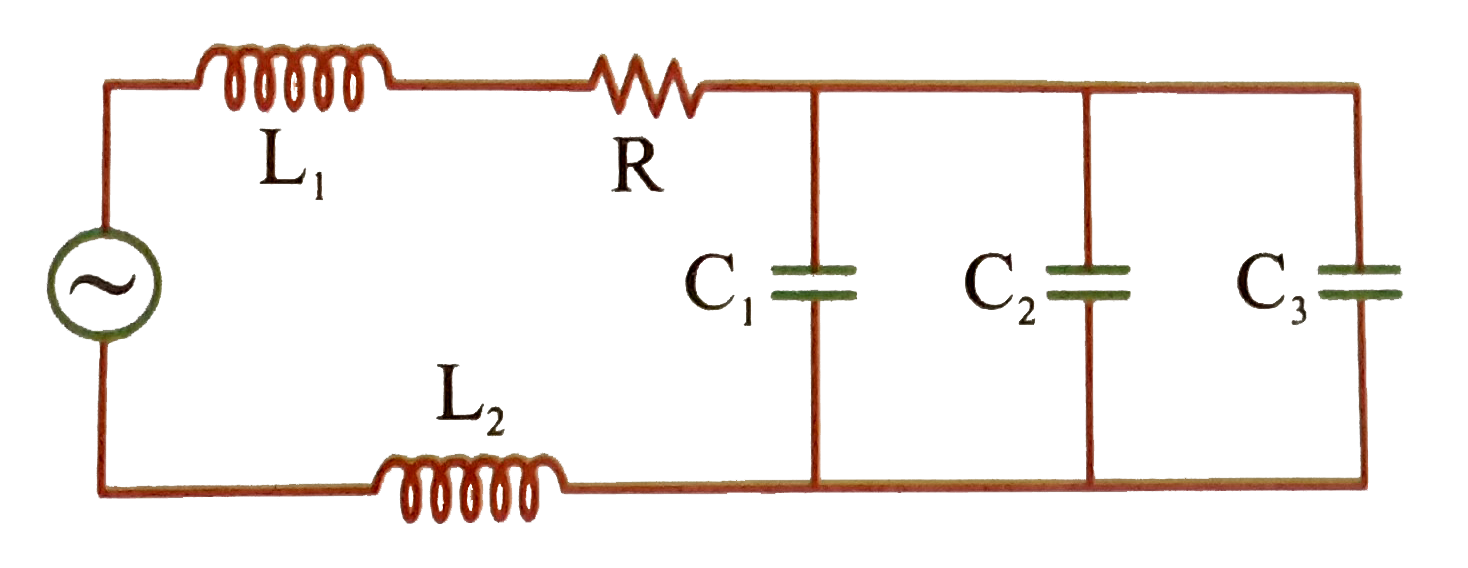
A generator with an adjustable frequency of oscillaton is connected to resistance, R=1000Ω, inductance, L1=1.7mH and L2=2.3mH and capacitance, C1=4μF,C2=2.5μF and C3=3.5μF. The resonant angular frequency of the circuit is
0.5rad/s
0.5×104rad/s
2rad/s
2×10−4rad/s
The correct Answer is:B
Ceff=C1+C2+C3
=4μF+2.5μF+3.5μF=10μF
Leff=L1+L2=1.7mH+2.3mH=4mH
Resonance frequency,
ω=1√LeffCeff=1√4×10−3×10×10−6
1042=0.5×104rad/s
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - VI120videos
ALTERNATING CURRENT
- BOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - I (H.W)23videos
ALTERNATING CURRENT
- BOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - IV NCERT Based Questions15videos
ALTERNATING CURRENT
- BOOK - NARAYNACHAPTER - ATOMIC PHYSICSEXERCISE - LEVEL-II (H.W)14videos
ATOMIC PHYSICS
Similar Questions
An inductance of 1mH and a charged capacitor capacitance of 10 mu F are connected across each other.The resonating angular frequency of circuit is
View SolutionIn a LCR circuit having L=8.0 henry, C=0.5 mu F and R=100ohm in series.The resonance angular frequency in radian per second is
View Solution
Knowledge Check
- Question 1 - Select One
If resistance R =10Ω, inductance L = 2 mH and capacitance C=5μF are connected in series to an AC source of frequency 50 Hz, then at resonance the impedance of circuit is
AZeroB10ΩC1000ΩD10KΩ - Question 2 - Select One
When L=2H,C=32μF and R=10Ω , then the resonant frequency is:
A125rads−1B140rads−1C130rads1D135rads−1 - Question 3 - Select One
Find the time constant (in μs) for the given RC circuits in the given order respectively.
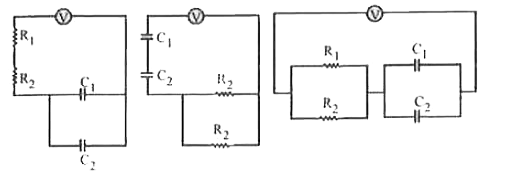
R1=1ω,R2=2ω,C1=4μF,C2=2μFA18,4,89B18,89,4C4,18,89D4,89,18
An AC circuit has R= 100Ω , C= 2μ and L= 80 mH, connected in series. The quality factor of the circuit is :
View SolutionIn LC oscillation resistance is 100 Ω and inductance and capacitance is 1 H and 10μF . Find the half power of frequency .
View SolutionThe frequency for which a 5μF capacitor has a reactance of 11000Ωis given by
View SolutionIn the arrangement C1=10μ,FC2=10μF and C3=20μF . What is the equivalent capacitance ( in μF ) between points A and B ?
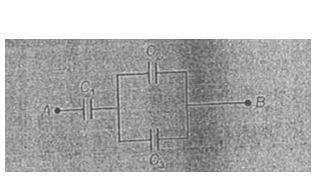 View Solution
View SolutionIf resistance R =100 Omega, inductance L = 12mH and capacitance C=15muF are connected in series to an AC sources, then at resonance the impedance of circuit is
View Solution

