O is the origin in the Cartesian plane. From the origin O take point A in the North-East direction such that |OA|=5,B is a point in the North-West direction such that |OB|=5.Then |OA−OB| is (A) 25 (B) 5√2 (C) 10√5 (D) √5
Step by step video & image solution for O is the origin in the Cartesian plane. From the origin O take point A in the North-East direction such that |OA| =5, B is a point in the North-West direction such that |OB| = 5.Then |OA-OB| is (A) 25 (B) 5sqrt2 (C) 10sqrt5 (D) sqrt5 by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
If a has magnitude 5 and points North-East and vector b has magnitude 5 and point North-West, then |a-b| is equal to
A25B5C7√3D5√2A point O lies inside a square ABCD such that OA=3, OB=4 and OD=5, find the value of OC.
AA)3√3BB)6.67CC)3√2DD)4√2Two points A and B in a plane are related if OA = OB, where O is a fixed point. This relation is
Apartial order relationBequivalence relationCreflexive but not symmetricDreflexive but not transitive
If O is the origin and the coordinates of A are (a,b,c) . Find the direction cosines of OA and the equation of the plane through A at right angles to OA.
View SolutionIf O is the origin and the coordinates of A are (a,b,c) . Find the direction cosines of OA and the equation of the plane through A at right angles to OA.
View Solution50 A current in north to south direction. Give the magnitude and direction of →B at a point2.5 m east of the wire.
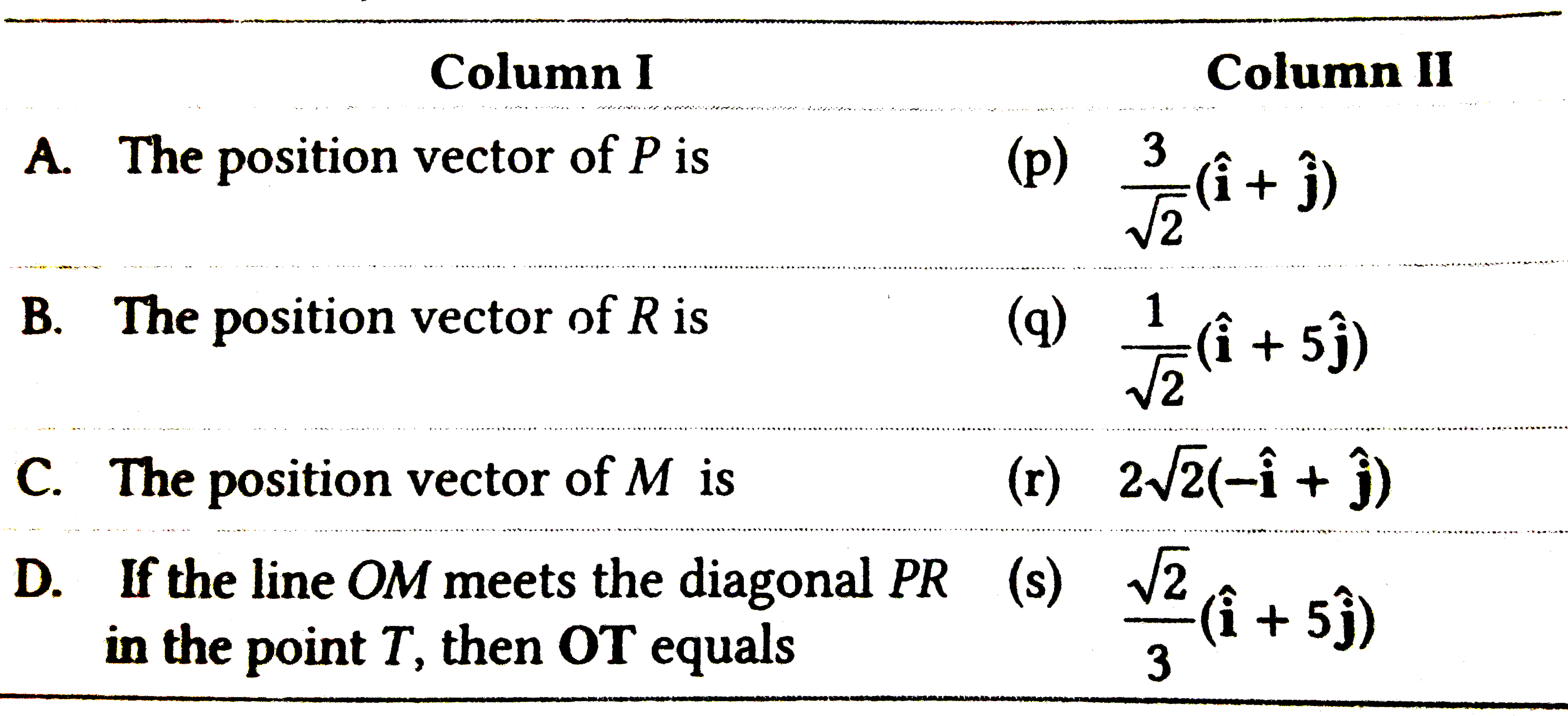
View SolutionIn the Cartesian plane, a man starts at origin and walks a distance of 3 units of the north-east direction and reaches a point P. from P, he walks a distance of 4 units in the north-west direction to reach a point Q. construct the parallelogram OPQR with OP and PQ as adjacent sides. let M be the mid-point of PQ.
View SolutionA line 2x+y=1 intersect co-ordinate axis at points A and B. A circle is drawn passing through origin and point A & B. If perpendicular from point A and B are drawn on tangent to the circle at origin then sum of perpendicular distance is (A) 5√2 (B) √52 (C) √54 (D) 52
View Solution
