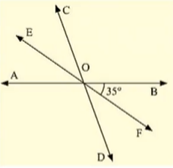
AB, CD, and EF
are three concurrent
lines passing through the point O
such that OF
bisects ∠BOD.
If ∠BOF=350,
find ∠BOC
and ∠AOD.
<AB, CD, and EFare three concurrent lines= passing through the point O.
∠dof=∠bof=350
Also AB is straight line ,∠AOD+∠DOF+∠BOF=180
∠AOD+35+35=180
∠AOD=180−70=110
∠AOD=∠BOC
/_BOC=110
Step by step video & image solution for A B ,\ C D ,\ a n d\ E F are three concurrent lines passing through the point O such that O F bisects /_B O Ddot If /_B O F=35^0, find /_B O C and /_A O Ddot<A B ,\ C D ,\ a n d\ E Fare three concurrent lines= passing through the point O./_dof=/_bof=35^0Also AB is straight line ,/_AOD+/_DOF+/_BOF=180/_AOD+35+35=180/_AOD=180-70=110/_AOD=/_BOC/_BOC=110 by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
AB, CD, and EF are three concurrent lines passing through the point O such that OF bisects ∠BOD. If ∠BOF=350, find ∠BOC and ∠AOD.
<AB, CD, and EFare three concurrent lines= passing through the point O.
∠dof=∠bof=350
Also AB is straight line ,∠AOD+∠DOF+∠BOF=180
∠AOD+35+35=180
∠AOD=180−70=110
∠AOD=∠BOC
/_BOC=110

View SolutionTwo lines AB and CD intersect at O .If "∠AOC=50∘, find ∠AOD,∠BOD and ∠BOC
View SolutionLine segments AB and CD intersect at O such that AC∣∣DB . If ∠CAB=350 and ∠CDB=550 find the ∠BOD.
View SolutionIn Fig. 6.13, lines AB and CD intersect at O. If ∠AOC +∠BOE=70∘and ∠BOD=40∘, find ∠BOE and reflex ∠COE.
View SolutionThe diagonals of a rectangle ABCD meet at O . If ∠BOC=44∘, find ∠OAD
View SolutionABCD is a parallelogram in which diagonal AC bisects ∠BAD. If ∠BAC=35∘ , then ∠ABC= 70∘ (b) 110∘ (c) 90∘ (d) 120∘
View Solution.In the adjoining figure,three coplanar linesAB,CDandEFintersect at a point O . Find the value of x . Hence, find ∠AOD,∠COEand∠AOE
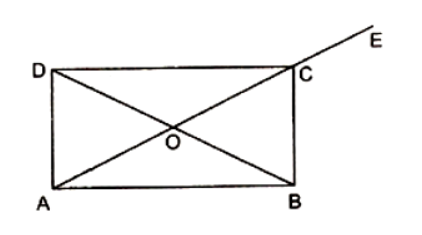
View SolutionIn Figure, ABCD is a rectangle in which diagonal AC is produced to E. If ∠ECD=1460, find ∠AOB
View SolutionIn Fig.81, side BC of ABC has been produced to D and CEBA. If ∠ABC=650, ∠BAC=550, find ∠ACE, ∠ECD and ∠ACD.
View SolutionLine segments AB and CD intersect at O such that AC∣∣DB. If ∠CAB=450and ∠CDB=550, then ∠BOD= 1000 (b) 800 (c) 900 (d) 1350
View Solution
