For a gaseous reaction 2A+B2→2AB, the following rate data were obtained at 300 K
Rate of disappearance of B2 Concentration(mol lit−1min−1)[A][B2](i)1.8×10−30.0150.15(ii)1.08×10−20.090.15(iii)5.4×10−30.0150.45
Calculate the rate constant for the reaction and rate of formation of AB when [A] is 0.02 and [B2] is 0.04 "mol lit"^(-1)" at 300 K".
The correct Answer is:k=0.8 litre mol−1−1min and required rate of formation =1.28×10−3 mol lit−1−1min
To solve the problem, we will follow these steps:
Step 1: Write the rate law expression
The rate law for the reaction 2A+B2→2AB can be expressed as:
Rate=k(A)α(B2)β
where k is the rate constant, and α and β are the orders of the reaction with respect to A and B2, respectively.
Step 2: Determine the orders of reaction (α and β)
We will use the provided rate data to find the values of α and β.
Using Experiments (i) and (iii):
R1R3=k(A)α1(B2)β1k(A)α3(B2)β3
Substituting the values:
1.8×10−35.4×10−3=0.015α⋅0.15β0.015α⋅0.45β
This simplifies to:
1.85.4=0.15β0.45β
13=(0.150.45)β
13=(13)β
Thus, β=1.
Using Experiments (i) and (ii):
R1R2=k(A)α1(B2)β1k(A)α2(B2)β2
Substituting the values:
1.8×10−31.08×10−2=0.015α⋅0.15β0.09α⋅0.15β
This simplifies to:
1.810.8=0.015α0.09α
16=(0.0150.09)α
16=(16)α
Thus, α=1.
Step 3: Write the complete rate law
Now we can express the rate law as:
Rate=k(A)(B2)
Step 4: Calculate the rate constant k
Using the first experiment data:
1.8×10−3=k(0.015)(0.15)
Solving for k:
k=1.8×10−30.015×0.15
k=1.8×10−30.00225=0.8mol−1litmin−1
Step 5: Calculate the rate of formation of AB
Using the rate law:
Rate=k(A)(B2)
Substituting k, (A)=0.02, and (B2)=0.04:
Rate=0.8×0.02×0.04
Rate=0.8×0.0008=0.00064mol lit−1min−1
Since the formation of AB is twice the rate of disappearance of B2:
Rate of formation of AB=2×0.00064=0.00128mol lit−1min−1
Final Answers:
- Rate constant k=0.8mol−1litmin−1
- Rate of formation of AB=0.00128mol lit−1min−1
The rate law for the reaction 2A+B2→2AB can be expressed as:
Rate=k(A)α(B2)β
where k is the rate constant, and α and β are the orders of the reaction with respect to A and B2, respectively.
We will use the provided rate data to find the values of α and β.
R1R3=k(A)α1(B2)β1k(A)α3(B2)β3
Substituting the values:
1.8×10−35.4×10−3=0.015α⋅0.15β0.015α⋅0.45β
This simplifies to:
1.85.4=0.15β0.45β
13=(0.150.45)β
13=(13)β
Thus, β=1.
R1R2=k(A)α1(B2)β1k(A)α2(B2)β2
Substituting the values:
1.8×10−31.08×10−2=0.015α⋅0.15β0.09α⋅0.15β
This simplifies to:
1.810.8=0.015α0.09α
16=(0.0150.09)α
16=(16)α
Thus, α=1.
Now we can express the rate law as:
Rate=k(A)(B2)
Using the first experiment data:
1.8×10−3=k(0.015)(0.15)
Solving for k:
k=1.8×10−30.015×0.15
k=1.8×10−30.00225=0.8mol−1litmin−1
Using the rate law:
Rate=k(A)(B2)
Substituting k, (A)=0.02, and (B2)=0.04:
Rate=0.8×0.02×0.04
Rate=0.8×0.0008=0.00064mol lit−1min−1
Since the formation of AB is twice the rate of disappearance of B2:
Rate of formation of AB=2×0.00064=0.00128mol lit−1min−1
- Rate constant k=0.8mol−1litmin−1
- Rate of formation of AB=0.00128mol lit−1min−1
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - PRADEEPCHAPTER - CHEMICAL KINETICSEXERCISE - CURIOSITY QUESTION
2 Videos
- CHEMICAL KINETICSBOOK - PRADEEPCHAPTER - CHEMICAL KINETICSEXERCISE - TEST YOUR GRIP (MULTIPLE CHOICE QUESTIONS)
25 Videos
- CHEMICAL KINETICSBOOK - PRADEEPCHAPTER - CHEMICAL KINETICSEXERCISE - ADVANCED PROBLEMS FOR COMPETITIONS
14 Videos
- BIOMOLECULES BOOK - PRADEEPCHAPTER - BIOMOLECULES EXERCISE - IMPORTANT QUESTIONS (FOR BOARD EXAMINATION)
25 Videos
- CHEMISTRY IN EVERYDAY LIFE BOOK - PRADEEPCHAPTER - CHEMISTRY IN EVERYDAY LIFE EXERCISE - IMPORTANT QUESTION FOR BOARD EXAMINATION
30 Videos
Similar Questions
form the gaseous reaction
2A+B2→2AB, the following rate data were obtained at 300K.
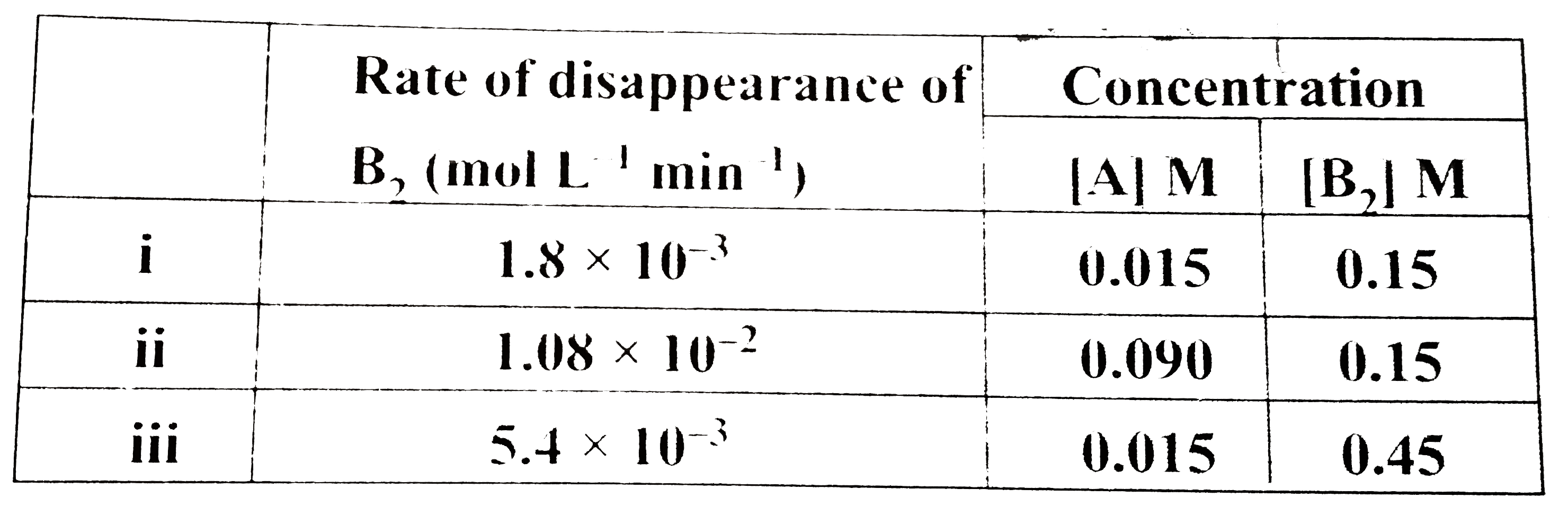
Calculate the rate constant for the reaction and the rate of formation of AB when [A] is 0.02 and [B2] is 0.04molL−1 at 300K.
View SolutionThe following data were obtained at 300K for the reaction 2A+B→C+D

Calculate the rate of formation of D when [A] = 0.5molL−1 and [B]=0.2molL−1
View Solution
Knowledge Check
form the gaseous reaction
2A+B2→2AB, the following rate data were obtained at 300K.
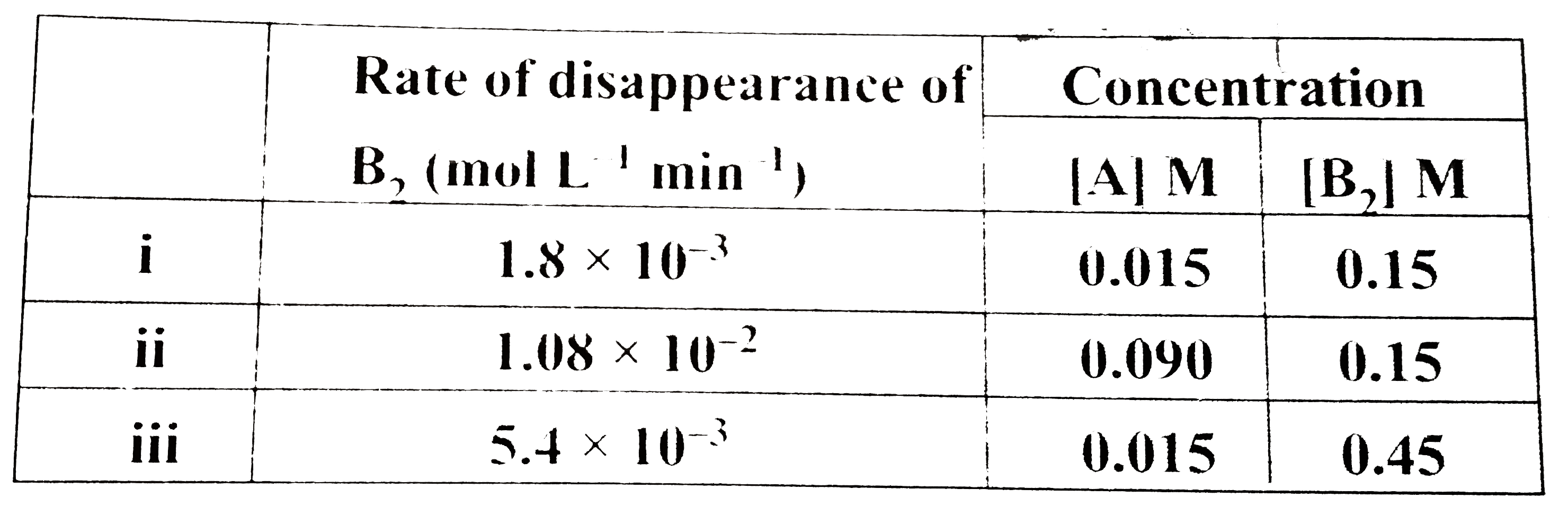
Calculate the rate constant for the reaction and the rate of formation of AB when [A] is 0.02 and [B2] is 0.04molL−1 at 300K.A0.5mol−1min−1litreB0.8mol−1min−1litreC1.5mol−1min−1litreD2mol−1min−1litreThe rate constant of a reaction is 0.69×10−1 and the initial concentration is 0.2mol l−1. The half-life period is
A400secB600secC800secD1200secFor a reaction A+2B→2C, the following data were obtained . Initial concentration
[A][B]Rate(moll−1−1min)i.1.01.00.15ii2.01.00.30iii.3.01.00.45iv.1.02.00.15v.1.03.00.15
The rate law for this reactionAR=k[A][B]0BR=k[A][B]2CR=k[A][B]DR=k[A]2[B]
For the reaction 2N2O5(g)→4NO2(g)+O2(g), the following results have been obtained :
S. No.[N2O5] mol L−1Rate of disappearance ofN2O5, mol L−1−1min11.13×10−234×10−520.84×10−225×10−530.62×10−218×10−5
(a) Calculate the order of reaction
(b) Write rate law
(c) Calculate rate constant of the reaction.
View SolutionThe following data are for the reaction A+B→ Products:
Conc.ofA(M)Conc.ofB(M)Initialrate(molL−1s−1)0.10.14.0×10−40.20.21.6×10−30.50.11.0×10−20.50.55.0×10−2
(a) What is the order with respect to A and B for the reaction?
(b) Calculate the rate constant
Determine the reaction rate when the concentrations of A and B are 0.2M and 0.35M respectvely.
View SolutionCalculate the order of the reaction in A and B :-
ABRate(mol/1)(mol/1)0.050.051.2×10−30.100.052.4×10−30.050.101.2×10−3
View SolutionFor the non-stoichiometric reaction 2A+B→C+D
The following kinetic data were obtained in theee separate experiment, all at 98K
∣∣ ∣ ∣ ∣ ∣∣Initial concentration (A)Initial concentration (B)Initial rate of formation of C(molL−1s−1)0.01M0.1M1.2×10−30.1M0.2M1.2×10−30.2M0.1M2.4×10−3∣∣ ∣ ∣ ∣ ∣∣
The rate law for the formation of C is:
View SolutionThe reaction of A2 and B2 follows the equation
A2(g)+B2(g)→2AB(g)
The following data were observed
[A2]0[B2]0Initial rate of appearance of AB(g)(inMs−10.100.102.5×10−40.200.105×10−40.200.2010×10−4
The value of rate constatnt for the above reaction is :
View Solution
