The difference of energy between two sets of d-orbitals obtained due to splitting in a crystal field is called …… .
To answer the question about the difference of energy between two sets of d-orbitals due to splitting in a crystal field, we can follow these steps:
1. Understanding d-Orbital Splitting:
In coordination chemistry, when transition metal ions are surrounded by ligands, the degeneracy of the d-orbitals is lifted, leading to a splitting of these orbitals into different energy levels. This is due to the electrostatic interactions between the d-electrons and the electric fields created by the surrounding ligands.
2. Identifying the Splitting Pattern:
The d-orbitals split into two main groups in an octahedral field: the lower energy set (often labeled as t2g) and the higher energy set (labeled as eg). In a tetrahedral field, the splitting is reversed, with eg being lower and t2g being higher.
3. Defining the Energy Difference:
The energy difference between these two sets of orbitals is a crucial concept in crystal field theory. It quantifies how much energy is required to promote an electron from the lower energy t2g orbitals to the higher energy eg orbitals.
4. Naming the Energy Difference:
The specific term used to describe this energy difference is the Crystal Field Splitting Energy (CFSE). This term reflects the energy stabilization that occurs when the d-electrons occupy the lower energy orbitals.
5. Conclusion:
Therefore, the difference of energy between the two sets of d-orbitals obtained due to splitting in a crystal field is called Crystal Field Splitting Energy (CFSE).
Final Answer:
The difference of energy between two sets of d-orbitals obtained due to splitting in a crystal field is called Crystal Field Splitting Energy (CFSE).
---
In coordination chemistry, when transition metal ions are surrounded by ligands, the degeneracy of the d-orbitals is lifted, leading to a splitting of these orbitals into different energy levels. This is due to the electrostatic interactions between the d-electrons and the electric fields created by the surrounding ligands.
The d-orbitals split into two main groups in an octahedral field: the lower energy set (often labeled as t2g) and the higher energy set (labeled as eg). In a tetrahedral field, the splitting is reversed, with eg being lower and t2g being higher.
The energy difference between these two sets of orbitals is a crucial concept in crystal field theory. It quantifies how much energy is required to promote an electron from the lower energy t2g orbitals to the higher energy eg orbitals.
The specific term used to describe this energy difference is the Crystal Field Splitting Energy (CFSE). This term reflects the energy stabilization that occurs when the d-electrons occupy the lower energy orbitals.
Therefore, the difference of energy between the two sets of d-orbitals obtained due to splitting in a crystal field is called Crystal Field Splitting Energy (CFSE).
The difference of energy between two sets of d-orbitals obtained due to splitting in a crystal field is called Crystal Field Splitting Energy (CFSE).
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - CORDINATION COMPOUNDSEXERCISE - Conceptual Questions51videos
CORDINATION COMPOUNDS
- BOOK - PRADEEPCHAPTER - CORDINATION COMPOUNDSEXERCISE - NCERT INTEXT QUESTIONS18videos
CORDINATION COMPOUNDS
- BOOK - PRADEEPCHAPTER - CORDINATION COMPOUNDSEXERCISE - Test Your Grip29videos
CORDINATION COMPOUNDS
- BOOK - PRADEEPCHAPTER - CHEMISTRY IN EVERYDAY LIFE EXERCISE - IMPORTANT QUESTION FOR BOARD EXAMINATION30videos
CHEMISTRY IN EVERYDAY LIFE
- BOOK - PRADEEPCHAPTER - D- AND F-BLOCK ELEMENTSEXERCISE - IMPORTANT QUESTIONS30videos
D- AND F-BLOCK ELEMENTS
Similar Questions
Knowledge Check
- Question 1 - Select One
According to crystal field theory d-orbitals split up in octahedral field into two sets.dxydyxdzx have lower energy and dx2−y2 and dz2 have higher energy. The difference in energy of these two sets of d-orbitals is called crystal field splitting energy denoted by Δ0.
In tetrahedral field dx2−y2 and dz2 have lower energy whereas dxydyzdzx have higher energy. The difference in energy is denoted Δt.
Δ0 can be determined by measuring λmax for absorption and converting into energy units. Δ0 depends upon nature of metal ions as well as nature of ligands. The magnitude of Δ0 also decides low energy levels are filled.
which one of the following has the highest magnetic momentAd2xyd2yzd1zxBd1xyd1yzd1zxdx2−y2d1z2Cd2xyd1yzd1zxd1x2−y2Dd2xyd1yzd1zxd2x2−y2 - Question 2 - Select One
According to crystal field theory d-orbitals split up in octahedral field into two sets.dxydyxdzx have lower energy and dx2−y2 and dz2 have higher energy. The difference in energy of these two sets of d-orbitals is called crystal field splitting energy denoted by Δ0.
In tetrahedral field dx2−y2 and dz2 have lower energy whereas dxydyzdzx have higher energy. The difference in energy is denoted Δt.
Δ0 can be determined by measuring λmax for absorption and converting into energy units. Δ0 depends upon nature of metal ions as well as nature of ligands. The magnitude of Δ0 also decides low energy levels are filled.
which one of the following has the highest magnetic momentA[CoF6]3−B[Ni(H2O)6]2+C[Fe(CN)6]3−D[Cu(NH3)4]2+ - Question 3 - Select One
According to crystal field theory d-orbitals split up in octahedral field into two sets.dxydyxdzx have lower energy and dx2−y2 and dz2 have higher energy. The difference in energy of these two sets of d-orbitals is called crystal field splitting energy denoted by Δ0.
In tetrahedral field dx2−y2 and dz2 have lower energy whereas dxydyzdzx have higher energy. The difference in energy is denoted Δt.
Δ0 can be determined by measuring λmax for absorption and converting into energy units. Δ0 depends upon nature of metal ions as well as nature of ligands. The magnitude of Δ0 also decides low energy levels are filled.
Given the following data about absorption maximum of several complex ions, the correct order of Δ0 for these ions is
Complexes λmax
[Cr(H2O)6]3+694nm
[Cr(NH3)6]3+465nm
[CrC16]3− 758nmAΔ0[Cr(NH3)6]3+>Δ0[Cr(H2O)6]3+>Δ0[CrC16]3−BΔ0[Cr(NH3)6]3+=Δ0[Cr(H2O)6]3+>Δ0[CrC16]3−CΔ0[Cr(NH3)6]3+<Δ0[Cr(H2O)6]3+<Δ0[CrC16]3−DΔ0[Cr(N2O)6]3+>Δ0[CrC16]3−>Δ0[Cr(NH3)6]3+
The difference in energy between the molecule orbital formed and the combining atomic orbitals is called
View SolutionCrystal field theory views the bonding in complexes as arising from electrostatic interaction and considers the effect of the ligand charges on the energies of the metal ion d-orbitals
In this theory, a ligand lone pair is modelled as a point negative charge that repels electrons in the d-orbitals of the central metal ion.The theory concentrated on the resulting splitting of the d-orbitals in two groups with different energies and used that splitting to rationalize and correlate the optical spectra,thermodynamic stability, and magnetic properties of complexes.This energy splitting between the two sets of d-orbitals is called the crystal field splitting Δ.
In general, the crystal field splitting energy Δ corresponds to wavelenght of light in visible region of the spectrum, and colours of the complexes can therefore be attributed to electronic transition between the lower and higher energy sets of d-orbitals.
In general, the colour that we see is complementry to the colour absorbed.

Different metal ions have different value of Δ, which explains why their complexes with the same ligand have different colour.
Similarly the crystal field splitting also depends on the nature of ligands and as the ligand for the same metal varies from H2O to NH3 to ethylenediamine, Δ for complexes increase.Accordingly, the electronic transition shifts to higher energy (shorter wavelength) as the ligand varies from H2O from NH3 to en, thus accounting for the variation in colour.
Crystal field theory accounts for the magnetic properties of complexes in terms of the relative values of Δ and the spin pairing energy P. Small Δ values favour high spin complexes, and large Δ values favour low spin complexes.
The [Ti(NCS)6]3− ion exhibits a single absorption band at 544 nm.What will be the crystal field splitting energy (in kJ mol−1) of the complex ? (h=6.626×10−34J.s,C=3.0×105m/s,NA=6.02×1023 ions/mole.View SolutionCrystal field theory views the bonding in complexes as arising from electrostatic interaction and considers the effect of the ligand charges on the energies of the metal ion d-orbitals
In this theory, a ligand lone pair is modelled as a point negative charge that repels electrons in the d-orbitals of the central metal ion.The theory concentrated on the resulting splitting of the d-orbitals in two groups with different energies and used that splitting to rationalize and correlate the optical spectra,thermodynamic stability, and magnetic properties of complexes.This energy splitting between the two sets of d-orbitals is called the crystal field splitting Δ.
In general, the crystal field splitting energy Δ corresponds to wavelenght of light in visible region of the spectrum, and colours of the complexes can therefore be attributed to electronic transition between the lower and higher energy sets of d-orbitals.
In general, the colour that we see is complementry to the colour absorbed.
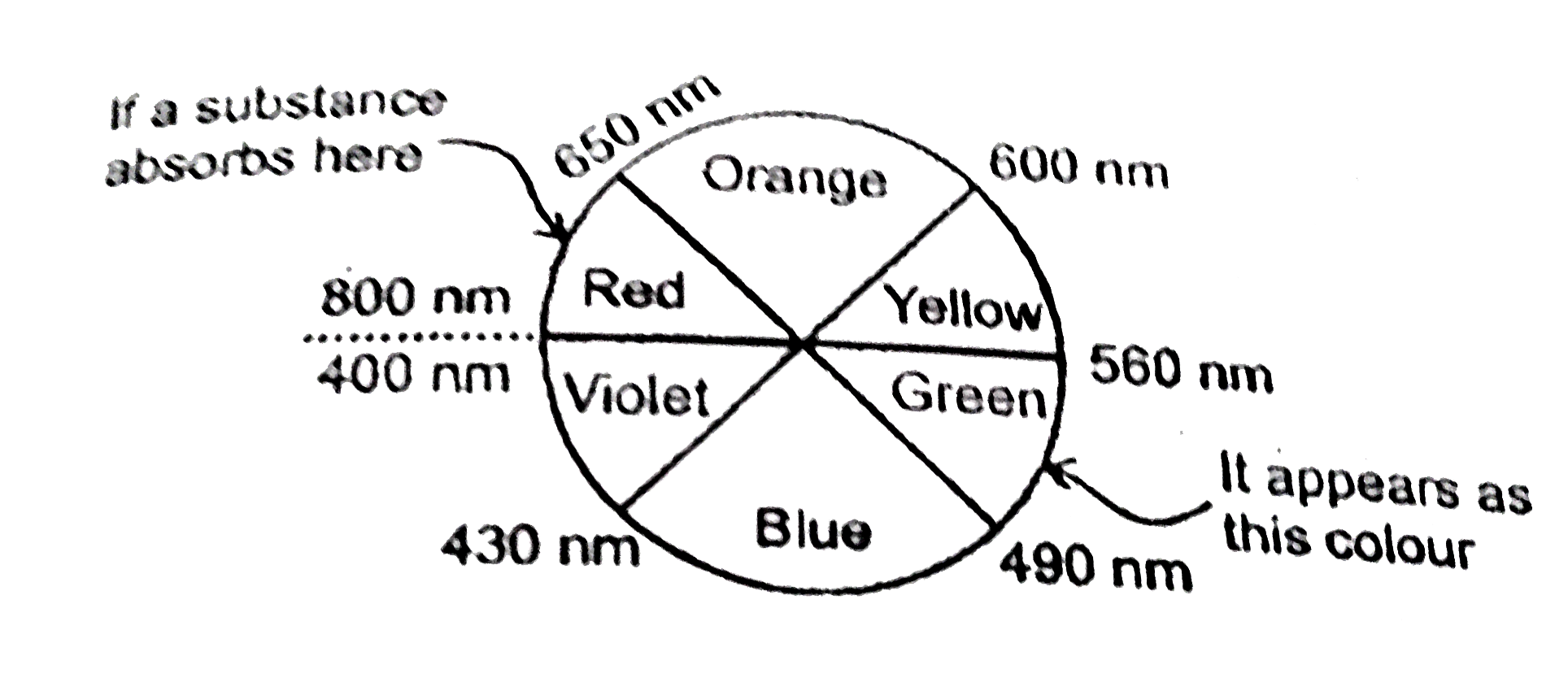
Different metal ions have different value of Δ, which explains why their complexes with the same ligand have different colour.
Similarly the crystal field splitting also depends on the nature of ligands and as the ligand for the same metal varies from H2O to NH3 to ethylenediamine, Δ for complexes increase.Accordingly, the electronic transition shifts to higher energy (shorter wavelength) as the ligand varies from H2O from NH3 to en, thus accounting for the variation in colour.
Crystal field theory accounts for the magnetic properties of complexes in terms of the relative values of Δ and the spin pairing energy P. Small Δ values favour high spin complexes, and large Δ values favour low spin complexes.
Which of the following complexes are diamagnetic ?
[Pt(NH3)4]2+[Co(SCN)4]2−[Cu(en)2]2+[HgI4]2−square planartetrahedralsquare planartetrahedral(i)(ii)(iii)ivView SolutionCrystal field theory views the bonding in complexes as arising from electrostatic interaction and considers the effect of the ligand charges on the energies of the metal ion d-orbitals
In this theory, a ligand lone pair is modelled as a point negative charge that repels electrons in the d-orbitals of the central metal ion.The theory concentrated on the resulting splitting of the d-orbitals in two groups with different energies and used that splitting to rationalize and correlate the optical spectra,thermodynamic stability, and magnetic properties of complexes.This energy splitting between the two sets of d-orbitals is called the crystal field splitting Δ.
In general, the crystal field splitting energy Δ corresponds to wavelenght of light in visible region of the spectrum, and colours of the complexes can therefore be attributed to electronic transition between the lower and higher energy sets of d-orbitals.
In general, the colour that we see is complementry to the colour absorbed.
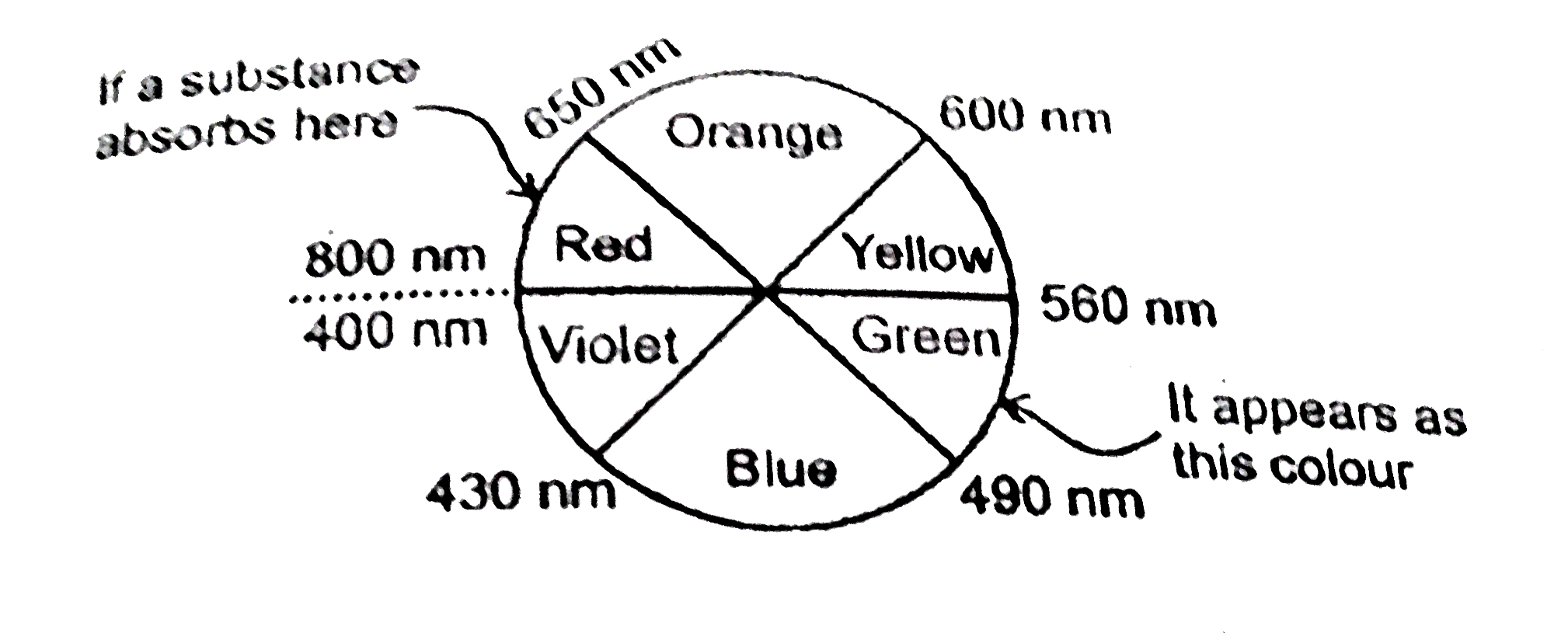
Different metal ions have different value of Δ, which explains why their complexes with the same ligand have different colour.
Similarly the crystal field splitting also depends on the nature of ligands and as the ligand for the same metal varies from H2O to NH3 to ethylenediamine, Δ for complexes increase.Accordingly, the electronic transition shifts to higher energy (shorter wavelength) as the ligand varies from H2O from NH3 to en, thus accounting for the variation in colour.
Crystal field theory accounts for the magnetic properties of complexes in terms of the relative values of Δ and the spin pairing energy P. Small Δ values favour high spin complexes, and large Δ values favour low spin complexes.
Which of the following statements is incorrect ?View SolutionIn the influence of electric field the splitting of spectral lines is called
View Solution

