Two men on either side of the cliff 80m high observes the angles of elevation of the top of the cliff to be 30o and 60o respectively. Find the distance between the two men.
To solve the problem, we will use trigonometric ratios to find the distances from each man to the base of the cliff. Let's denote the height of the cliff as XY=80 m, the distance from the first man to the base of the cliff as AY=a, and the distance from the second man to the base of the cliff as BY=b.
1. Identify the Angles and Heights:
- The height of the cliff XY=80 m.
- The angle of elevation from the first man (let's call him Man A) to the top of the cliff is 30∘.
- The angle of elevation from the second man (let's call him Man B) to the top of the cliff is 60∘.
2. Set Up the Right Triangle for Man A:
- For Man A, we can use the tangent function:
tan(30∘)=XYAY=80a
- We know that tan(30∘)=1√3. Therefore:
1√3=80a
- Rearranging gives:
a=80√3
3. Set Up the Right Triangle for Man B:
- For Man B, we again use the tangent function:
tan(60∘)=XYBY=80b
- We know that tan(60∘)=√3. Therefore:
√3=80b
- Rearranging gives:
b=80√3=80√33
4. Calculate the Total Distance Between the Two Men:
- The total distance D between the two men is the sum of a and b:
D=a+b=80√3+80√33
- To add these fractions, we need a common denominator:
D=240√33+80√33=240√3+80√33=320√33
5. Final Answer:
- The distance between the two men is:
D=320√33 meters
- The height of the cliff XY=80 m.
- The angle of elevation from the first man (let's call him Man A) to the top of the cliff is 30∘.
- The angle of elevation from the second man (let's call him Man B) to the top of the cliff is 60∘.
- For Man A, we can use the tangent function:
tan(30∘)=XYAY=80a
- We know that tan(30∘)=1√3. Therefore:
1√3=80a
- Rearranging gives:
a=80√3
- For Man B, we again use the tangent function:
tan(60∘)=XYBY=80b
- We know that tan(60∘)=√3. Therefore:
√3=80b
- Rearranging gives:
b=80√3=80√33
- The total distance D between the two men is the sum of a and b:
D=a+b=80√3+80√33
- To add these fractions, we need a common denominator:
D=240√33+80√33=240√3+80√33=320√33
- The distance between the two men is:
D=320√33 meters
Topper's Solved these Questions
Similar Questions
Two men on either side of the cliff 80m high observes the angles of elevation of the top of the cliff to be 30o and 60o respectively. Find the distance between the two men.
View SolutionTwo men on either side of a temple 126 m high observe the angle of elevation of the top of the temple to be 30∘and60∘ respectively. Find the distance between the two men ?
View SolutionTwo men on either side of a tower 75 m high observe the angle of elevation of the top of the tower to be 30° and 60°. What is the distance between the two men ?
View SolutionA man on the top of a cliff 100 meter high, observes the angles of depression of two points on the opposite sides of the cliff as 30∘ and 60∘ respectively. Find the difference between the two points.
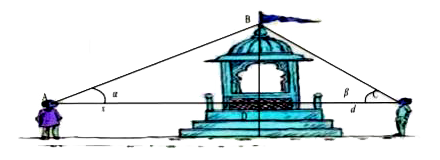
View SolutionTwo men on either side of a temple of 30 meters high observe its top at the angles of elevation? α and beta respectively. (as shown in the figure above). The distance between the two men is 40√3 meters and the distance between the first person A and the temple is30√3 meters. Based on the above information answer the following:

∠CAB=α=
View SolutionTwo men on either side of a temple of 30 meters high observe its top at the angles of elevation α and β respectively. (as shown in the figure above). The distance between the two men is 40√3 meters and the distance between the first person A and the temple is30√3 meters. Based on the above information answer the following:

∠CAB=α=
View SolutionTwo men on either sideof a 75 m high building and in line with base of buildig observe the angle of elevation of the top of the building as 30∘ and 60∘. Find the distance between the two men. (Use √3=1.73)
View SolutionTwo men on either side of a temple of 30 meters high observe its top at the angles of elevation α and β? respectively. (as shown in the figure above). The distance between the two men is 40√3 meters and the distance between the first person A and the temple is30√3 meters. Based on the above information answer the following:
Domain and Range of cos−1x=
View SolutionTwo men on either side of a temple of 30 meters high observe its top at the angles of elevation α and β? respectively. (as shown in the figure above). The distance between the two men is 40√3 meters and the distance between the first person A and the temple is30√3 meters. Based on the above information answer the following:

∠ABC=
View SolutionTwo men on either side of a temple of 30 meters high observe its top at the angles of elevation α and β? respectively. (as shown in the figure above). The distance between the two men is 40√3 meters and the distance between the first person A and the temple is30√3 meters. Based on the above information answer the following:

∠BCA=β=
View Solution
