An aeroplane is flying at a height of 210 m. Flying at this height at some instant the angles of depression of two points in a line in opposite directions on both the banks of the river are 45∘ and 60∘. Find the width of the river. (Use √3=1.73)
To find the width of the river based on the given information, we can follow these steps:
Step 1: Understand the problem
An aeroplane is flying at a height of 210 m. The angles of depression to two points on opposite banks of the river are 45° and 60°. We need to find the width of the river.
Step 2: Draw a diagram
Draw a horizontal line representing the height of the aeroplane (210 m). Mark point P as the position of the aeroplane. Draw two lines from point P down to the ground at angles of depression of 45° and 60° to points A and B on the opposite banks of the river.
Step 3: Label the distances
Let:
- AD=x (distance from point A directly below the plane to the point on the bank)
- DB=y (distance from point B directly below the plane to the point on the bank)
- The width of the river AB=x+y
Step 4: Use trigonometry for angle 45°
For triangle PDA:
- The height PD=210 m
- Angle ∠DAP=45°
Using the tangent function:
tan(45°)=PDAD=210x
Since tan(45°)=1:
1=210x⟹x=210 m
Step 5: Use trigonometry for angle 60°
For triangle PDB:
- The height PD=210 m
- Angle ∠DBP=60°
Using the tangent function:
tan(60°)=PDDB=210y
Since tan(60°)=√3:
√3=210y⟹y=210√3=70√3 m
Step 6: Calculate the width of the river
Now, we can find the width of the river:
AB=x+y=210+70√3
Substituting √3≈1.73:
y=70×1.73=121.1 m
Thus,
AB=210+121.1=331.1 m
Final Answer
The width of the river is 331.1 meters.
---
An aeroplane is flying at a height of 210 m. The angles of depression to two points on opposite banks of the river are 45° and 60°. We need to find the width of the river.
Draw a horizontal line representing the height of the aeroplane (210 m). Mark point P as the position of the aeroplane. Draw two lines from point P down to the ground at angles of depression of 45° and 60° to points A and B on the opposite banks of the river.
Let:
- AD=x (distance from point A directly below the plane to the point on the bank)
- DB=y (distance from point B directly below the plane to the point on the bank)
- The width of the river AB=x+y
For triangle PDA:
- The height PD=210 m
- Angle ∠DAP=45°
tan(45°)=PDAD=210x
Since tan(45°)=1:
1=210x⟹x=210 m
For triangle PDB:
- The height PD=210 m
- Angle ∠DBP=60°
tan(60°)=PDDB=210y
Since tan(60°)=√3:
√3=210y⟹y=210√3=70√3 m
Now, we can find the width of the river:
AB=x+y=210+70√3
Substituting √3≈1.73:
y=70×1.73=121.1 m
Thus,
AB=210+121.1=331.1 m
The width of the river is 331.1 meters.
Topper's Solved these Questions
Similar Questions
An aeroplane is flying at a height of 210 m. Flying at this height at some instant the angles of depression of two points in a line in opposite directions on both the banks of the river are 45∘ and 60∘. Find the width of the river. (Use √3=1.73)
View SolutionAn aeroplane id flying at a height of 300 m above the ground. Fying at this height , theangles of depression from the aeroplane of two points on both banks of a river in opposite directions are 45∘ and 60∘ respectively. Find the width of the river.[use √3=1.732]
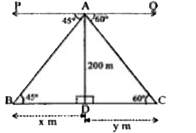
View SolutionAn aeroplane at an altitude of 200 m observes angles of depression of opposite points on the two banks of the river to be 45∘ and 60∘, find the width of the river.
View SolutionAn aeroplane at an altitude of 200 m observes the angles od depression of opposite points on the two banks of a river to be 45∘and60∘. Find the width of the river.
 View Solution
View SolutionAn aeroplane at an altitude of 200 metres observes the angles of depression of opposite points on the two banks of a river to be 450and600. Find the width of the river.
View SolutionThe pilot of an aeroplane at an altitutde of 200 m observes the angle of depression of opposite points on the two banks of a river to be 45∘ and 60∘. Find the width of the river.
View SolutionFrom a point on a bridge across a river, the angles of depression of the banks on opposite sides of the river are 30oand 45o, respectively. If the bridge is at a height of 3 m from the banks, find the width of the river.
View SolutionFrom the top of a 60 m high building, the angles of depression of the top and the bottom of a tower are 45∘ and 60∘ respectively. Find the height of the tower. [Take √3=1.73]
View SolutionAn aeroplane is flying over two houses which are at a distance of 300 m from each other. If at some moment from the aeroplane the angle of depression of two houses on the same side are 45∘ and 60∘, at what height the plane is flying ?
View SolutionA man sitting at a height of 20m on a tall tree on a small island in the middle of a river observes two poles directly opposite to each other on the two banks of the river and in line with the foot of tree. If the angles of depression of the feet of the poles from a point at which the man is sitting on the tree on either side of the river are 600and300 respectively. Find the width of the river.
View Solution

