Find the area of a triangle two sides of which are 18 cm and 10 cm and the perimeter is 42 cm.
To find the area of the triangle using Heron's formula, we will follow these steps:
Step 1: Identify the sides of the triangle
We are given two sides of the triangle:
- Side A = 18 cm
- Side B = 10 cm
We need to find the third side (C) using the perimeter.
Step 2: Calculate the third side
The perimeter of the triangle is given as 42 cm. The formula for the perimeter (P) of a triangle is:
P=A+B+C
Substituting the known values:
42=18+10+C
42=28+C
To find C, we rearrange the equation:
C=42−28
C=14 cm
Step 3: Calculate the semi-perimeter (s)
The semi-perimeter (s) is half of the perimeter:
s=P2=422=21 cm
Step 4: Apply Heron's formula to find the area
Heron's formula for the area (A) of a triangle is given by:
A=√s(s−A)(s−B)(s−C)
Substituting the values we have:
- s=21
- A=18
- B=10
- C=14
Now substituting into the formula:
A=√21(21−18)(21−10)(21−14)
Calculating each term:
- s−A=21−18=3
- s−B=21−10=11
- s−C=21−14=7
Thus, the area becomes:
A=√21×3×11×7
Step 5: Calculate the area
Now we will calculate the product:
A=√21×3×11×7
Calculating step-by-step:
- First calculate 3×7=21
- Next, 21×21=441
- Then, 441×11=4851
Now we take the square root:
A=√4851
Final Result
The area of the triangle is approximately:
A≈69.7 cm2
We are given two sides of the triangle:
- Side A = 18 cm
- Side B = 10 cm
The perimeter of the triangle is given as 42 cm. The formula for the perimeter (P) of a triangle is:
P=A+B+C
Substituting the known values:
42=18+10+C
42=28+C
To find C, we rearrange the equation:
C=42−28
C=14 cm
The semi-perimeter (s) is half of the perimeter:
s=P2=422=21 cm
Heron's formula for the area (A) of a triangle is given by:
A=√s(s−A)(s−B)(s−C)
Substituting the values we have:
- s=21
- A=18
- B=10
- C=14
A=√21(21−18)(21−10)(21−14)
Calculating each term:
- s−A=21−18=3
- s−B=21−10=11
- s−C=21−14=7
A=√21×3×11×7
Now we will calculate the product:
A=√21×3×11×7
Calculating step-by-step:
- First calculate 3×7=21
- Next, 21×21=441
- Then, 441×11=4851
A=√4851
The area of the triangle is approximately:
A≈69.7 cm2
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The area of triangle two sides of which are 18cm and 10cm and its perimeter is 42cm will be-
A14√11cm2B21√11cm2C35√11cm2DNone of these
Find the area of triangle ABC with vertices are A(4,2),B(3,9) and C(10,10)
View SolutionThe sides of a triangle are 11cm, 15cm and 16cm. The altitude to the largest side is
30 √7 cm (b) 15√72 cm (c) 15 √74cm (d) 30 cm
View SolutionThe sides of a triangle are 50cm,78cmand112cm The smallest altitude is
(a) 20cm
(b) 30cm
(c) 40cm
(d) 50cmView SolutionThe figure obtained by joining the mid-points of the adjacent sides of a rectangle of sides 8cm and 6 cm is a rhombus of area 24 cm2 (b) a rectangle of area 24cm2 a square of area 26 cm2 (d) a trapezium of area 14 cm2
View SolutionIn Figure, ABCD is a rectangle with sides AB=10cmandAD=5cm Find the area of EFG
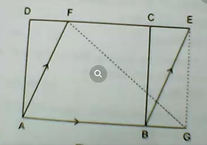 View Solution
View SolutionA paper is in the form of a rectangle ABCD in which AB=20 cm and BC=14 cm. A semicircular portion with BC as diameter is cut off. Find the area of the remaining part.
View SolutionThe area of the figure formed by joining the mid-points of the adjacent sides of a rhombus with diagonals 16cm and 12cm is 28 cm2 (b) 48 cm2 (c) 96 cm2 (d) 24 cm2
View Solution

