A cathod ray tube has a potential different of V between the cathod and anode . The speed of the cahtod rays is given by
To solve the problem regarding the speed of cathode rays in a cathode ray tube with a potential difference V between the cathode and anode, we can follow these steps:
1. Understanding the Setup:
- In a cathode ray tube, electrons are emitted from the cathode and are accelerated towards the anode due to the potential difference V applied between them.
2. Potential Energy Change:
- When the electrons are accelerated through the potential difference V, they gain kinetic energy. The work done on the electrons by the electric field is equal to the change in their potential energy.
- The change in potential energy (ΔPE) for an electron moving through a potential difference V is given by:
ΔPE=qV
- Here, q is the charge of the electron.
3. Kinetic Energy:
- The kinetic energy (KE) gained by the electron as it moves from the cathode to the anode can be expressed as:
KE=12mv2
- Where m is the mass of the electron and v is its speed.
4. Equating Potential Energy and Kinetic Energy:
- Since the work done on the electron converts its potential energy into kinetic energy, we can equate the two:
qV=12mv2
5. Solving for Speed:
- Rearranging the equation to solve for v:
v2=2qVm
- Taking the square root of both sides gives:
v=√2qVm
6. Conclusion:
- The speed of the cathode rays (electrons) is directly proportional to the square root of the potential difference V:
v∝√V
Final Answer:
The speed of the cathode rays is given by:
v=√2qVm
- In a cathode ray tube, electrons are emitted from the cathode and are accelerated towards the anode due to the potential difference V applied between them.
- When the electrons are accelerated through the potential difference V, they gain kinetic energy. The work done on the electrons by the electric field is equal to the change in their potential energy.
- The change in potential energy (ΔPE) for an electron moving through a potential difference V is given by:
ΔPE=qV
- Here, q is the charge of the electron.
- The kinetic energy (KE) gained by the electron as it moves from the cathode to the anode can be expressed as:
KE=12mv2
- Where m is the mass of the electron and v is its speed.
- Since the work done on the electron converts its potential energy into kinetic energy, we can equate the two:
qV=12mv2
- Rearranging the equation to solve for v:
v2=2qVm
- Taking the square root of both sides gives:
v=√2qVm
- The speed of the cathode rays (electrons) is directly proportional to the square root of the potential difference V:
v∝√V
The speed of the cathode rays is given by:
v=√2qVm
Topper's Solved these Questions
- ATOMIC PHYSICSBOOK - NARAYNACHAPTER - ATOMIC PHYSICSEXERCISE - LEVEL-I (C.W)
23 Videos
- ATOMIC PHYSICSBOOK - NARAYNACHAPTER - ATOMIC PHYSICSEXERCISE - LEVEL-II (C.W)
16 Videos
- ATOMIC PHYSICSBOOK - NARAYNACHAPTER - ATOMIC PHYSICSEXERCISE - LEVEL-II (H.W)
14 Videos
- ALTERNATING CURRENTBOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - II(H.W)
13 Videos
- ATOMS BOOK - NARAYNACHAPTER - ATOMS EXERCISE - EXERCISE -4
47 Videos
Similar Questions
Knowledge Check
Cathode rays are
AElectromagnetic wavesBRadiationsCStream of α-particlesDStream of electrons.Cathode rays are
APositive raysBnetural raysChe raysDelectron wavesIf the potential difference between the anode and cathode of the X - ray tube is increases
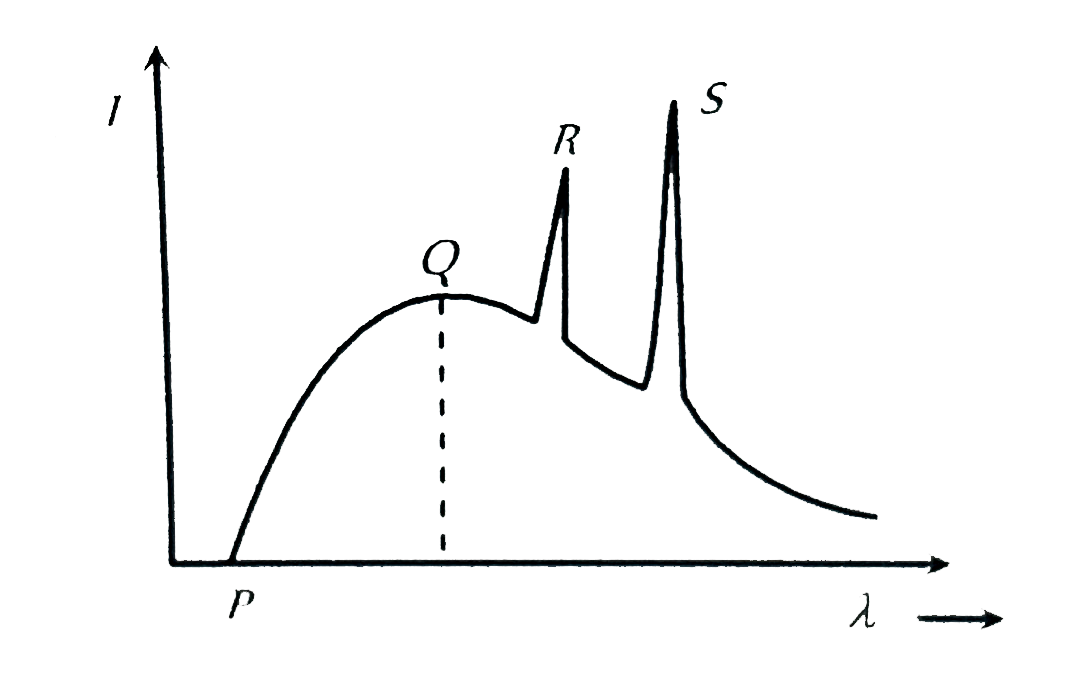
 AThe peaks at R and S would move to shorter wavelengthBThe peaks at R and S would remain at the same wavelengthCThe cut off wavelength at P would decreaseD(b) and (c) both are correct
AThe peaks at R and S would move to shorter wavelengthBThe peaks at R and S would remain at the same wavelengthCThe cut off wavelength at P would decreaseD(b) and (c) both are correct