A hemispherical bowl has inner diameter 11.2cm. Find the volume of milk it can hold.
Inner diameter of hemispherical bowl, d=11.2cm
So, Inner radius, r=11.22=5.6cm
Volume of hemispherical bowl =23πr3
=23×227×5.63
=367.96cm3
Hence, Volume of bowl is 367.96cm3.
Topper's Solved these Questions
- SURFACE AREA AND VOLUME OF A RIGHT CIRCULAR CYLINDER CONEBOOK - RD SHARMACHAPTER - SURFACE AREA AND VOLUME OF A RIGHT CIRCULAR CYLINDER CONEEXERCISE - All Questions
128 Videos
- TABULAR REPRESENTATION OF STATISTICAL DATA BOOK - RD SHARMACHAPTER - TABULAR REPRESENTATION OF STATISTICAL DATA EXERCISE - All Questions
62 Videos
Similar Questions
A hemispherical bowl made of brass has inner diameter 10.5cm. Find the cost of tinplating it on the inside at the rate of Rs. 4 per 100 cm2
View SolutionA hemispherical bowl made of brass has inner diameter 10.5 cm. Find the cost of tin-plating it on the inside at the rate of Rs 32 per 100cm2.
View Solution
Knowledge Check
If a hemispherical dome has an inner radius 21 m then its volume ( in m3) is :
A4910m3B18354m3C19404m3Dnone of the aboveA hemispherical bowl of internal diameter 24 cm contains water. This water is to be filled in cylindrical bottles, each of radius 6 cm and height 8 cm. How many such bottles are required to empty the bowl?
A3B4C5D6
A hemispherical bowl made of brass has inner diameter 10.5 cm. Find the cost of tin-plating it on the inside at the rate of Rs 16 per100\ cm2 .
View SolutionA hemispherical bowl made of brass has inner diameter 10.5 cm. Find the cost of tin-plating it on the inside at the rate of Rs 16 per100cm2.
View SolutionA hemispherical bowl has a radius of 3.5cm. What would be the volume of water it would contain?
View SolutionA thick hemispherical vessel has outer diameter 18 cm and thickness 1 cm. How much water can the vessel hold? (tak π as 3.14)
View SolutionA hollow hemispherical bowl of thickness 1 cm has an inner radius of 6 cm. Find the volume of metal required to make the bowl.
View SolutionIn Figure a decorative block is shown which is made of two solids , a cube and a hemisphere .The base of the block is cube with edge 6 cm and the hemisphere fixed on the top has a diameter of 4.2 cm .Find :
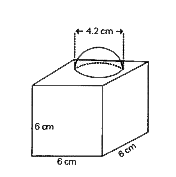
the volume of the block formed
View Solution
