The vapour pressure of a certain liquid is given by the equation:
Log10P=3.54595−313.7T+1.40655log10T where P is the vapour pressure in mm and T= Kelvin Temperature. Determine the molar latent heat of vaporisation as a function of temperature. calculate the its value at 80K.
The correct Answer is:ΔH=1659.9 Cal. At 80K,ΔH=R[313.7×2.303+1.40655T]
dInPdT=ΔHRT2 ...(i)
logP=3.54595−313.7T+1.40655logT
InP=3.54595×2.303−313.7T×2.303+1.40655InT
dInPdT=313.7×2.303T2+1.40655T ...(ii)
Compairing equation (i) & (ii)
ΔH=R[313.7×2.303+1.40655T]
at T=80K
ΔH=1659.9Cal.
Topper's Solved these Questions
- SOLUTIONSBOOK - ALLENCHAPTER - SOLUTIONSEXERCISE - EXERCISE-05 [A]
46 Videos
- SOLUTIONSBOOK - ALLENCHAPTER - SOLUTIONSEXERCISE - EXERCISE -05 [B]
22 Videos
- SOLUTIONSBOOK - ALLENCHAPTER - SOLUTIONSEXERCISE - EXERCISE-04 [A]
17 Videos
- S-BLOCK ELEMENTS BOOK - ALLENCHAPTER - S-BLOCK ELEMENTS EXERCISE - EXERCISE -3
1 Videos
- Some Basic Concepts of Chemistry (Mole concept)BOOK - ALLENCHAPTER - Some Basic Concepts of Chemistry (Mole concept)EXERCISE - All Questions
39 Videos
Similar Questions
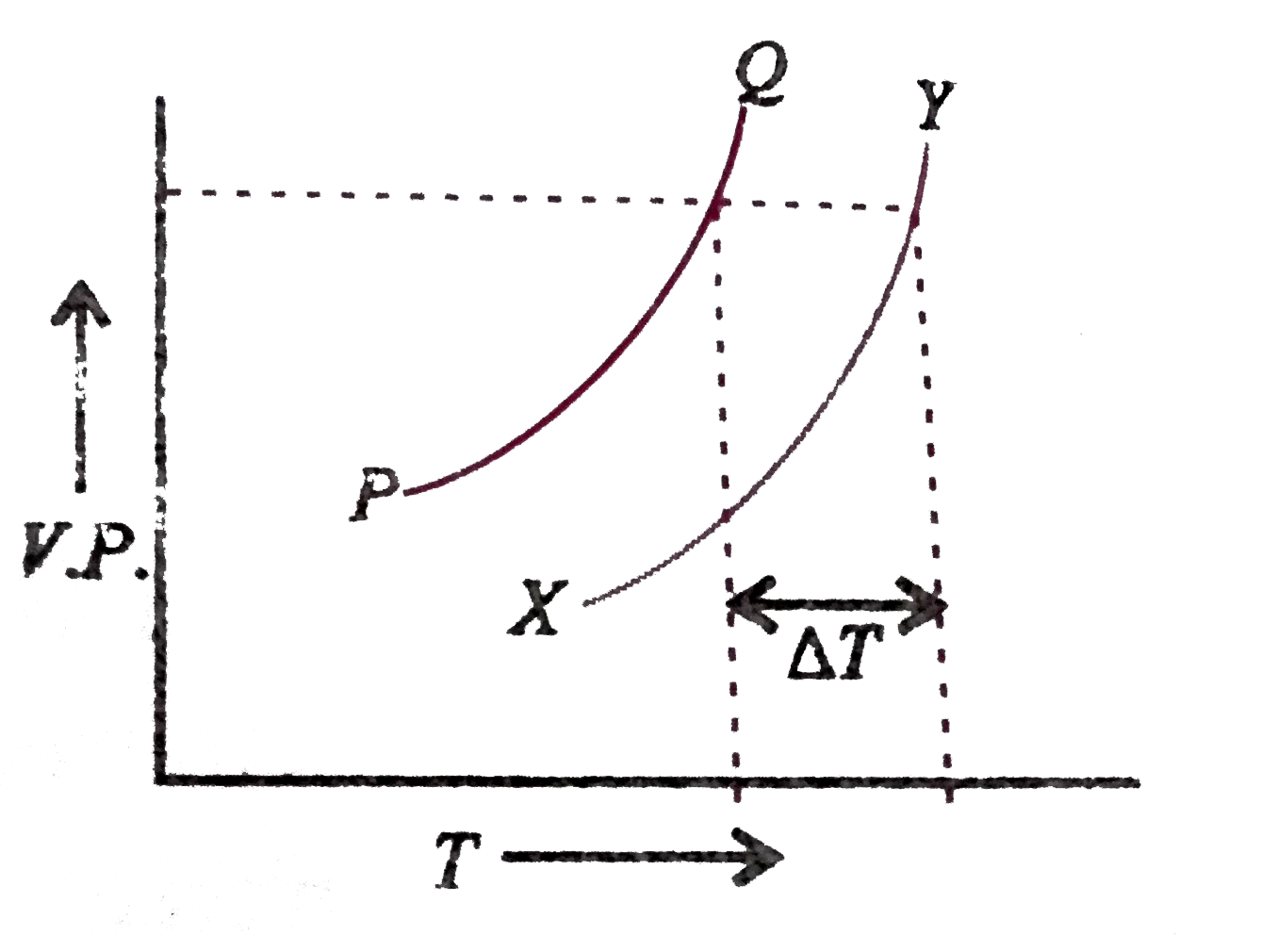
In the graph plotted between vapour pressure (V.P.) and temperature (T),
View SolutionFor an elementary reaction the variation of rate constant (k) with temperature is given by the following equation
log10k=5.4−100T
Where, T is temperature on Kelvin scal and k is in terms of sec−1
Identify the incorrect options.
View Solution
Knowledge Check
The vapour pressure of a liquid varies with temperature as log P=−AT+I. The plot of
Alog P against T will be a straight lineBlog P against 1/T will be straight lineClog P against I will be a parabolaDNone of theseThe vapour pressure of a liquid varies with temperature as log P=−AT+I. The plot of
Alog P against T will be a straight lineBlog P against 1/T will be a straight lineClog P against I will be a parabolaDnone of these.K represents the rate constant of a reaction when log K is p lotted again st 1/T (T=temperature) the graph obtained is a
AcurveBa straight line with a constant positive slopeCa straight line with constant negative slopeDa straight line with no slope
At a given temperature, the vapour pressure in mm of Hg. of a solution of two volatile liquids A and B is given by the equation :
ρ=120−80XB, XB= mole fraction of B.
Vapour pressures of pure A and B at the same temperature are respectively
View SolutionVapour pressure of a solvent is the pressure exterted by vapour when they are in equilibrium with its solvent at that temperature. The vapour pressure of solvent is dependent of nature of solvent, temperature, addition of non-volatile solute as well as nature of solute to dissociate or associate. The vapour pressure of a mixture obtained by mixing two valatile liquids is given by PM=P∘A.XA+P∘B.XB where P∘A and P∘B are vapour pressures of pure components A and B and XA,XB are their mole fractions in mixture. For solute-solvent system, the relatio becomes PM=P∘A.XA where B is non-volatile solute.
If M is mol.wt. of solvent, Kb is molal elevation constant and tb is its boiling point, P∘ is its vapour pressure ta temperature T and PS is vapour pressure of non-volatile solute in it at TK, then:
View SolutionThe total vapour pressure of equimolar solution of benzene and toluene is given by PT (in mm Hg) =600−400Xtoluene then:
View SolutionVapour pressure of a solvent is the pressure exterted by vapour when they are in equilibrium with its solvent at that temperature. The vapour pressure of solvent is dependent of nature of solvent, temperature, addition of non-volatile solute as well as nature of solute to dissociate or associate. The vapour pressure of a mixture obtained by mixing two valatile liquids is given by PM=P∘A.XA+P∘B.XB where P∘A and P∘B are vapour pressures of pure components A and B and XA,XB are their mole fractions in mixture. For solute-solvent system, the relatio becomes PM=P∘A.XA where B is non-volatile solute.
A mixture of two volatile liquids A and B 1 and 3 moels respectively has a V.P of 300mm at 27∘C. IF one mole of A is further added to this solution, the vapour pressure becomes 290mm at 27∘C. The vapour pressure of A is:
View SolutionVapour pressure of a solvent is the pressure exterted by vapour when they are in equilibrium with its solvent at that temperature. The vapour pressure of solvent is dependent of nature of solvent, temperature, addition of non-volatile solute as well as nature of solute to dissociate or associate. The vapour pressure of a mixture obtained by mixing two valatile liquids is given by PM=P∘A.XA+P∘B.XB where P∘A and P∘B are vapour pressures of pure components A and B and XA,XB are their mole fractions in mixture. For solute-solvent system, the relatio becomes PM=P∘A.XA where B is non-volatile solute.
The vapour pressure of benzene and its solution with a non-electrolyte are 640 and 600mm respectively. The molality of solution is:
View Solution
