A flywheel can rotate in order to store kinetic energy. The flywheel is a uniform disc made of a material with a density ρ and tensile strength σ (measured in Pascals), a radiys r, and a thickness h. The flywheel is rotating at the maximum possible angular velocity so that it does not break. Which of the following expression correctly gives the maximum kinetic energy kilogram that can be stored in the flywheel? Assume that α is a dimensionless constant.
A
α√ρσ/r
B
αh√ρσ/r
C
σα√(h/r)ρ
D
ασ/ρ
Video Solution
Text Solution
The correct Answer is:D
Dimensions of kinetic energy per unit mass =L2T−2
[ασρ]=ML−1T−2ML−3=L2T−2
|
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
The rotational kinetic energy is -
Amω2B12Iα2C12Iω2D12mv2 - Question 2 - Select One
The mass of a flywheel is concentrated at its rim
Ato obtain a strong wheelBto decrease the moment of inertiaCto increase the moment of inertiaDto obtain the stable equilibrium - Question 3 - Select One
Rotational kinetic energy is equal to
A1w2BI2w2C32Iw2D12Iw2
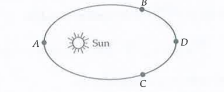
The maximum rotational kinetic energy of a planet moving around the sun is at position
 View Solution
View SolutionWhat does the maximum kinetic energy (or the maximum speed) of a photoelectron depend on ?
View SolutionWhen a disc rotates with uniform angular velocity , which of the following is not true ?
View SolutionWhen a disc rotates with uniform angular velocity, which of the following is not true ?
View SolutionWhen a disc rotates with uniform angular velocity, which of the following is not true ?
View Solution

