Match the entries of column-I with those of column - II
Column-IColumn-IIt∈R such that there is at least one z satisfying |Z|=3,|z−{t−(1+i)−i}|≤3and|z+2t−(t+1)i|>3(p) 6 (B) Solve for x : (1+i)x−2i3+i+(2−3i)y+i3−i=i(p) 0(C) The integer n for which (1+i1−i)n is real(r) 3(D) The greatest and least absolutte value of z + 1 , where |z+4|≤3are(s) 4(t) 8
The correct Answer is:A(r,s),B(r ) , C(p,q,s,t) , D( p,q)
Topper's Solved these Questions
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBook:AAKASH INSTITUTEChapter:COMPLEX NUMBERS AND QUADRATIC EQUATIONSExercise:section-G( Integer Answer type Questions)
Explore 8 Videos
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBook:AAKASH INSTITUTEChapter:COMPLEX NUMBERS AND QUADRATIC EQUATIONSExercise:section-H ( Multiple True-False Type Questions
Explore 5 Videos
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBook:AAKASH INSTITUTEChapter:COMPLEX NUMBERS AND QUADRATIC EQUATIONSExercise:Assertion -Reason Type Questions
Explore 19 Videos
- BINOMIAL THEOREMBook:AAKASH INSTITUTEChapter:BINOMIAL THEOREMExercise:Assignment (section-J) Objective type question (Aakash Challengers Questions)
Explore 4 Videos
- CONIC SECTIONSBook:AAKASH INSTITUTEChapter:CONIC SECTIONSExercise:SECTION - J ( Aakash Challengers Questions )
Explore 14 Videos
Similar Questions
If z=(1+i)(1+2i)(1+3i)(1−i)(2−i)(3−i) then the principal argument of z
View SolutionMatch the following :
Column - IColumn - II(A) The smallest positive integer for which(1+i)n=(1−i)n is (p) 1(B) If 3√a+ib=x+iyandby−ax=k(x2+y2) then k is equal to (p) -3 (C) If x=1+i√2, then the value of 1+x2+x4+x6+x8+x10+....+x2004+x2006+x2008(r) 2(D) If the minimum value of |z+1+i|+|z−1−i|+|2−z|+|3−z| is k then (k-8) equals (s)4
View SolutionMatch the following :
Column - IColumn - II(A) The smallest positive integer for which(1+i)n=(1−i)n is (p) 1(B) If 3√a+ib=x+iyandby−ax=k(x2+y2) then k is equal to (p) -3 (C) If x=1+i√2, then the value of 1+x2+x4+x6+x8+x10+....+x2004+x2006+x2008(r) 2(D) If the minimum value of |z+1+i|+|z−1−i|+|2−z|+|3−z| is k then (k-8) equals (s)4
View SolutionIf the complex number z is to satisfy |z|=3,|z−{a(1+i)−i}|≤3 and |z+2a−(a+1)i|>3, where i=√−1 simultaneously for atleast one z, then find all a∈R.
View SolutionThe complex number z satisfying |z+¯z|+|z−¯z|=2and|iz−1|+|z−i|=2 is/are A)i B) −i C)1i D)1i3
View SolutionColumn-I shows a-t graph and column-II shows v-t graph. Mathc the column-I and column-II.
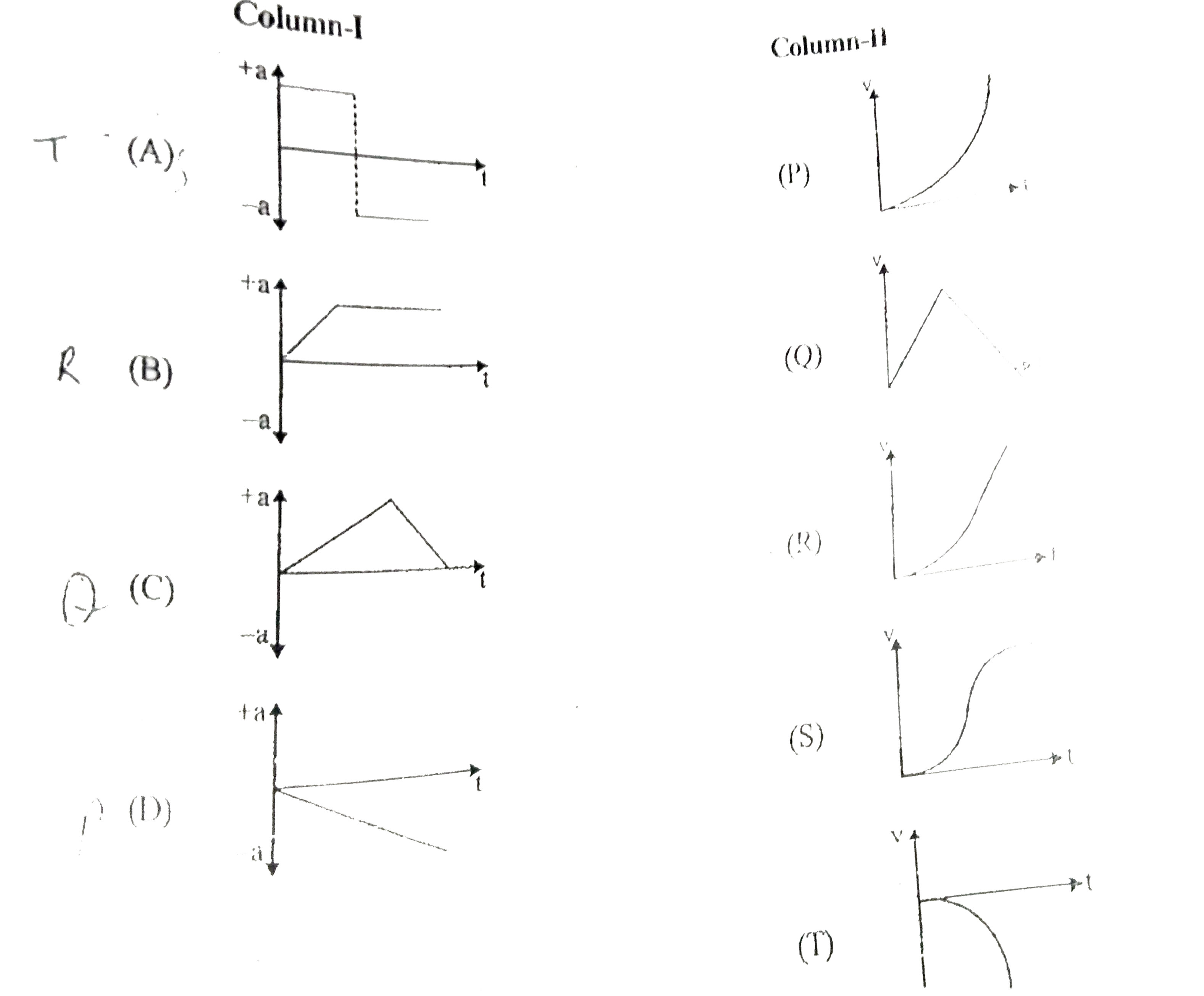
View SolutionFind the values of x and y from the following : (i) (3x−7)+2iy=−5y+(5+x)i (ii) 2xi+12=3y−6i (iii)z=x+iyandi(z+2)+1=0 (iv)(1+i)x−2i3+i+(2−3i)y+i3−i=i (v) (3x−2iy)(2+i)2=10(1+i)
View SolutionFind the values of x and y from the following : (i) (3x−7)+2iy=−5y+(5+x)i (ii) 2xi+12=3y−6i (iii)z=x+iyandi(z+2)+1=0 (iv)(1+i)x−2i3+i+(2−3i)y+i3−i=i (v) (3x−2iy)(2+i)2=10(1+i)
View SolutionIf z1=1+i,z2=−2+3i and z3=ai3, where i2=−1 are collinear, then the value of a is
View SolutionMatch the Column-I with Column-II :

Here, t1/2 = half life
t2/3=3/4 life
k= rate constant
View Solution
