Statement: For any △ A B C , the expression (b+c-a)(c+a-b)(a+b-c)-abc is negative
A
TTT
B
FTT
C
TFT
D
TTF
Text Solution
The correct Answer is:A
|
Topper's Solved these Questions
- TRIGNOMETRIC FUNCTIONS BOOK - AAKASH INSTITUTECHAPTER - TRIGNOMETRIC FUNCTIONS EXERCISE - Section I (subjective Type questions)
35 Videos
- TRIGNOMETRIC FUNCTIONS BOOK - AAKASH INSTITUTECHAPTER - TRIGNOMETRIC FUNCTIONS EXERCISE - Section - J (Akash Challengers Question)
15 Videos
- TRIGNOMETRIC FUNCTIONS BOOK - AAKASH INSTITUTECHAPTER - TRIGNOMETRIC FUNCTIONS EXERCISE - Section -G (Integer Answer type questions)
18 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - AAKASH INSTITUTECHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - ASSIGNMENT SECTION - J
10 Videos
- VECTOR ALGEBRABOOK - AAKASH INSTITUTECHAPTER - VECTOR ALGEBRAEXERCISE - SECTION-J (Aakash Challengers Questions)
5 Videos
Similar Questions
Knowledge Check
If a, b, c are all positive integers, then the minimum value of the expression (a+b+c)(b2+b+1)(c2+c+1)abc is :
A3B9C27D1For which of the following graphs of the quadratic expression y=ax2+bx+c, the product a.b.c is negative.
A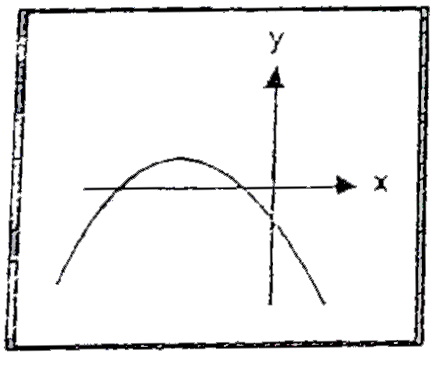 B
B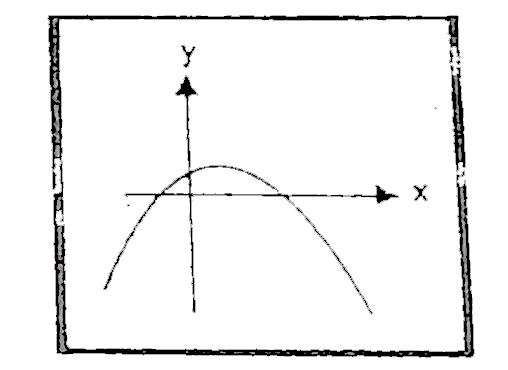 C
C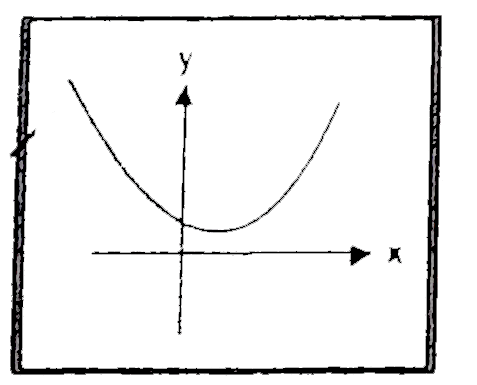 D
D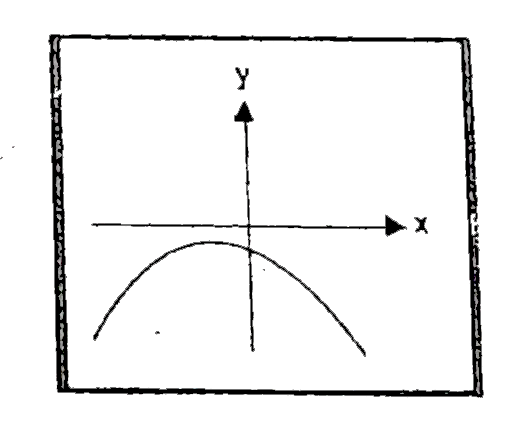
In any Delta ABC, (a+b+c)(b+c−a)(c+a−b)(a+b−c)4b2c2 equals
Asin2BBcos2ACcos2BDsin2A
If a, b and c are distinct positive numbers, then the expression (a+b−c)(b+c−a)(c+a−b)−abc is:
View SolutionIf a,b,c are different positive real numbers such that b+c−a,c+a−b and a+b−c are positive, then (b+c−a)(c+a−b)(a+b−c)−abc is a. positive b. negative c. non-positive d. non-negative
View SolutionIf a, b and c are distinct positive numbers, then the expression (a+b−c)(b+c−a)(c+a−b)−abc is:
View SolutionIn any ΔABC, prove that sinBsinC=c−acosBb−acosC
View SolutionIn ΔABC,(a+b−c)(b+c−a)(c+a−b)−abc is always
View Solution
