A point charge +q is held at a distance R from the centre of an uncharged spherical conductor of radius a(a<R). Calculate the potential of the conductor in the presence of q.
Remember that the superposition principle is valid even in the presence of conducors.

'q' alone would produce an electric field at all points within the volume of the conductor . The conductor must some how cancel the field due to q at all points interior to it. But there is no charge given to the conductor. The conductor solves this problem in a remarkable way. It pulls out positive and negative charges of equal magnitude from its volume and spreads them on its surface in such a manner that at every point in its interior the field due to q is cancelled by the field due to the surface charges. The potential ϕ within the sphere is uniform, because it is a conducting sphere. If we can find the value of ϕ at any point in the sphere, our problem is solved . Of all points within the sphere, there is one point which is best suited from the calculation of ϕ . This point is the centre C of the sphere . Every element of the surface is at the same distance 'a' from the centre of the sphere and , therefore, the potential at C due to all the surface charges ( irrespective of their distribution ) is
∮σ.ds4πε0a=14πε0a∮σ.ds=0
Because the total charge on the surface of the sphere is zero in this problem. So only contribution to potential at C comes from q now, and this is equal to q4πε0R
Thus the potential of the sphere is q4πε0R
Note that the above discussion can be extended to the case where the net charge on the spherical has any given value Q . Several point charges outside the conductor can also be taken into account.
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTECHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - TRY YOURSELF110videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - AAKASH INSTITUTECHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - ASSIGNMENT (SECTION-A) Objective Type Questions (Only one answer)47videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions25videos
ELECTROMAGNETIC WAVES
- BOOK - AAKASH INSTITUTECHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)16videos
GRAVITATION
Similar Questions
A point charge Q is situated (outside) at a distance r from the centre of an uncharged conducting solid sphere of radius R. The potential due to induced charges (on the conductor) at a point B inside the conductor whose distance form the point charge is d is ______
View SolutionA charge q is placed at a distance of 2R from the centre of a spherical conducting shell of radius R. What is electrical potential, due to charges induced on the shell, at a point which is farthest from the point charge ?
View Solution
Knowledge Check
- Question 1 - Select One
A point charge q is placed at a distance of r from the centre O of an uncharged spherical shell of inner radius R and outer radius 2R. The electric potential at the centre of the shell will be
 Aq4πε0(1r−12R)Bq4πε0rCq4πε0(1r+12R)DNone of these
Aq4πε0(1r−12R)Bq4πε0rCq4πε0(1r+12R)DNone of these - Question 2 - Select One
A point charge q is placed at a distance of r from cebtre of an uncharged conducting sphere of rad R(<r). The potential at any point on the sphere is
AzeroB14πε0qrC14πε0qRr2D14πε0qr2R - Question 3 - Select One

A point charge q'q is placed at distance 'a' from the centre of an uncharged thin spherical conducting shell of radius R=2a. A point 'P' is located at a distance 4a from the centre of the conducting shell as shown. The electric potential due to induced charge on the inner surface of the conducting shell at point 'P' isAkq5aB−kq5aCkq4aD−kq4a
[" A point of charged "q" is placed at a distance "2r" from the "],[" centre "0" of a conducting uncharged sphere of radius "r" ."],[" What is potential of induced charges at point "P" (lying in a "P(2....)],[" line joining the point charge and centre of sphere).Given "],[q=10nC" and "r=5m]
View SolutionA point charge +Q is placed at the centre 0 of an uncharged hollow spherical conductor of inner radius 'a' and outer radius 'b'.Find the following.
The magnitude and sign of the charge induced on the inner and outer surface of the conducting shell.View SolutionLet at any instant point charge is at x distance from centre
Q. Net charge on surface of conductor at this instantView SolutionThe magnitude of an electric intensity at a point which is at a distance 'r' from the centre of a charged spherical conductor of radius 'R' in terms of the surface charge density 'σ' is given by 'E' where
View Solution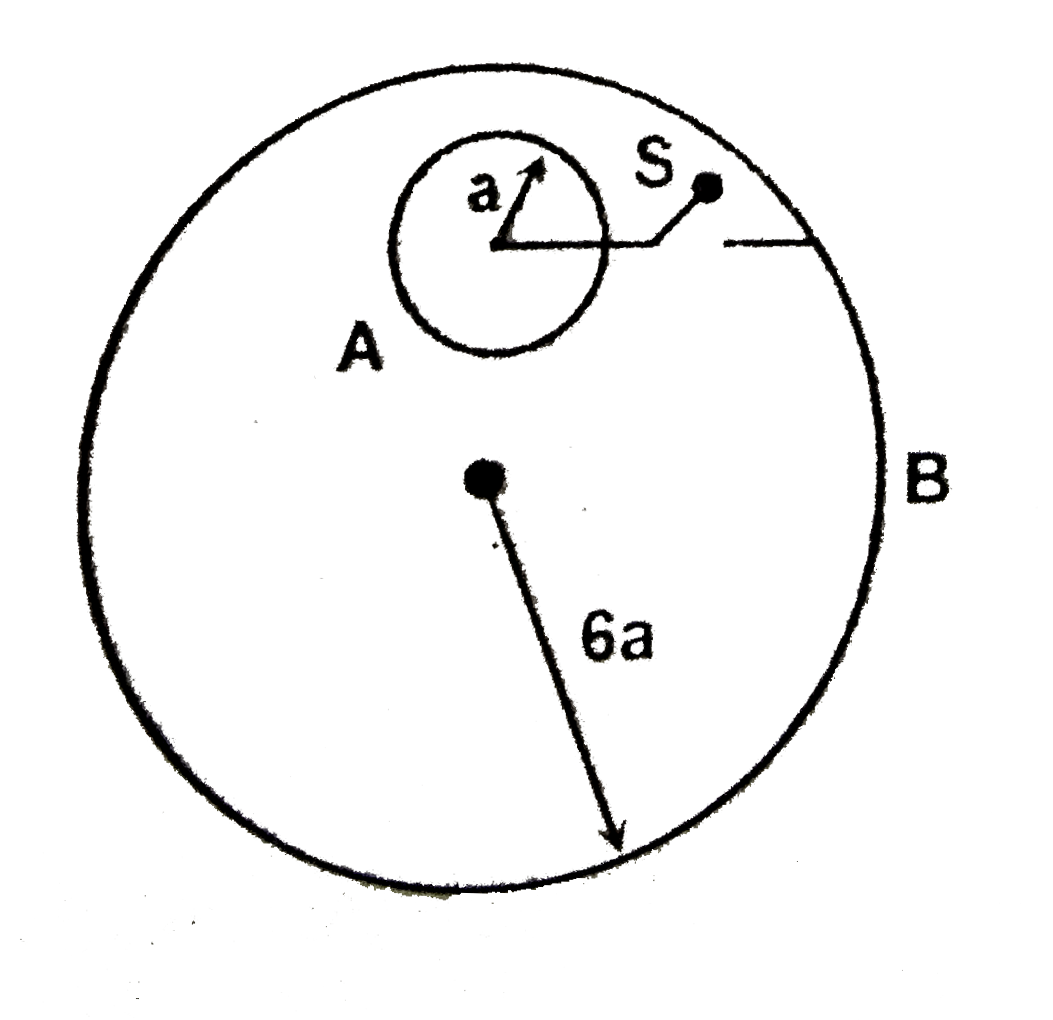
A small spherical conductor 'A' of radias 'a' is initially charged to a potential 'V' and then placed inside an uncharged spherical conducting shell 'B' of radius 6a as shown. The potential of the spherical conductor 'A' after closing the switch S isView Solution

