In a triangle ABC,vertex angles A,B,C and side BC are given .The area of ΔABC is
s(s−a)(s−b)(s−c)2
b2sinCsinAsinB
ab sin C
12a2sinBsinCsinA
The correct Answer is:D
Step by step video, text & image solution for In a triangle ABC,vertex angles A,B,C and side BC are given .The area of DeltaABC is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMBOOK - OBJECTIVE RD SHARMACHAPTER - PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMEXERCISE - Chapter Test
55 Videos
- PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMBOOK - OBJECTIVE RD SHARMACHAPTER - PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMEXERCISE - Section II - Assertion Reason Type
13 Videos
- PLANE AND STRAIGHT LINE IN SPACE BOOK - OBJECTIVE RD SHARMACHAPTER - PLANE AND STRAIGHT LINE IN SPACE EXERCISE - Chapter Test
31 Videos
- REAL FUNCTIONS BOOK - OBJECTIVE RD SHARMACHAPTER - REAL FUNCTIONS EXERCISE - Chapter Test
60 Videos
Similar Questions
In a triangle ABC, the sides b,c are given. If there is an error δA in measuring angle A.Then side a is error δa in the side a is
View Solution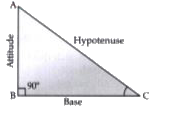
ΔABC is a right triangle, right angled at B. ∠C is a given acute angle. So side BC is base, a side AB is altitude and side AC is hypotenuse for given acute angle C.
Find the ratio of sin C.
View Solution
Knowledge Check
In a triangle ABC,vertex angles A,B,C and side BC are given .The area of ΔABC is
As(s−a)(s−b)(s−c)2Bb2sinCsinAsinBCab sin CD12a2sinBsinCsinAIn △ABC right angled at B, if the two sides AB and BC are in the ratio 1 : 3, evaluate the value of sin C.
A√103B3√10C13D1√10Let triangle ABC is right triangle right angled at C such that A<B and r=8,R=41 .
Q. Area of ΔABC is :A720B1440C360D480
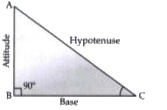
△ABC is a right triangle, right angled at B. ∠C is a given acute angle. So side BC is base, a side AB is altitude and side AC is hypotenuse for given acute angle C. The ratio BCAC is equal to ________
View Solution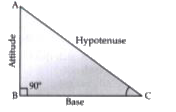
ΔABC is a right triangle, right angled at B. ∠C is a given acute angle. So side BC is base, a side AB is altitude and side AC is hypotenuse for given acute angle C.
Find the ratio of secant of ∠C
View SolutionLet triangle ABC is right triangle right angled at C such that A<B and r=8,R=41 .
Q. Area of ΔABC is :
View SolutionThe triangle is formed by joining the mid-points of the sides AB,BC and CA of △ABCand the area of △PQR is 6cm2, then the area of △ABC is :
View SolutionABC is a right angled triangle. B being the right angle. Mid-points of BC and AC are respectively B' and A'. Area of ΔA'B'C' is
View Solution
