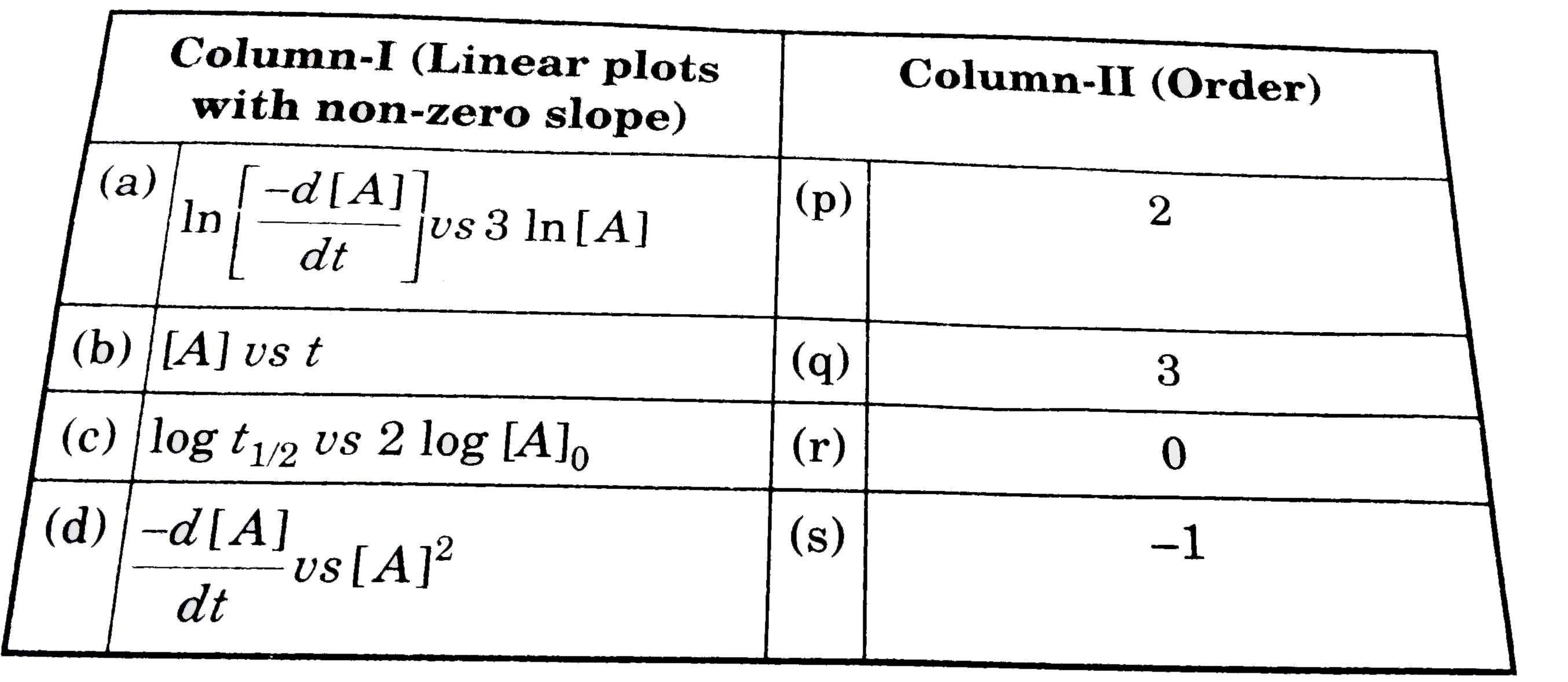A substance 'A' undergoes a reaction such that its concentration at anytime t (in sec.) can be represented by the equation [A]4t=1t+1 where 't' is time in sec and [At] represents molar concentration. Calculate rate of disappearance of 'A' at concentration of 2M.
The correct Answer is:8
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - GRB PUBLICATIONCHAPTER - CHEMICAL KINETICSEXERCISE - match the column type
13 Videos
- CHEMICAL BONDING-IBOOK - GRB PUBLICATIONCHAPTER - CHEMICAL BONDING-IEXERCISE - Subjective Type
120 Videos
- COORDINATION COMPOUNDSBOOK - GRB PUBLICATIONCHAPTER - COORDINATION COMPOUNDSEXERCISE - SBJECTIVE TYPE
98 Videos
Similar Questions
Knowledge Check
In a 1st order reaction, reactant concentration C varies with time t as:
A1/C increases linearly with tBlog C decreases linearly with tCC decreases with 1/tDlog C decreases with 1/tIn a 1st order reaction, reactant concentration C varies with time t as:
A1/C increases linearly with tBlog C decreases linearly with tCC decreases with 1/tDlog C decreases with 1/tIn a 1st order reaction, reactant concentration C varies with times t as:
A1C increase linearly with tBlogC decrease linearly with tCC decrease with 1tDlogC decrease with 1t
In the II order reaction: 2A→A2 .The rate of formation of A2 is 10−5M sec−1 at 0.01M concentration of A .Calculate the rate constant and the rate of disappearance of 'A'
View SolutionIn the II order reaction 2A→A2 .The rate of formation of A2 is 10−5M sec−1 at 0.01M concentration of A . Calculate the rate constant in the rate of disappearance of 'A:
View SolutionA metallic disc is being heated. Its area (in m2) at any time t (in sec) is given by A=5t2+4t. Calculate the rate of increase in area at t=3sec.
View SolutionA metallic disc is being heated. Its area (in m2) at any time t (in sec) is given by A=5t2+4t. Calculate the rate of increase in area at t=3sec.
View SolutionA simple harmonic motion having an amplitude A abd time period T is represented by the equation :y=5sinπ(t−4)m. Then the values of A ("in m")andT("in sec") are:
View Solution