Rain is falling with a velocity $(- 4 ˆ i + 8 ˆ j - 10 ˆ k)$ . A person is moving with a velocity of $(6 ˆ i + 8 ˆ j)$ on the ground.
The velocity of man $w . r . t .$ rain is $:$

A

$- 6 ˆ i - 8 ˆ j$

B

$4 ˆ i - 8 ˆ j + 10 ˆ k$

C

$- 10 ˆ i - 10 ˆ k$

D

$10 ˆ i + 10 ˆ k$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

${\to V}_{r m} = {\to V}_{r} - {\to V}_{m} = (- 10 ˆ i - 10 ˆ k)$
Ans. $(- 10 ˆ i - 10 ˆ k)$ rain appears to come $45^{\circ}$ with $ˆ j$
${\to V}_{m r} = - {\to V}_{r m} = 10 ˆ i + 10 ˆ k$
Maximum power $= F_{max} \times V$
Maximum force applied by camel is during the accelerated motion.

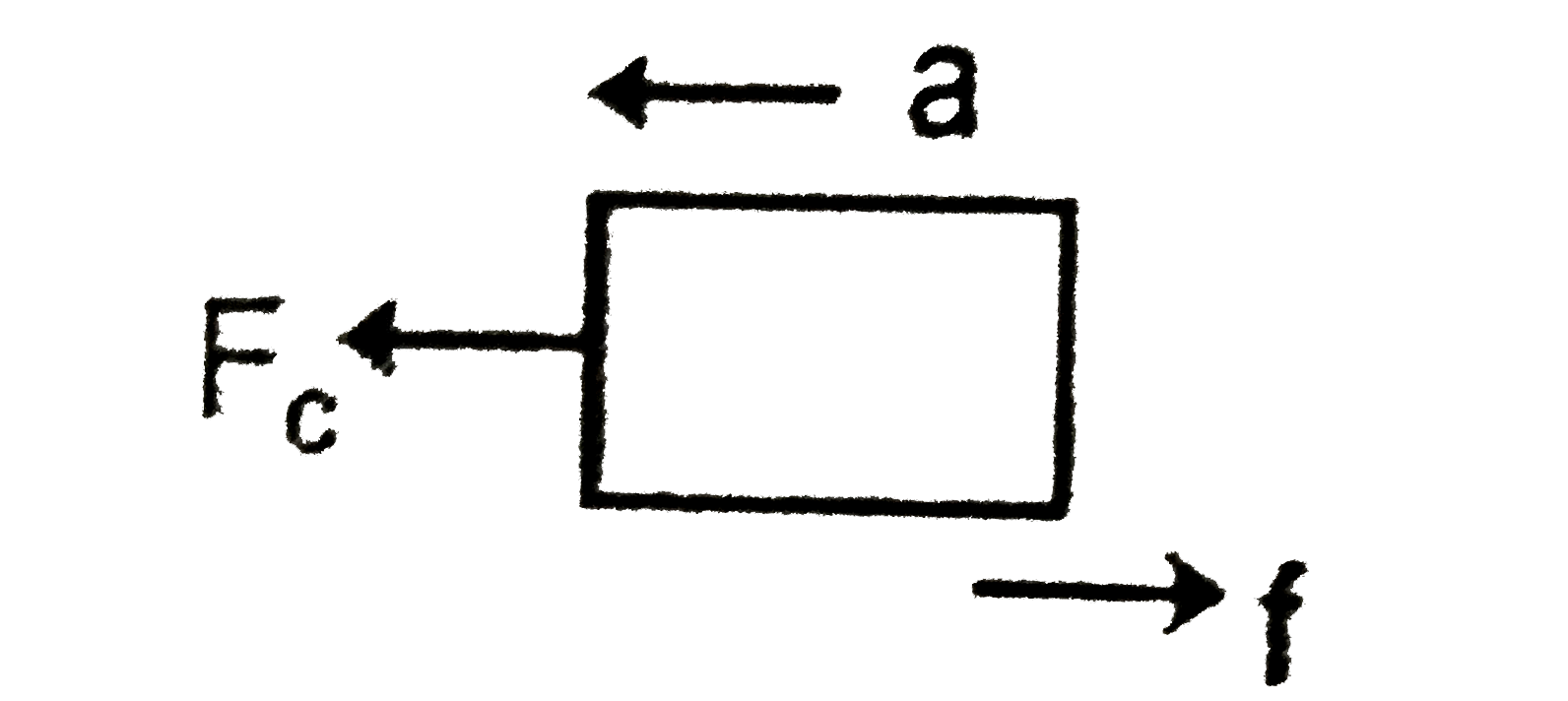
We have $V^{2} - U^{2} = 2 a s$
$25 = 0^{2} + 2 . a .50$
$a = 0.25 m / s^{2},$ for accelerated motion
$∴ F_{C} - f = m a$
$∴ F_{C} = μ m g + m a = 0.1 \times 1000 \times 10 + 1000 \times 2.5$
$= 1000 + 250 = 1250 N$
This is the critical point just before the point where it attains the maximum velocity of almost $5 m / s$
Hence maximum power at this point is $= 1250 \times 5 = 6250 J / s$ .

|

Updated on:21/07/2023

Class 11 PHYSICS QUESTION BANK

Similar Questions

Knowledge Check

Question 1 - Select One
Rain is falling with a velocity $(- 4 ˆ i + 8 ˆ j - 10 ˆ k)$ . A person is moving with a velocity of $(6 ˆ i + 8 ˆ j)$ on the ground.
The speed with the rain drops hit the person is $:$
A $10 m / s$
B $10 \sqrt{2} m / s$
C $\sqrt{180} m / s$
D $\sqrt{360} m / s$
Question 2 - Select One
A boat is moving with a velocity $3 ˆ i + 4 ˆ j$ with respect to ground. The water in the river is moving with a velocity $- 3 ˆ i - 4 ˆ j$ with respect to ground. The relative velocity of the boat with respect to water is.
A $8 ˆ j$
B $- 6 ˆ i - 8 ˆ j$
C $6 ˆ i + 8 ˆ j$
D $5 \sqrt{2})$
Question 3 - Select One
A plane mirror is moving with velocity $4 (ˆ i) + 5 (ˆ j) + 8 (ˆ k)$ . A point object in front of the mirror moves with a velocity $3 (ˆ i) + 4 (ˆ j) + 5 (ˆ k)$ . Here, $ˆ k$ is along the normal to the plane mirror and facing towards the object. The velocity of the image is
A $- 3 (ˆ i) - 4 (ˆ j) + 5 (ˆ k)$
B $3 (ˆ i) + 4 (ˆ j) + 11 (ˆ k)$
C $- 4 (ˆ i) + 5 (ˆ j) + 11 (ˆ k)$
D $7 (ˆ i) + 9 (ˆ j) + 3 (ˆ k)$

Recommended Questions

Home
Ask Doubt
Profile