A body covered a distance of Lm along a curved path of a quarter circle. The ratio of distance to displacements.
π2√2
2√2π
π√2
√2π
The correct Answer is:A
Displacement d1=√2r
Distance from A to Bd2=(πr2)
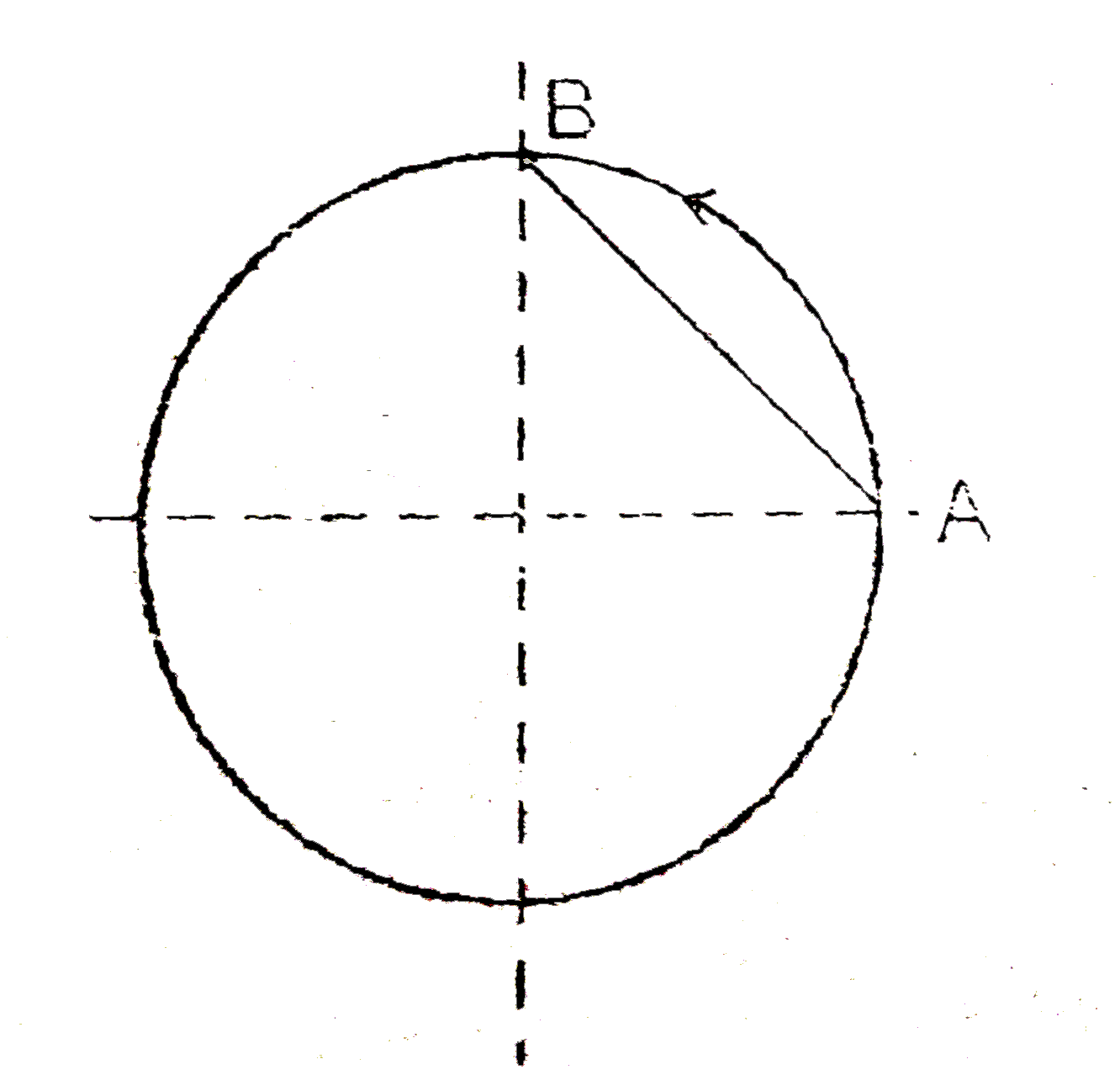
d2d1=πr2√2r=(π2√2).
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.49videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.520videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.210videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)21videos
CURRENT ELECTRICITY
- BOOK - RESONANCECHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 327videos
ELECTRO MAGNETIC WAVES
Similar Questions
Knowledge Check
- Question 1 - Select One
A body moves along a curved path of a quarter circle. Calculate the ratio of distance to displacement :
A11:7B7:11C11:√2×7D7:11√2 - Question 2 - Select One
A body covers a distance of L along a semicircular path. Then the ratio of distance to displacement is
A2LπBLπCπ2LDπ2 - Question 3 - Select One
A body moves with a constant speed along a curved path its acceleration :
Ais zeroBis parallel to its velocityCcan make any arbitrary angle with its velocityDis perpendicular to its velocity
A body travels a distance of 15m from A to B and then moves a distance of 20m at right angles to AB. Calculate the total distance travelled and the displacement.
View SolutionA body is moving along a circular path of radius R. What will be the distance travelled and displacement of the body when it completes half a revolution ?
View SolutionIf the distance covered by a body moving in a circular path of radius (r ) is equal to 2πr, find its displacement.
View SolutionIf the distance covered by a body moving in a circular path of radius (r ) is equal to 2πr, find its displacement.
View SolutionA body starting from rest covers a distance of 9 m in the fifth second. The acceleration of the body is:
View Solution

