The radius of the orbit of an electron in a Hydrogen - like atom is 4.5s0 where a0 is the bohr radius its orbital angular momentum is 3h2π it is given that is is plank constant and R is rydberg constant .The possible wavelength (s) , when the atom de- excite , is (are)
A
932R
B
916R
C
95R
D
93R
Video Solution
Text Solution
The correct Answer is:A, C
Rn=4.5a0
L=mvr=3h2π[asn=3,x=2]
1λ=Rz2⎛⎝1n2f−1n21⎞⎠
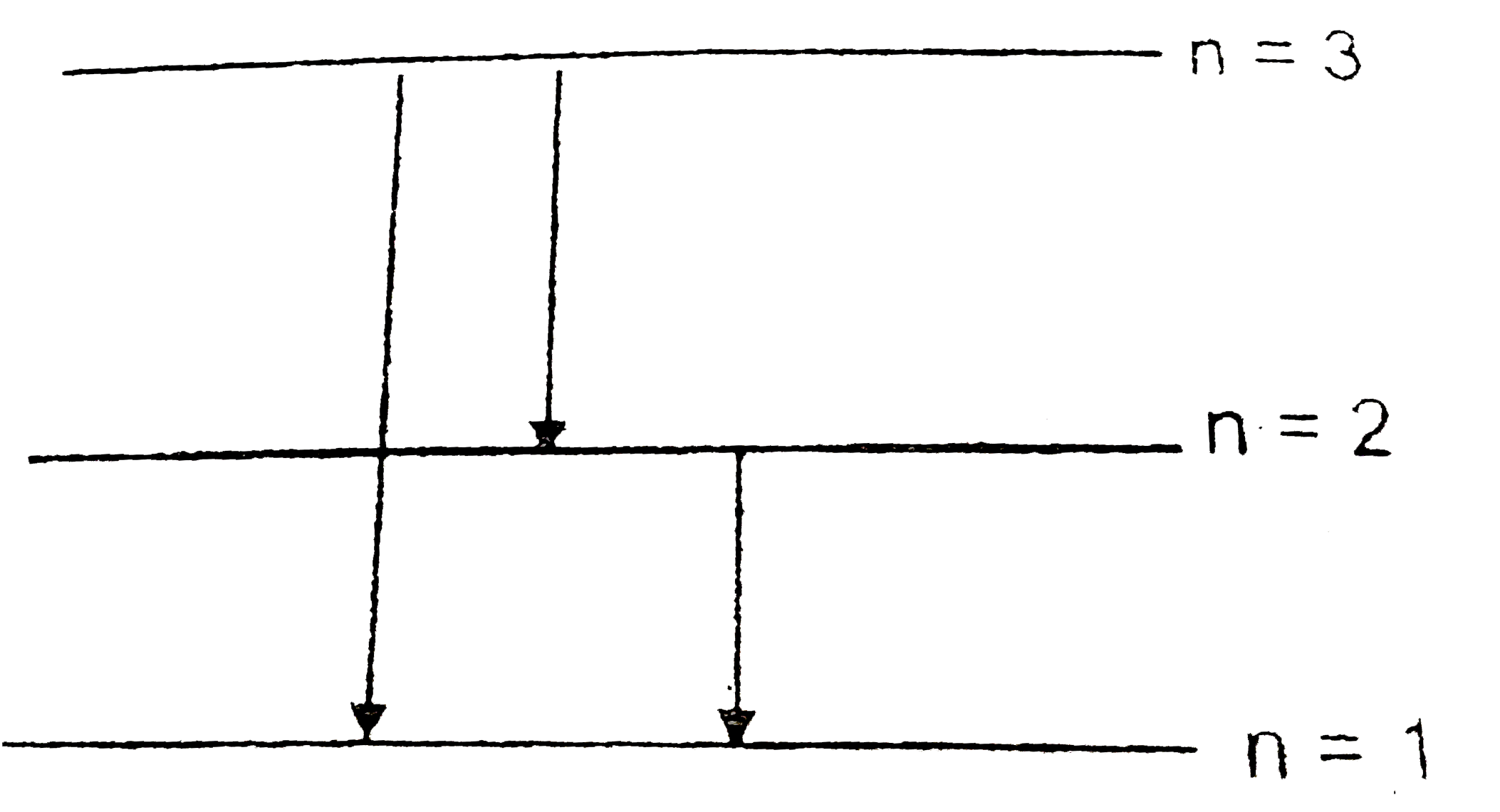
1λ3→1R4[11−19]=4R89⇒λ3→1=932R
1λ2→1=R4[11−14]=344R⇒λ2→1=13R
1λ3→2=R4[14−19]=5364R⇒λ3→2=95R.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.2120videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.229videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.1920videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)21videos
CURRENT ELECTRICITY
- BOOK - RESONANCECHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 327videos
ELECTRO MAGNETIC WAVES
Similar Questions
Knowledge Check
- Question 1 - Select One or More
The radius of the orbit of an electron in a Hydrogen - like atom is 4.5s0 where s0 is the bohr radius its orbital angular momentum is 3b2π it is given that is is plank constant and R is rabdery constant .The possible wavelength (s) , when the atom de- exciter , is (are)
A932RB916RC95RD43R - Question 2 - Select One or More
The radius of the orbit of an electron in a Hydrogen-like atom is 4.5a0, where a0 is the Bohr radius. Its orbital angular momemtum is 3h2π. It is given that h is Plank constant and R si Rydberg constant. The possible wavelength (s), when the atom-de-excites. is (are):
A932RB916RC95RD43R - Question 3 - Select One
The radius of second Bohr’s orbit of Hydrogen atom is:
A0.053 nmB0.0534nmC0.053×4nmD0.053×20nm
The radius of 2nd Bohr.s orbit of hydrogen atom is :
View SolutionThe radius of 2nd Bohr orbit of hydrogen atom is :
View SolutionThe velocity of electrons in the 3rd excited state of a hydrogen atom is [a0 is Bohr radius ]:
View SolutionThe kinetic energy of an electron in the second Bohr orbit of a hydrogen atom is [a0 is Bohr radius] :
View SolutionThe radius of the second orbit of an electron in hydrogen atom is 2.116A. The de Broglie wavelength associated with this electron in this orbit would be
View Solution

