Two light strings, each of length l are fixed at points A andB on a fixed horizontal and xy A small are making angle 45∘ with the bob if the bob is displaced normal to the plane of the string and released then period of the resulting small oscillation will be
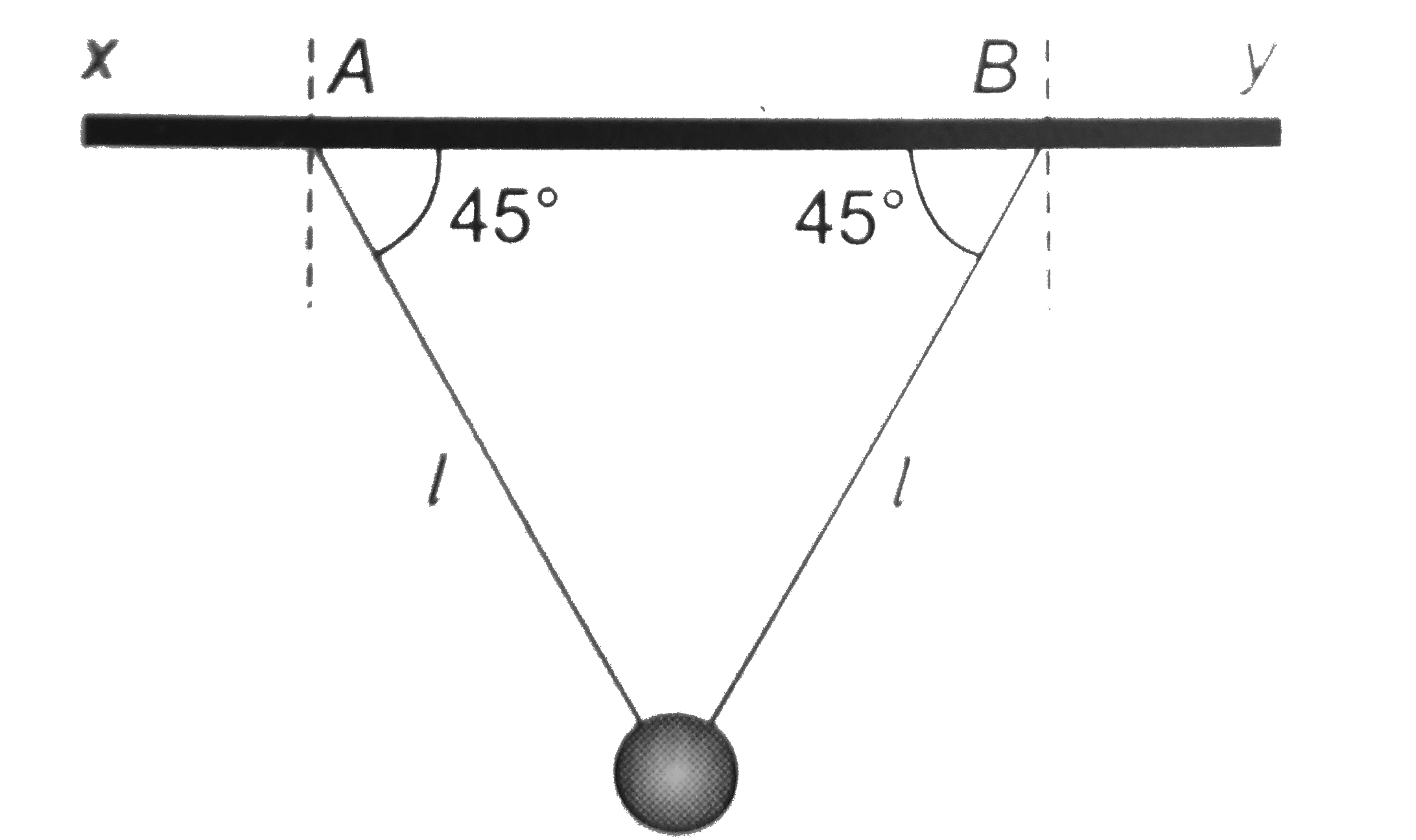
2π√2√2lg
2π√√2lg
2π√lg
2π√l√2g
The correct Answer is:D
Resulting torque on the bob =mgl√2sinθ
Ml of bob about axis xy=ml22
For small angle θ=mgl√2sinθ
α=τI=√2glθ
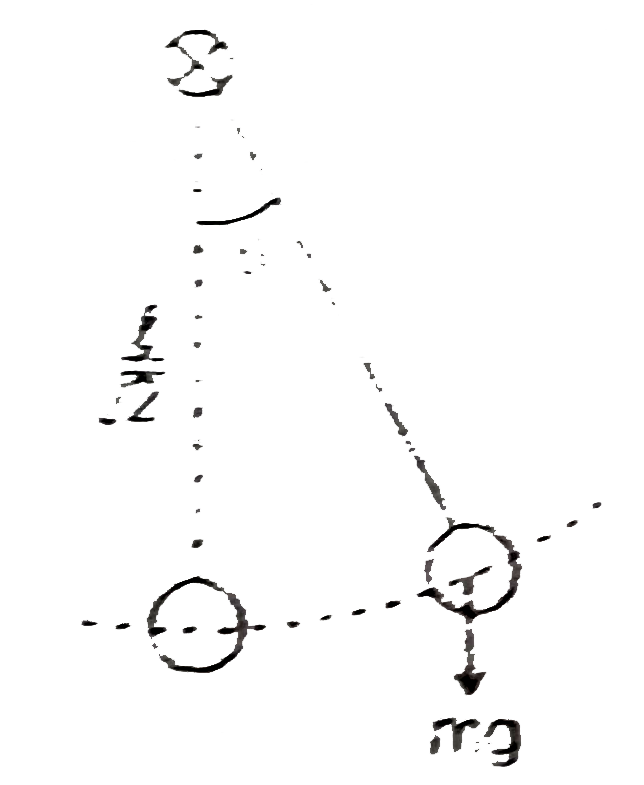
ω=√√2gk⇒T=2π√l√2g.
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.7110videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.7220videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.6920videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)21videos
CURRENT ELECTRICITY
- BOOK - RESONANCECHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 327videos
ELECTRO MAGNETIC WAVES
Similar Questions

A weightless rigid rod with a small iron bob at the end is hinged at point A to the wall so that it can rotate in all directions. The rod is kept in the horizontal position by a vertical inextensible string of length 20cm, fixed at its midpoint. The bob is displaced slightly perpenducular to the plane of the rod and string. Find period of small oscillations of the system in the form πX10s and fill the value of X.View SolutionA pendulum bob of mass m and length L is released from angle θ with the vertical. Find
(a) the speed of the bob at the bottom of the swing and
(b) tension in the string at that timeView Solution
Knowledge Check
- Question 1 - Select One
Time period of small oscillation (in a verical plane normal to the plane of strings) of the bob in the arrangement shown will be
_E01_023_Q01.png) A2π√lgB2π√l2gC2π√√2lgD2π√2lg
A2π√lgB2π√l2gC2π√√2lgD2π√2lg - Question 2 - Select One
A simple pendulum of length 1 m the bob performs circular motion in horizontal plane if its string making an angle 60∘ with the verticle , then the period of rotation of the bob will be (g=10m/s2
A2sB1.4sC1,98sD4s - Question 3 - Select One
A small ball B of mass m is suspended with light inelastic string of length L from a block A of same mass in which can move on smooth horizontal surface as shown in the figure. The ball is displaced by angle θ from equilibrium position and then released.
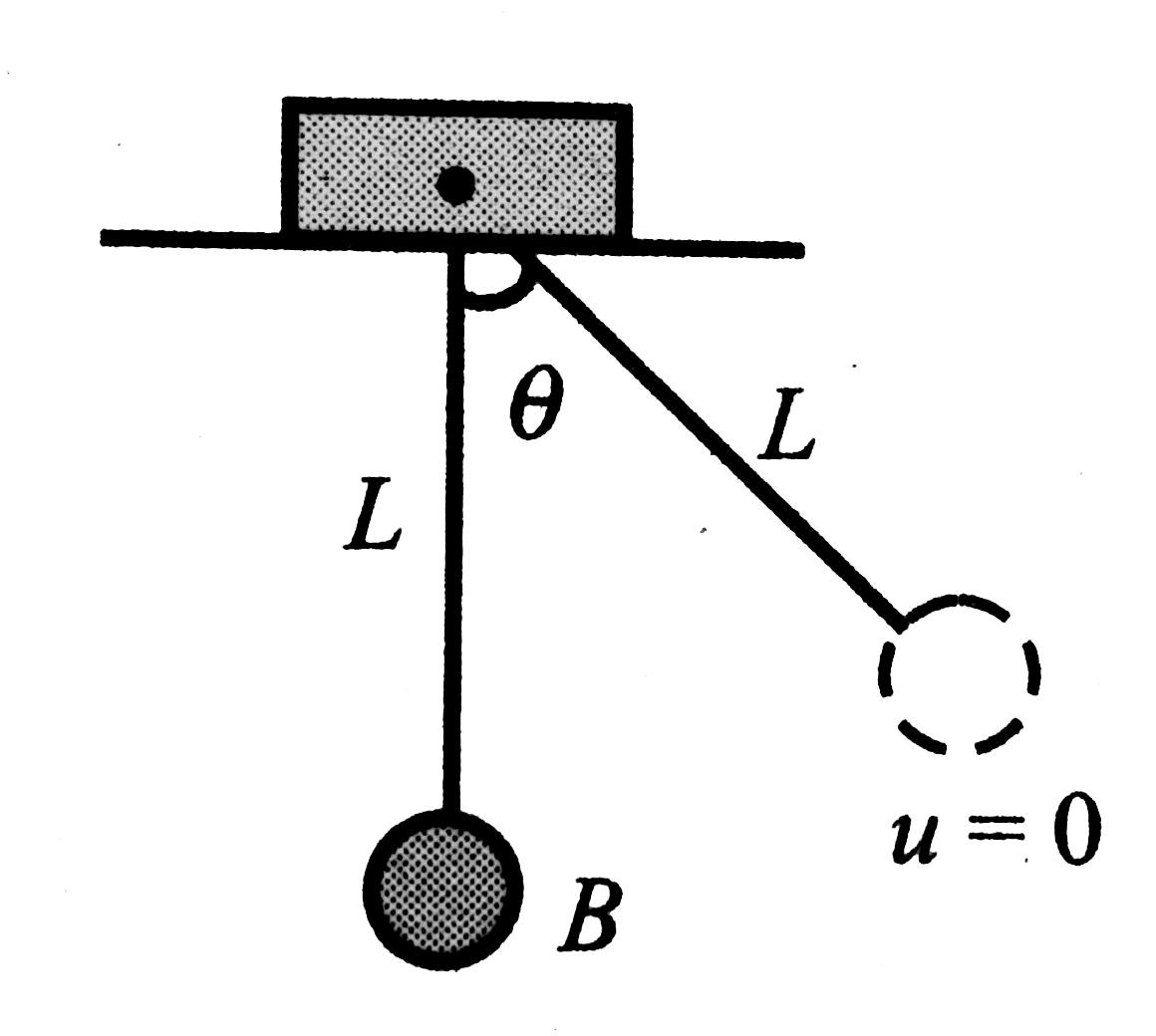 The displacement of centre of mass of A+B system till the string becomes vertical is AzeroBL(1−cosθ)CL2(1−cosθ)Dnone of these
The displacement of centre of mass of A+B system till the string becomes vertical is AzeroBL(1−cosθ)CL2(1−cosθ)Dnone of these
A small ring of mass m1 is connected by a string of length l to a small heavy bob of mass m2. The ring os free to move (slide) along a fixed smooth horizontal wire. The bob is given a small displacement from its equilibrium position at right angles to string. Find period of small oscillations.
_E01_106_Q01.png) View Solution
View SolutionA spherical bob of mass m and radius R is attached to a fixed point by means of a massless rigid rod whose length from the point of support up to the centre of bob is l. Find the period of small oscillation.
 View Solution
View SolutionThe ring R in the arrangement shown can slide along a smooth, fixed, horizontal rod XY. It is attached to the block B by a light string. The block is released from rest, with the string horizontal
 View Solution
View SolutionThe ring R in the arrangement shown can slide along a smooth fixed, horizontal rod XY. It is attached to the block B by a light string. The block is released from rest, with the string horizontal. Then which of the following are true.
 View Solution
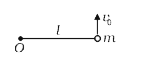
View SolutionA bob in tied to a string of length l & and kept on a horizontal rough surface as shown in the figure when point O of the string is fixed. The bob is given a tangential velocity V0 keeping the string taut as shown in figure. Find the tension in the string as a function of time. The coefficient of kinetic friction is μ.
 View Solution
View Solution

