There are 10 professors and 20 students out of whom a committee of 2
professors and 3 students is to be formed. Find the number of ways in which
this can be done. Further find in how many of these committees:
A particular professor is included
A particular student is included
A Particular student is excluded
To solve the problem, we need to break it down into parts. We have 10 professors and 20 students, and we need to form a committee consisting of 2 professors and 3 students.
Step 1: Calculate the total number of ways to form the committee
1. Choosing 2 professors from 10:
The number of ways to choose 2 professors from 10 is given by the combination formula:
(nr)=n!r!(n−r)!
Here, n=10 and r=2:
(102)=10!2!(10−2)!=10×92×1=45
2. Choosing 3 students from 20:
Similarly, the number of ways to choose 3 students from 20 is:
(203)=20!3!(20−3)!=20×19×183×2×1=1140
3. Total number of committees:
The total number of ways to form the committee is the product of the two combinations:
Total Committees=(102)×(203)=45×1140=51300
Step 2: Find the number of committees with specific conditions
A. A particular professor is included
1. If a particular professor is included, we only need to choose 1 more professor from the remaining 9:
(91)=9
2. We still need to choose 3 students from 20:
(203)=1140
3. Total committees with a particular professor included:
Committees with Professor=(91)×(203)=9×1140=10260
B. A particular student is included
1. If a particular student is included, we need to choose 2 more students from the remaining 19:
(192)=19×182×1=171
2. We still need to choose 2 professors from 10:
(102)=45
3. Total committees with a particular student included:
Committees with Student=(102)×(192)=45×171=7695
C. A particular student is excluded
1. If a particular student is excluded, we need to choose 3 students from the remaining 19:
(193)=19×18×173×2×1=969
2. We still need to choose 2 professors from 10:
(102)=45
3. Total committees with a particular student excluded:
Committees without Student=(102)×(193)=45×969=43605
Summary of Results
- Total number of committees: 51300
- Committees with a particular professor included: 10260
- Committees with a particular student included: 7695
- Committees with a particular student excluded: 43605
The number of ways to choose 2 professors from 10 is given by the combination formula:
(nr)=n!r!(n−r)!
Here, n=10 and r=2:
(102)=10!2!(10−2)!=10×92×1=45
Similarly, the number of ways to choose 3 students from 20 is:
(203)=20!3!(20−3)!=20×19×183×2×1=1140
The total number of ways to form the committee is the product of the two combinations:
Total Committees=(102)×(203)=45×1140=51300
(91)=9
(203)=1140
Committees with Professor=(91)×(203)=9×1140=10260
(192)=19×182×1=171
(102)=45
Committees with Student=(102)×(192)=45×171=7695
(193)=19×18×173×2×1=969
(102)=45
Committees without Student=(102)×(193)=45×969=43605
- Total number of committees: 51300
- Committees with a particular professor included: 10260
- Committees with a particular student included: 7695
- Committees with a particular student excluded: 43605
Topper's Solved these Questions
Similar Questions
There are 10 professors and 20 students out of whom a committee of 2 professors and 3 students is to be formed. Find the number of ways in which this can be done. Further find in how many of these committees:
A particular professor is included
A particular student is included
A Particular student is excludedView SolutionThere are 10 professors and 20 lecturers, out of whom a committee of 2 professors and 3 lecturers is to be formed. Find
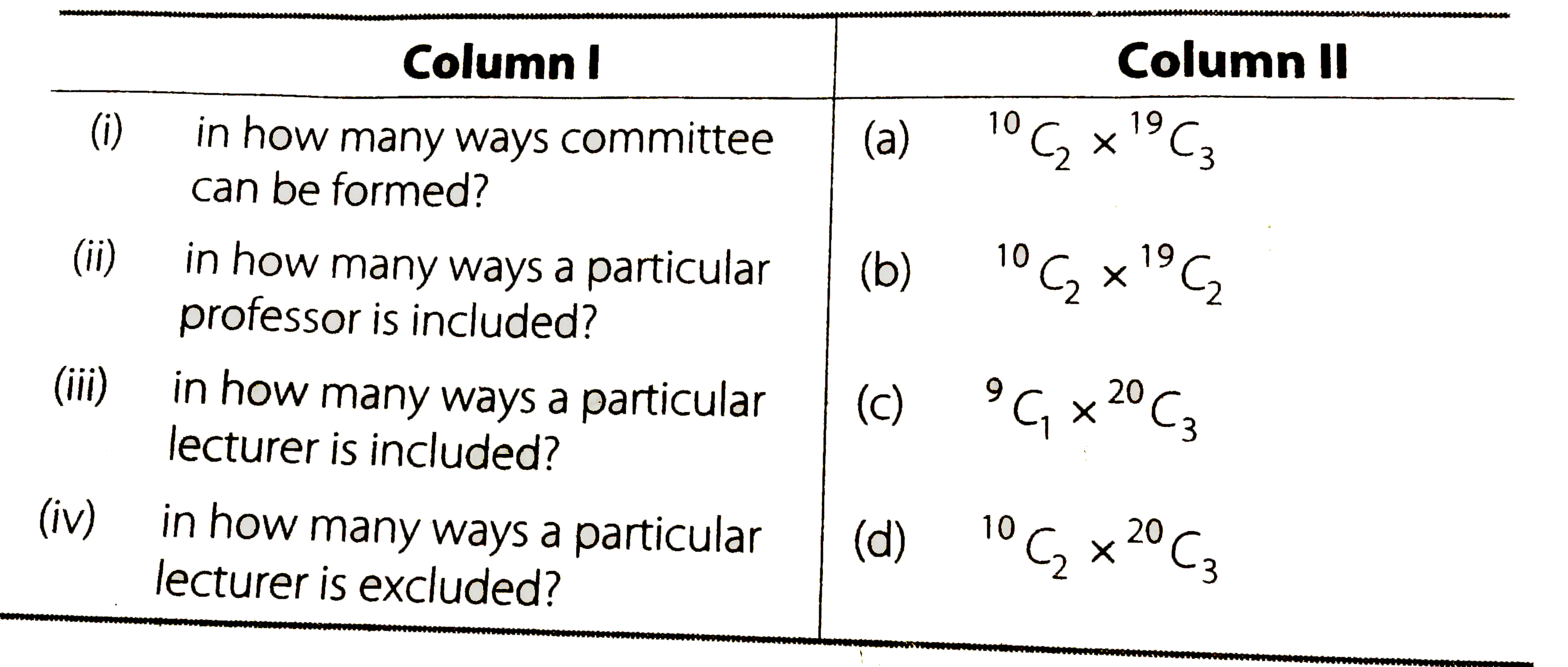 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
There are 25 students in a class . Find the number of ways in which a commitee of 3 students is to be formed.
A2200B2300C2400D3200 - Question 2 - Select One
The ratio of cost price and selling price of an article is 25:26. Find the gain percentage.
Aa.6Bb.5Cc.4Dd.3 - Question 3 - Select One
A 3cm long perpendicular is drawn from the centre of a circle to a 8cm long chord. Find the diameter of the circle.
Aa. 6cmBb. 5cmCc. 4cmDd. 10cm
Out of the 12 professors a committee of 5 professors is to be formed . Find the number of ways in which this can be done if 3 particular professors is excluded ?
View SolutionFrom 6 boys nd 7 girls a committee of 5 is to be formed so as to include at least one girl. Find the number of ways in which this can be done.
View SolutionFrom 10 boys and 20 girls, a committee of 2 boys and 3 girls is to be formed. In how many ways can this be done if a particular boy is included,
View SolutionFrom 10 boys and 20 girls, a committee of 2 boys and 3 girls is to be formed. In how many ways can this be done if a particular girl is included
View SolutionFrom 10 boys and 20 girls, a committee of 2 boys and 3 girls is to be formed. In how many ways can this be done if a particular girl is excluded
View Solution

