The radius of the orbit of an electron in a Hydrogen-like atom is $4.5 a_{0}$ , where $a_{0}$ is the Bohr radius. Its orbital angular momemtum is $\frac{3 h}{2 π}$ . It is given that $h$ is Plank constant and $R$ si Rydberg constant. The possible wavelength $(s)$ , when the atom-de-excites. is (are):

A

$\frac{9}{32 R}$

B

$\frac{9}{16 R}$

C

$\frac{9}{5 R}$

D

$\frac{4}{3 R}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, C

$R_{n} = 4.5 a_{0}$
$L = m v r = \frac{3 h}{2 π} [a s n = 3, z = 2]$
$\frac{1}{λ} = R z^{2} ⎛ ⎝ \frac{1}{n_{f}^{2}} - \frac{1}{n_{1}^{2}} ⎞ ⎠$
$\frac{1}{λ_{3 \to 1}} = R 4 [\frac{1}{1} - \frac{1}{9}] = 4 R \frac{8}{9} \Rightarrow λ_{3 \to 1} = \frac{9}{32 R}$
$\frac{1}{λ_{2 \to 1}} = R 4 [\frac{1}{1} - \frac{1}{4}] = \frac{3}{4} R \Rightarrow λ_{2 \to 1} = \frac{1}{3 R}$
$\frac{1}{λ_{3 \to 2}} R 4 [\frac{1}{4} - \frac{1}{9}] = \frac{5}{36} 4 R \Rightarrow λ_{3 \to 2} = \frac{9}{5 R}$

|

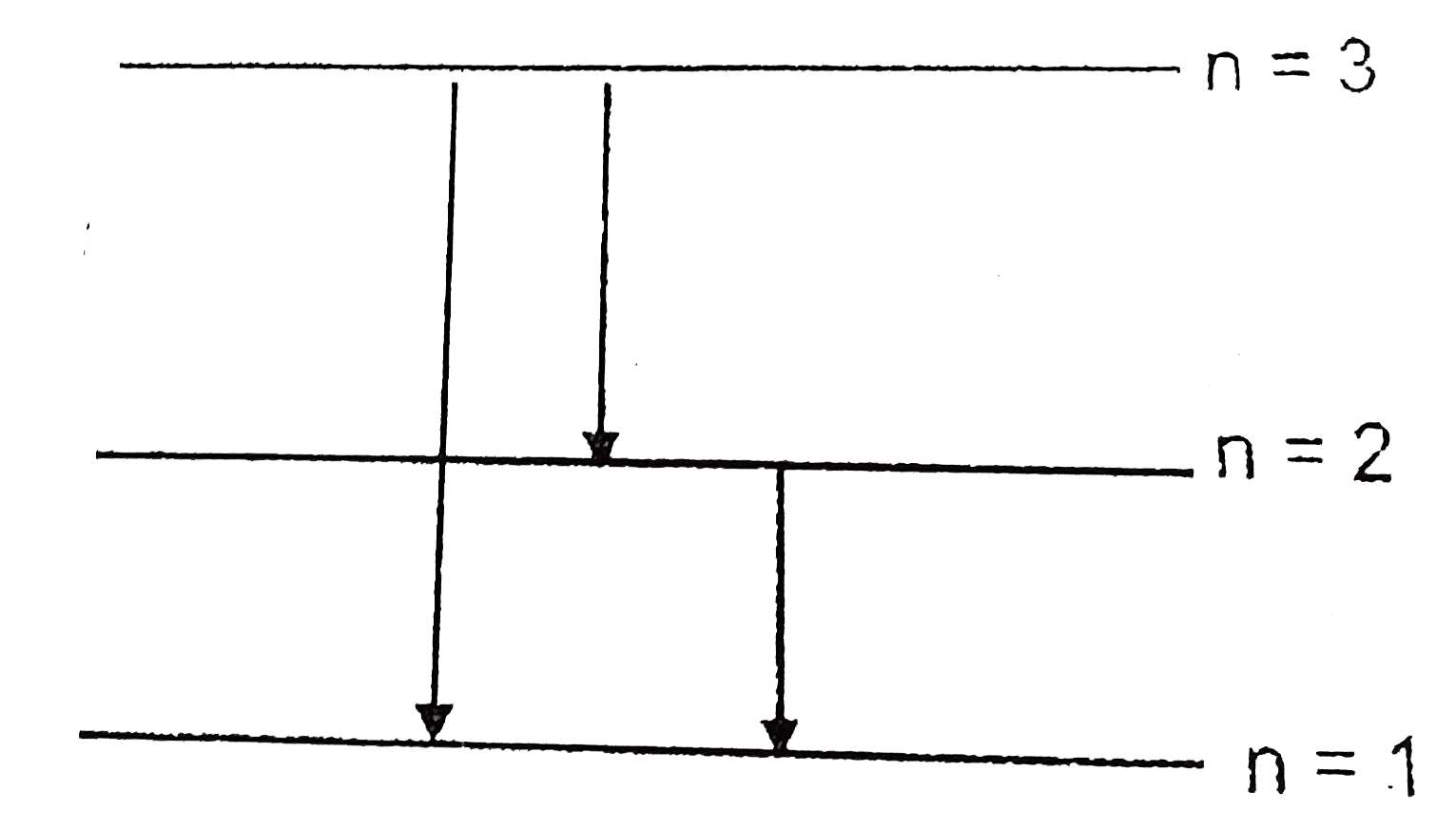
Updated on:21/07/2023

Class 12 PHYSICS ATOMS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One or More
The radius of the orbit of an electron in a Hydrogen - like atom is $4.5 s_{0}$ where $s_{0}$ is the bohr radius its orbital angular momentum is $\frac{3 b}{2 π}$ it is given that is is plank constant and R is rabdery constant .The possible wavelength $(s)$ , when the atom de- exciter , is (are)
A $\frac{9}{32 R}$
B $\frac{9}{16 R}$
C $\frac{9}{5 R}$
D $\frac{4}{3 R}$
Question 2 - Select One
The velocity of electrons in the 3rd excited state of a hydrogen atom is [ $a_{0}$ is Bohr radius ]:
A $\frac{h^{2}}{3 π^{2} m a_{0}}$
B $\frac{h^{2}}{3 π^{2} m a_{0}^{2}}$
C $\frac{8 h}{π m a_{0}}$
D $\frac{h}{8 π m a_{0}}$
Question 3 - Select One
The kinetic energy of an electron in the second Bohr orbit of a hydrogen atom is [ $a_{0}$ is Bohr radius] :
A $\frac{h^{2}}{4 π^{2} m a_{0}^{2}}$
B $\frac{h^{2}}{16 π^{2} m a_{0}^{2}}$
C $\frac{h^{2}}{32 π^{2} m a_{0}^{2}}$
D $\frac{h^{2}}{64 π^{2} m a_{0}^{2}}$

RESONANCE-ATOMIC PHYSICS-Exercise -3 part -I JEE (Advanced)

Home
Ask Doubt
Profile