If f(x)=14x2+2x+1 , then its maximum value is
(a) 43
(b) 23
(c) 1
(d) 34
To find the maximum value of the function f(x)=14x2+2x+1, we will follow these steps:
Step 1: Find the first derivative f′(x)
To find the critical points, we first need to compute the derivative of f(x).
Using the quotient rule, we have:
f′(x)=0⋅(4x2+2x+1)−1⋅(8x+2)(4x2+2x+1)2
This simplifies to:
f′(x)=−(8x+2)(4x2+2x+1)2
Step 2: Set the first derivative to zero
To find the critical points, we set f′(x)=0:
−(8x+2)=0
Solving for x:
8x+2=0⟹8x=−2⟹x=−14
Step 3: Determine if it is a maximum or minimum
To determine whether this critical point is a maximum or minimum, we can use the first derivative test. We will check the sign of f′(x) around x=−14.
- For x<−14 (e.g., x=−1):
f′(−1)=−(8(−1)+2)(4(−1)2+2(−1)+1)2=−(−8+2)(4−2+1)2=6(3)2>0
- For x>−14 (e.g., x=0):
f′(0)=−(8(0)+2)(4(0)2+2(0)+1)2=−2(1)2<0
Since f′(x) changes from positive to negative at x=−14, this indicates that there is a local maximum at this point.
Step 4: Calculate the maximum value
Now we need to find the maximum value of f(x) at x=−14:
f(−14)=14(−14)2+2(−14)+1
Calculating the denominator:
=4⋅116−12+1=14−24+44=34
Thus,
f(−14)=134=43
Conclusion
The maximum value of f(x) is 43.
Answer
The correct answer is (a) 43.
---
To find the critical points, we first need to compute the derivative of f(x).
f′(x)=0⋅(4x2+2x+1)−1⋅(8x+2)(4x2+2x+1)2
This simplifies to:
f′(x)=−(8x+2)(4x2+2x+1)2
To find the critical points, we set f′(x)=0:
−(8x+2)=0
Solving for x:
8x+2=0⟹8x=−2⟹x=−14
To determine whether this critical point is a maximum or minimum, we can use the first derivative test. We will check the sign of f′(x) around x=−14.
f′(−1)=−(8(−1)+2)(4(−1)2+2(−1)+1)2=−(−8+2)(4−2+1)2=6(3)2>0
- For x>−14 (e.g., x=0):
f′(0)=−(8(0)+2)(4(0)2+2(0)+1)2=−2(1)2<0
Now we need to find the maximum value of f(x) at x=−14:
f(−14)=14(−14)2+2(−14)+1
Calculating the denominator:
=4⋅116−12+1=14−24+44=34
Thus,
f(−14)=134=43
The maximum value of f(x) is 43.
The correct answer is (a) 43.
Topper's Solved these Questions
Similar Questions
A gardener has supply of fertilizer A which consists of 10% nitrogen and 6% phosphoric acid and fertilizer B which consists of 5% nitrogen and 100/6 phosphoric acid. After testing the soil conditions, he finds that he needs at least 14 kg of nitrogen and 14 kg of phosphoric acid for his crop. If fertilizer A costs 10.60 per kg and fertilizer B costs 8A0 per kg, what is the minimum 'cost at which the farmer can meet the nutrient requirement by using a combination of both types of fertilizers? Rs.1488 b. Rs.1576 c. Rs.1648
View SolutionFind the probability distribution of the number of doublets in four throws of a pair of dice. Also find the mean and variance of this distribution.
View Solution[Co(NH3)5SO4]Br and [Co(NH3)5Br]SO4 shown _________isomerism .
View SolutionThe value of |[a,a+d,a+2d] , [a+d,a+2d,a+3d] , [a+2d,a+3d,a+4d]|+|[b
View SolutionChoose the chain terminating step
(1) H2→H⋅+H⋅
(2) Br2→Br⋅+Br⋅
(3) Br⋅+HBr→H⋅+Br2
(4) H⋅+Br2→HBr+Br⋅
(5) Br⋅+Br⋅→Br2
View SolutionIn CH_(3)CH_(2)Br,% of Br is
View SolutionWhich one of the following is more reactive towards SN2 mechanism.
((CH3)3C−Br). ((CH2)3CH−Br). ((CH3)CH2−Br). (CH_3-Br).
View Solution1) Fragmentation () a) Planaria br 2) Unripe fruits () b) Yeast br 3) Desire () c) Spirogyra br 4) Reproduction () d) Watermelon br
View SolutionCan Fe3+ oxidize Br− to Br2 at 1 M concentration ?
E∘(Fe3+∣Fe2+=0.77VandE∘(Br∣Br−)=1.09V
View Solution[" Which alkyl bromide will yield only one alkene upon "E_(2)" elemination? "],[[" 1) "," ( "" Br "," 2) "," Br "" 3) "," Br "," ( "" ) "," ( Br "]]
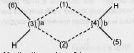
View SolutionWite down the corresponding elements in the figure : 1)……………… br 2)…………… br 3).……………. Br 4)………….. Br 5)…………. Br 6)…………..
View Solution[Co(NH3)5SO4]Br and [Co(NH3)5Br]SO4 show _________isomerism .
View Solution[Co(NH_(3))_(5)SO_(4)]Br and [Co(NH_(3))_(5)Br]SO_(4) shown _________isomerism .
View SolutionThe oxidation states of V and Br in V(BrO2)2 are respectively : 2 and 2 , 2 and 1, 4 and 2, 2 and 3
View Solution[Co(NH3)5Br]SO4and[Co(NH3)5SO4]Br show …. isomerism.
View Solution[Co(NH_(3))_(5)Br]SO_(4)and[Co(NH_(3))_(5)SO_(4)]Br show …. isomerism.
View Solution