Ballistic Pendulum: A ballistic pendulum is used to measure speed of bullets. It consists of a wooden blocksuspended from fixed support.
A wooden block mass M is suspended with the help of two threads to prevent rotation while swinging. A bullet of mass m moving horizontally with velocity v0 hits the block and becomes embedded in the block. Receiving momentum from the bullet, the bullet in terms of given quatities.

When the bullet hits the block, in a negligible time interval, it becomes embedded in the block system starts moving horizontally. During this process, net force acting on the bullet-lock system in vertical direction is zero and no force acts in the horizontal direction. Therefore, momentum if the bullet-block system remains conserved.
Let us denote momentum of the bullet-block system immediately after the bullet becomes embedded in the block by p and apply principle of conservation of momentum to the system for horizontal component of momentum.
p=mv0
Using equation K=p22m, we can find kinetic energy K1 of the bullet-block system immediately after the bullet becomes embedded in the block.
K1=(mv0)22(M+m)
During swing, only gravity does not work on the bullet-block system. Applying work-kinetic energy theorm during swing of the bullet-block system, we have
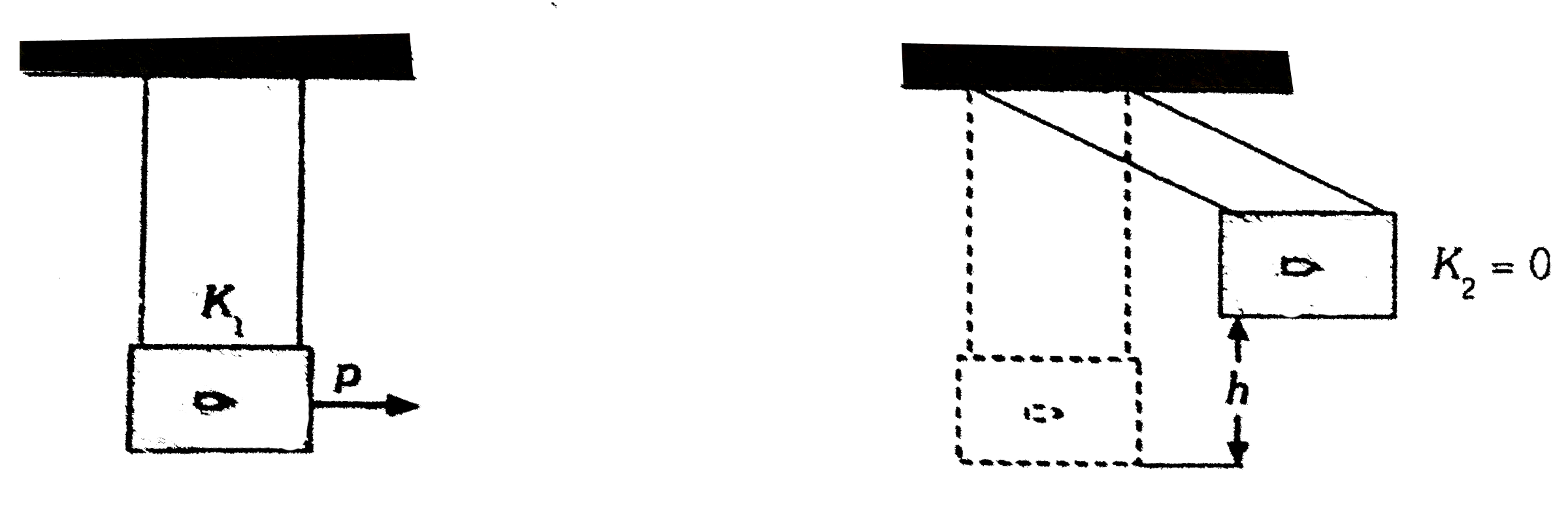
W1→2=K2−K1→ −(M+m)gh=0−(mv0)22(M+m)
Rearringing terms, we have v0=(M+m)m√2gh
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-I40videos
CENTRE OF MASS
- BOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II43videos
CENTRE OF MASS
- BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON11videos
BASIC MATHEMATICS USED IN PHYSICS &VECTORS
- BOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)3videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
Similar Questions
A bullet of mass 0.1Kg moves with velocity 10ms it strikes a block and comes to rest after travels 0.5 m inside block. Find retardation of bullet
View SolutionA bullet of mass 0.1Kg moves with velocity 10ms it strikes a block and comes to rest after travels 0.5 m inside block. Find retardation of bullet
View Solution
Knowledge Check
- Question 1 - Select One
A bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
A12mv2×m(m+M)B12mv2×M(m+M)C12mv2×(M+m)mD12Mv2×m(m+M) - Question 2 - Select One
A bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
A12mv2×m(m+M)B12mv2×M(m+M)C12mv2×(M+m)MD12mv2(M+m)m - Question 3 - Select One
A bullet of mass m moving horizontally with a velocity v strikes a block of wood of mass M and gets embedded in the block. The block is suspended from the ceiling by a massless string. The height to which block rises is
Av22g(mM+m)2Bv22g(M+mm)2Cv22g(mM)2Dv22g(Mm)2
A wooden block of mass 0.9kg is suspended from the ceiling of a room by thin wires. A bullet of mass 0.1kg moving horizontally with a speed of 10ms−1 strikes the block and sticks to it. What is the height to which the block rises ? Take g=10ms−2:
View SolutionA bullet of mass m moving with a speed v strikes a wooden block of mass M & gets embedded into the block. The final speed is
View SolutionA bullet is fired into a wooden block. If the bullet gets embedded in wooden block, then
View SolutionA bullet of mass m moving with velocity υ1 strikes a suspended wooden block of mass M as shown in the figure and sticks to it. If the block rises to a height y. the initial of the bullet is
 View Solution
View SolutionA bullet of mass 'x' moves with a velocity y, hits a wooden block of mass z at rest and gets embedded in it. After collision, the wooden block and bullet moves with a velocity
View Solution

