If f(x)≥0 and g(x)≥0 for all real x, which of the following statements must be true ?
I. f(x)+g(x)≥0
II. f(x)−g(x)≥0
III. f(x)g(x)≥0
A
I only
B
II only
C
III only
D
I and III
Video Solution
Text Solution
The correct Answer is:D
Don't let the functions symbolism confuse you. Just think of these as two quantities - f(x) and g(x) - that are both nonnegative. Statement I says their sum is nonnegative. That's true - add any two nonnegatives and you'll get a nonnegative sum. Statement II says the difference f(x)−g(x) is nonnegative. Well, that's true only if f(x)≥g(x). But there's no reason that g(x) couldn't be greater than f(x). Statement III says their product is nonnegative. That's true - multiply any two nonnegatives and you'll get a nonnegative product. Statements I and III are true.
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
If f(x)≥0 for all x, then f(2-x) is
A≥−2B≥0C≥2D≤0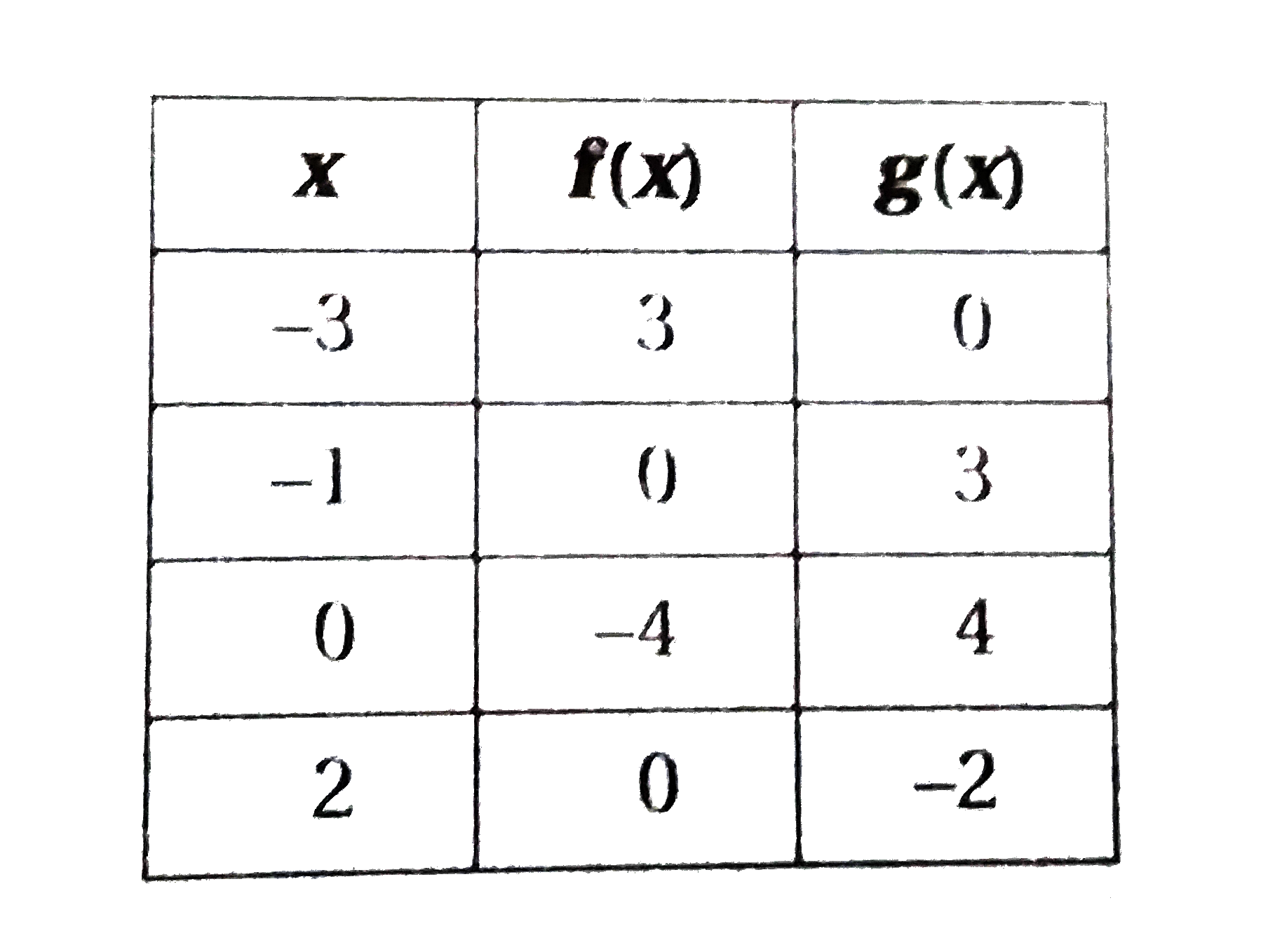
Several values of x, and the corresponding values for polynomial functions f and g are shown in the table above. Which of the following statements is true?
I. f(0)+g(0)=0
II. f(x) is divisible by x+2
III. g(x) is divisible by x+3.AI, II, and IIIBI and II, onlyCII and III, onlyDI and III, only
f(x)=ex[x+1],x≥0
View Solution2x+y≥6,x+2y≥8,x≥0,y≥0
View Solution2x+y≤6,x+2y≤8,x≥0,y≥0
View Solutionsolve for ,x where f(x)=|x|≥0
View SolutionA quadratic function f(x) satisfies f(x)≥0 for all real x. If f(2)=0 and f(4)=12, then the value of f(6) is :
View SolutionLet f(x) be a continuous function (define for all x) which satisfies f3(x)−5f2(x)+10f(x)−12≥0,f2(x)+3≥0andf2(x)−5f(x)+6≤0
The equation of tangent that can be drawn from (2,0) on the curve y=x2f(sinx) is :
View Solution
