A circle center (3, 8) contains the point (2, -1). Which of the following is also a point on the circle ?
(1, -10)
(4, 17)
(5, -9)
(7, 15)
The correct Answer is:B
To solve the problem step by step, we need to determine which of the given points lies on the circle centered at (3, 8) that contains the point (2, -1).
Step 1: Find the radius of the circle
The radius of the circle can be calculated using the distance formula between the center of the circle (3, 8) and the point (2, -1).
The distance formula is given by:
d=√(x2−x1)2+(y2−y1)2
Here, (x1,y1)=(3,8) and (x2,y2)=(2,−1).
Calculating the distance:
d=√(2−3)2+(−1−8)2
=√(−1)2+(−9)2
=√1+81
=√82
Thus, the radius r of the circle is √82.
Step 2: Write the equation of the circle
The general equation of a circle with center (h,k) and radius r is:
(x−h)2+(y−k)2=r2
Substituting h=3, k=8, and r=√82:
(x−3)2+(y−8)2=82
Step 3: Check each option to see if it satisfies the equation
We need to check which of the given points satisfies the equation (x−3)2+(y−8)2=82.
Assuming the options are:
- A: (1, -10)
- B: (4, 17)
- C: (5, -9)
- D: (7, 15)
Check Option A: (1, -10)
(1−3)2+(−10−8)2=(−2)2+(−18)2=4+324=328(not equal to 82)
Check Option B: (4, 17)
(4−3)2+(17−8)2=(1)2+(9)2=1+81=82(equal to 82)
Check Option C: (5, -9)
(5−3)2+(−9−8)2=(2)2+(−17)2=4+289=293(not equal to 82)
Check Option D: (7, 15)
(7−3)2+(15−8)2=(4)2+(7)2=16+49=65(not equal to 82)
Conclusion
The only point that satisfies the equation of the circle is Option B: (4, 17).
The radius of the circle can be calculated using the distance formula between the center of the circle (3, 8) and the point (2, -1).
d=√(x2−x1)2+(y2−y1)2
Here, (x1,y1)=(3,8) and (x2,y2)=(2,−1).
d=√(2−3)2+(−1−8)2
=√(−1)2+(−9)2
=√1+81
=√82
The general equation of a circle with center (h,k) and radius r is:
(x−h)2+(y−k)2=r2
Substituting h=3, k=8, and r=√82:
(x−3)2+(y−8)2=82
We need to check which of the given points satisfies the equation (x−3)2+(y−8)2=82.
- A: (1, -10)
- B: (4, 17)
- C: (5, -9)
- D: (7, 15)
(1−3)2+(−10−8)2=(−2)2+(−18)2=4+324=328(not equal to 82)
(4−3)2+(17−8)2=(1)2+(9)2=1+81=82(equal to 82)
(5−3)2+(−9−8)2=(2)2+(−17)2=4+289=293(not equal to 82)
(7−3)2+(15−8)2=(4)2+(7)2=16+49=65(not equal to 82)
The only point that satisfies the equation of the circle is Option B: (4, 17).
Topper's Solved these Questions
Similar Questions
(1,2√2)is a point on circle, x2+y2=9. Which of the following is not the point on the circle at 2 units distance from (1,2√2)?
View SolutionPoint M moves on the circle (x−4)2+(y−8)2=20. Then it brokes away from it and moving along a tangent to the circle, cuts the x-axis at the point (-2,0). The co-ordinates of a point on the circle at which the moving point broke away is
View Solution
Knowledge Check
In the xy - plane, the point (0, 2) is the center of a circle that has radius 2. Which of the following is NOT a point on the circle?
A(0,4)B(−2,4)C(−2,2)D(0,0)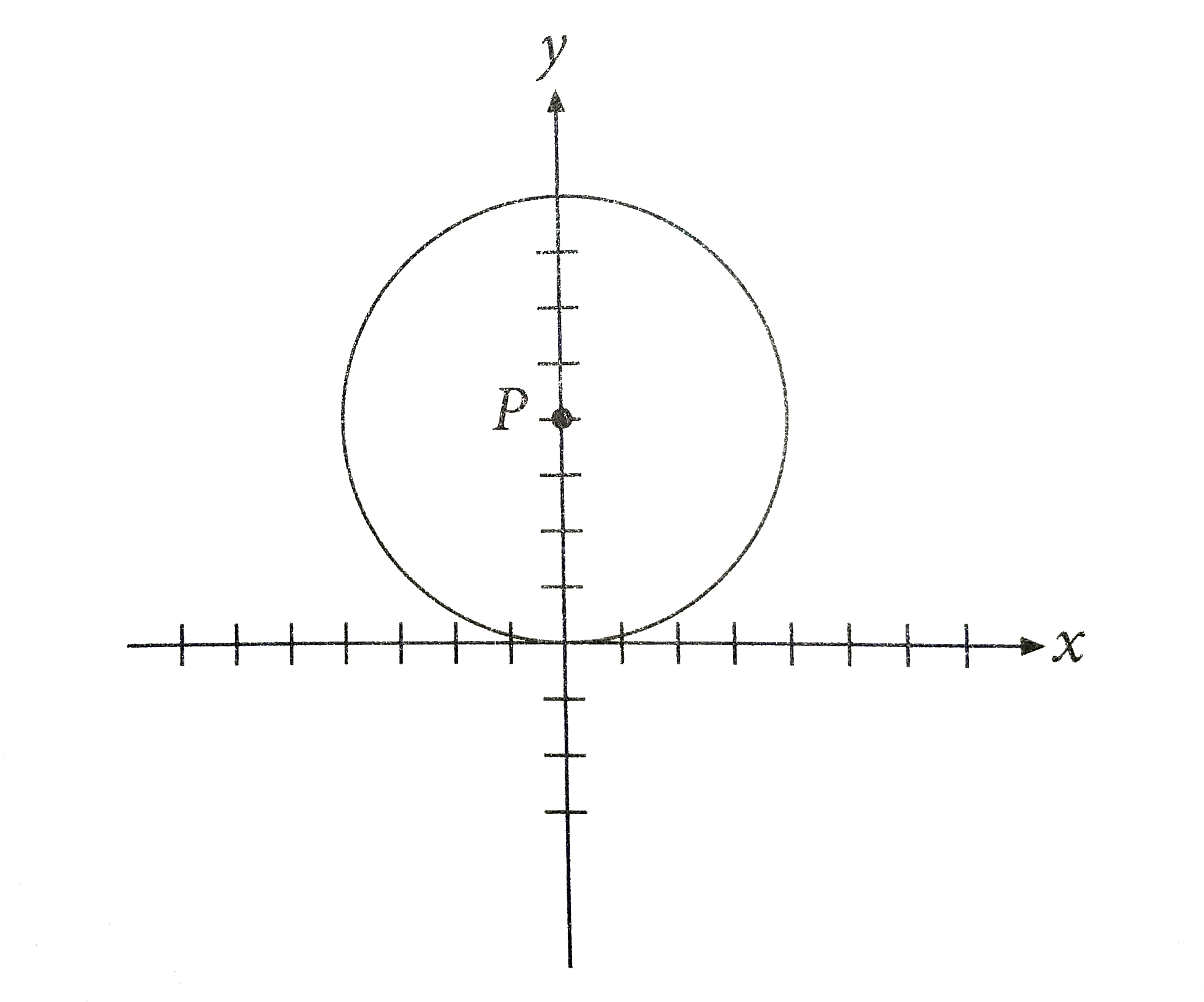
Point P is the certer of the circle shown above, which has a radius of 4. Which of the following points lies on circle P?A(4,0)B(0,4)C(−4,4)D(4,3)In the xy-plane, a circle with center O passes through the point (2, 0) and has a radius of 4. Which of the following could be the equation of circle O?
A(x−2)2+(y+4)2=4B(x−2)2+(y+4)2=16C(x−4)2+(y+2)2=16D(x+2)2+(y−2)2=16
Find the equation of the circle which has extremities of a diameter the origin and the point (2, - 4). Find also the equations of the tangents to the circle which are parallel to this diameter
View SolutionFind the equation of the Circle whose centre is at the point (4, 5) and which touches the x-axis. Also find the co-ordinates of the points at which the circle cuts the y-axis.
View SolutionThe center of circle O (not shown) falls on the point where the line y=43x+4 intersects the x-axis on the coordinate plane. The point (3, 8) lies on the circumference of the circle. Which of the following could be the equation for circle O?
View SolutionA circle in the xy‑plane has equation (x+3)2+(y−1)2=25. Which of the following points does NOT lie in the interior of the circle?
View SolutionIn the figure, ΔABC is inscribed in the circle. The triangle does not contain the center of the circle O. Which one of the following could be the value of x in degree?
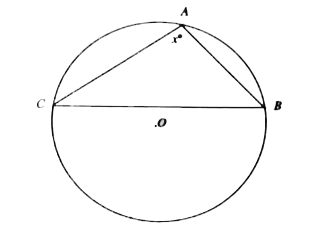
View Solution
