Suppose that a radio tube inserted into a certain type of set has probability 0.2 of functioning more than 500 hours. If we test 4 tubes at random what is the probability that exactly three of these tubes function for more than 500 hours?
Let denote the number of tubes that function for more than hours.
Then, follows a binomial distribution with
Let be the probability that the tubes function more than hours.
Here,
Hence, the distribution is given by
Therefore, required probability
Topper's Solved these Questions
Similar Questions
Suppose that a radio tube inserted into a certain type of set has probability 0.2 of functioning more than 500 hours. If we test 4 tubes at random what is the probability that exactly three of these tubes function for more than 500 hours?
View SolutionA word consist of 9 letters; 5 consonants and 4 vowels. Three letters are chosen at random. What is the probability that more than one vowel will be selected?
View Solution
Knowledge Check
- Question 1 - Select One
80 bulbs are selected at random from a lot and their lifetime in hours is recorded as under

One bulb is selected at random from the lot . What is the probability that the selected bulb has a life more than 500 hours ?A2740B2940C516D1140 - Question 2 - Select One
The percentages of marks obtained by a student in six unit tests are given below :

A unit test is selected at random . What is the probability that the student gets more than 60% marks in the test ?A15B14C12D13 - Question 3 - Select One
A word consists of 7 letters : 4 consonants and 3 vowels. If three letters are chosen at random, then the probability that more than one vowel will be selected, is
A1335B1742C735D1342
The probability that a positive two digit number selected at random has its tens digit at least three more than its unit digit is
View SolutionThe probability that a positive two digit number selected at random has its tens digit at least three more than its unit digit is -
View SolutionIn a locality of 5000 families were chosen at random and the following data was collected
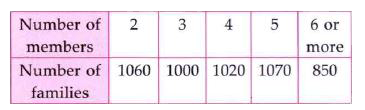
Out of these families, a family is chosen at random. What is the probability that the chosen family has less than 5 but more than 3 members?View SolutionThe bulbs produced in a factory are supposed to contain 5% defective bulbs . What is the probability that a sample of 10 bulbs will contain not more than 2 defective bulbs?
View SolutionFrom the set of numbers {1,2,3,4,5,6,7,8} two numbers are selected at random without replacement. The probability that their sum is more than 13 is
View Solution

