Two bad eggs are accidently mixed up with ten good ones. Three eggs are drawn at random with replacement from this lot. compute the mean for the number of bad eggs drawn.
The correct Answer is:Mean=12
Let X denote the number of bad eggs
Total number of eggs= bad eggs+good eggs=2+10=12
Now the probability of drawing bad eggs are:
P(X=0)=P(no bad egg or all three good eggs)
=10C312C3=10!3!7!12!3!9!
=10×9×8×7!3×2×7!12×11×10×9!3×2×9!=1222
P(X=1)=P(one bad egg and two good eggs)
=2C1×10C212C3=2!1!1!×10!2!8!12!3!9!
=(2×11×1)×(10×9×8!2×8!)12×11×10×9!3×2×9!=922
⇒P(X=2)=P(two bad eggs and one good egg)
=2C2×10C112C3=2!2!0!×10!1!9!12!3!9!
=(2×12×1)×(10×9!1×9!)12×11×10×9!3×2×9!=122
P(X=3)=P(3 bad eggs)=0
As there are only two eggs and we cannot draw three from them
Probability of Distribution:
X: 0 1 2
P(X): 1222 922 122
Now,
Mean=E(X)=Σxi⋅P(xi)=0×1222+1×922+2×122
=0+922+222=1122=12
⇒E(X)=122
Topper's Solved these Questions
Similar Questions
Four rotten oranges are accidentally mixed with 16 good ones. Three oranges are drawn at random from the mixed lot. Let X be the number of rotten oranges drawn. Find the mean and variance of X.
View SolutionFour bad eggs are mixed with 10 good ones. Three eggs are drawn one by one without replacement. Let X be the number of bad eggs drawn. Find the mean and variance of X.
View Solution
Knowledge Check
- Question 1 - Select One
Five defective mangoes are accidenty mixed with 15 good ones. Four mangoes are drawn at random from this lot. Then the probabilty distribution of the number of defective mangoes is
A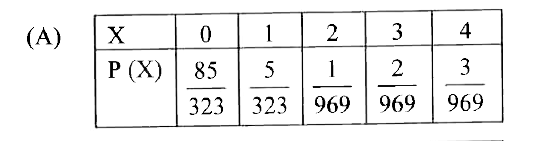 B
B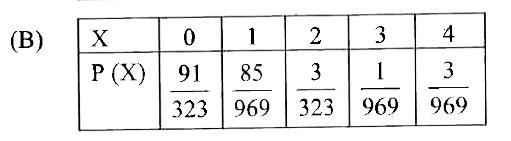 C
C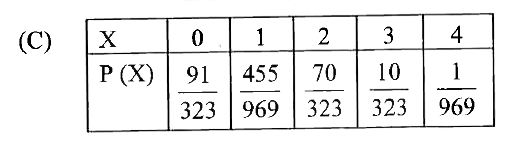 D
D
- Question 2 - Select One
An urn contains 4 white and 3 red balls. Three balls are drawn with replacement from this urn. Then, the standard deviation of the number of red balls/drawn is
A67B3649C57D2549
Five defective mangoes are accidentally mixed with 15 good ones. Four mangoes are drawn at random from this lot. Find the probability distribution of the number of defective mangoes.
View SolutionFive defective bolts are accidently mixed with twenty good ones. If four bolts are drawn at random from this lot, find the probability distribution of the number of defective bolts.
View SolutionFive bad oranges are accidently mixed with 20 good ones. If four oranges are drawn one by one successively with replacement, then find the probability distribution of number of bad oranges drawn. Hence find the mean and variance of the distribution.
View SolutionThere are 5 cards numbered 1 to 5, one number on one card. Two cards are drawn at random without replacement. Let X denote the sum of the numbers on two cards drawn. Find the mean and variance.
View SolutionThere are 5 cards numbered 1 to 5 , one number on one number on one card . Two cards are drawn at random without replacement . Let X denotes the sum of the numbers on two cards drawn . Find the mean and variance of X.
View SolutionThere are 4 cards numbered 1, 3, 5 and 7, one number on one card. Two cards are drawn at random without replacement. Let 'X' denote the sum of the numbers on the two drawn cards. Find the mean and variance of X.
View Solution

