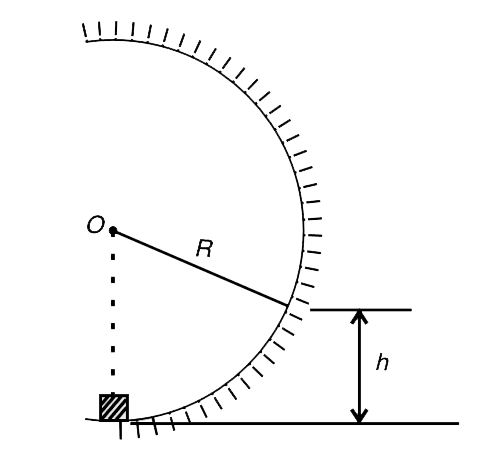
(i) There is a vertical loop of radius R. A small block of mass m is slowly pushed along the loop from bottom to a point at height h. Find the work done by the external agent if the coefficient of friction is μ. Assume that the external agent pushes tangentially along the path. (ii) A block of mass m slides down a smooth slope of height h, starting from rest. The lower part of the track is horizontal. In the beginning the block has potential energy U = mgh which gets converted into kinetic energy at the bottom. The velocity at bottom is v=√2gh. Now assume that an observer moving horizontally with velocity v=√2gh towards right observes the sliding block. She finds that initial energy of the block is E=mgh+12mv2 and the final energy of the block when it reaches the bottom of the track is zero. Where did the energy disappear?

The correct Answer is:(i)mgh+μmg√2Rh−h2
Step by step video, text & image solution for (i) There is a vertical loop of radius R. A small block of mass m is slowly pushed along the loop from bottom to a point at height h. Find the work done by the external agent if the coefficient of friction is mu. Assume that the external agent pushes tangentially along the path. (ii) A block of mass m slides down a smooth slope of height h, starting from rest. The lower part of the track is horizontal. In the beginning the block has potential energy U = mgh which gets converted into kinetic energy at the bottom. The velocity at bottom is v=sqrt(2gh). Now assume that an observer moving horizontally with velocity v=sqrt(2gh) towards right observes the sliding block. She finds that initial energy of the block is E=mgh+1/2 mv^(2) and the final energy of the block when it reaches the bottom of the track is zero. Where did the energy disappear? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
Similar Questions
Find the work done by an external agent in lowering a block of mass m slowly on a light of stiffness k, till it comes to rest.
View SolutionA particle of mass 'm' is raised from the surface of earth to a height h = 2R. Find work done by some external agent in the process. Here, R is the radius of earth and g the acceleration due to gravity on earth's surface.
View Solution
Knowledge Check
- Question 1 - Select One or More
A small mass m is moved slowly from the surface of the earth to a height h above the surface. The work done (by an external agent) in doing this is
Amgh, for all values of hBmgh, for h<<RC1/2 mgR for h=RD−1/2mgR for h=R - Question 2 - Select One
A small mass m is moved slowly from the surface of the earth to a height h above the surface. The work done (by an external agent) in doing this is
Amgh, for all values of hBmgh, for h<<RC1/2,mgR, for h=RD−1/2mgR, for h=R - Question 3 - Select One or More
A small mass m is moved slowly from the surface of the earth to a height h above the surface. The work done ( by an external agent) in doing this is
Amgh, for all values of hBmgh, for h<<RC12mgR for h = RD−12mgR, for h = R
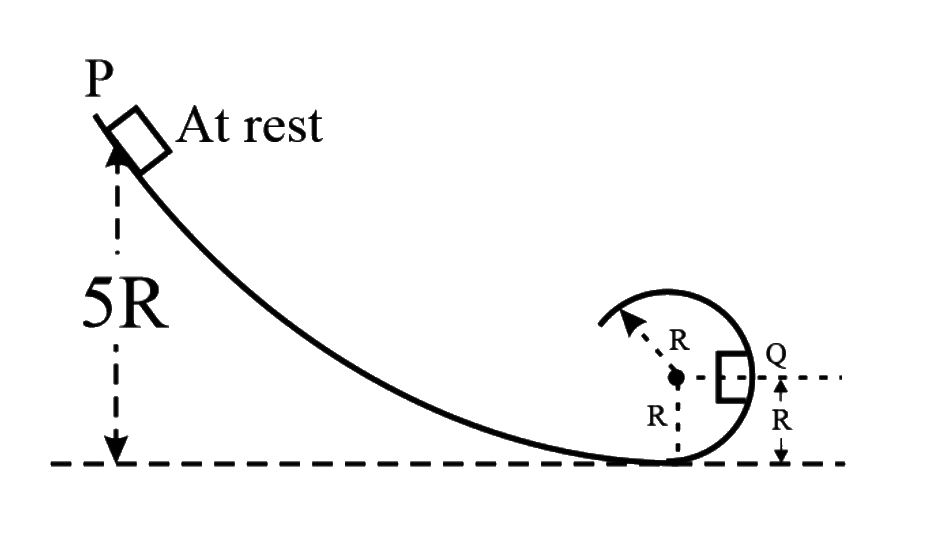
A small block of mass m slides along a smooth frictional track as shown in the figure. If it starts from rest at P, what is the resultant force acting on it at Q?
 View Solution
View SolutionTwo blocks of mass 2m and m slide down the slope AB having same friction coefficient Then:
 View Solution
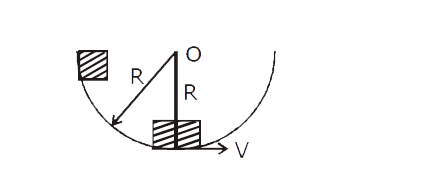
View SolutionA block of mass m slides down along the surface of the bowl from the rim to the bottom as shown in fig. The velocity of the block at the bottom will be-
 View Solution
View SolutionAn object of mass m is slowly pushed some partway up a verticle up a verticle loop ending at a point a height h<R above the bottom. The coefficient of friction between the object and the track is a constant mu. Find work that has to be done against friction.
 View Solution
View SolutionWork done by an external agent in bringing three particles each of mass m at the vertices of an equilateral triangle of length l is
View Solution

