Angular speed of rotation of the earth is ω0. A train is running along the equator at a speed v from west to east. A very sensitive balance inside the train shows the weight of n object as W1. During the return journey when the train is running atsame speed from east to west the balance shows the weight of the object to be W2. Weight of the object when the train is at rest was shown to be W0 by the balance. Calculate W2–W1.
The correct Answer is:4W0ω0vg
Step by step video, text & image solution for Angular speed of rotation of the earth is omega_(0). A train is running along the equator at a speed v from west to east. A very sensitive balance inside the train shows the weight of n object as W_(1). During the return journey when the train is running atsame speed from east to west the balance shows the weight of the object to be W_(2). Weight of the object when the train is at rest was shown to be W_(0) by the balance. Calculate W_(2) – W_(1). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Weight of an object on the moon is ____ the weight of the object on earth.
Aequal toB1/6thC1/2D1/5th - Question 2 - Select One
Weight of an object on the moon is _________the weight of the object on earth .
Aequal toB1/6 thC2/3D1/5 th - Question 3 - Select One
If the earth stops rotating, then the weight of an object at the north pole will
AdecreaseBincreaseChave twice its value at south poleDremain thee same
A train is running at a speed of 60 km/h. Calculate the speed of train in m/s.
View SolutionThe given figure represents a weighing balance. The weights of some objects in the balance are given. Find the weight of each square and the circle .
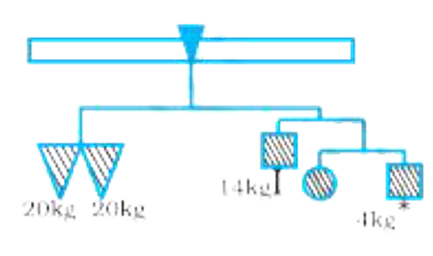 View Solution
View SolutionIf earth were to rotate faster than its present speed, the weight of an object
View SolutionIf the rotational speed of earth increase, then weight of body at the equator
View SolutionIf earth suddenly stop rotating , then the weight of an object of mass m at equator will [ ω is angular speed of earth and R is its radius . ]
View Solution

