Two particles have equal mass m and electric charge of equal magnitude (q) and opposite sign. The particles are held at rest at co-ordinates (– a, 0) and (a, 0) as shown in the figure. The particles are released simultaneously. Consider only the electrostatic force between the particles and the force applied by the external magnetic field on them.
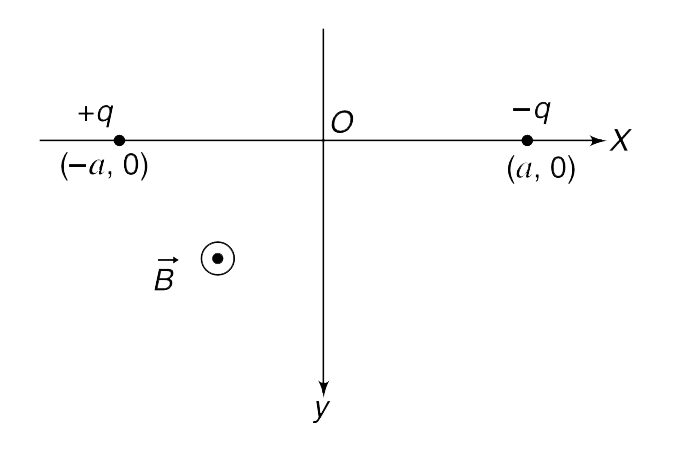
(a) Find the speed of negatively charged particle as function of its x co-ordinate.
(b) Find the y component of velocity of the negative particle as a function of its x co-ordinate.
The correct Answer is:(a)V=√kq22m(1x−1a) (b)Vy=qBm(a−x)
Step by step video, text & image solution for Two particles have equal mass m and electric charge of equal magnitude (q) and opposite sign. The particles are held at rest at co-ordinates (– a, 0) and (a, 0) as shown in the figure. The particles are released simultaneously. Consider only the electrostatic force between the particles and the force applied by the external magnetic field on them. (a) Find the speed of negatively charged particle as function of its x co-ordinate. (b) Find the y component of velocity of the negative particle as a function of its x co-ordinate. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MOTION OF CHARGE IN MAGNETIC FIELDBOOK - ARIHANTCHAPTER - MOTION OF CHARGE IN MAGNETIC FIELDEXERCISE - Level-3
4 Videos
- MOTION OF CHARGE IN MAGNETIC FIELDBOOK - ARIHANTCHAPTER - MOTION OF CHARGE IN MAGNETIC FIELDEXERCISE - Level-3
4 Videos
- MAGNETIC EFFECT OF CURRENTBOOK - ARIHANTCHAPTER - MAGNETIC EFFECT OF CURRENTEXERCISE - Magnetic effect of Current
45 Videos
- NUCLEAR PHYSICSBOOK - ARIHANTCHAPTER - NUCLEAR PHYSICSEXERCISE - All Questions
54 Videos
Similar Questions
Knowledge Check
Three charged particle sare in equilibrium under their electrostatic forces only. Then
Athe particle smust be collinearBall the charges cannot have the same magnitudeCall the charges cannot have the same signDthe equilibrium is unstableA charged particle is moved along a magnetic field line. The magnetic force on the particle is
Aalong its velocityBopposite to its velocityCperpendicular to its velocityDzeroA charged particle is moving along a magnetic field line. The magnetic force on the particle is
Aalong its velocityBopposite to its velocityCperpendicular to its velocityDzero
A particle having mass m and charge q is released from the origin in a region in which electric field and magnetic field are given by
→B=−B0→Jand→E=E0→K.
Find the speed of the particle as a function of its z-coordinate.
View SolutionA particle having mass m and charge q is released from the origin in a region in which electric field and magnetic field are ginen by
B=+B0ˆj and →E=+E0ˆi.
Find the speed of the particle as a function of its X-coordinate.
View SolutionThe magnetic force on a charged particle is always perpendicular to its velocity. Can the magnetic force change the velocity of the particle?Speed of the particle?
View SolutionIf a charged particle at rest experiences no electromagnetic force, then electric field in that region
View SolutionForce acting on a moving charged particle in a region of electric and magnetic fields is called
View Solution