Prove that the frequency of oscillation of an electric dipole of moment p and rotational inertia $I$ for small amplitudes about its equilibrium position in a uniform electric field strength E is $\frac{1}{2 π} \sqrt{(\frac{p E}{I})}$

Video Solution

Text Solution

Verified by Experts

Let an electric dipole (charge q and -q at a distance 2a apart) placed in a uniform external electric field of strength E.

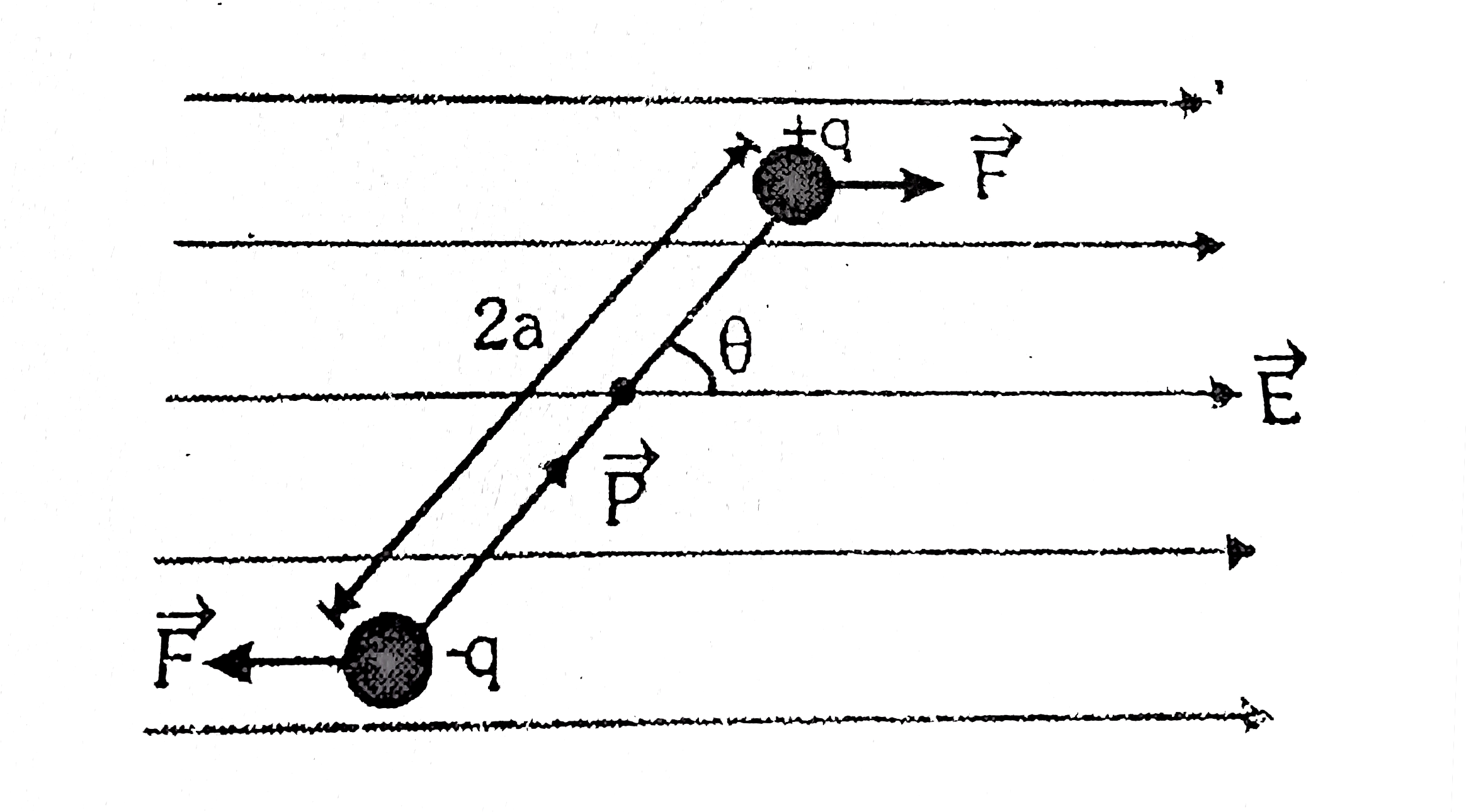
Restoring torque on dipole $τ = - p E sin θ = - p E θ (as θ is small)$
Here -ve sign shows the restoring tendendency of torque $∵ τ = I α ∴$ angular acceleration $= α = \frac{τ}{I} = \frac{P E}{I} θ$
For SHM $α = - ω^{2} θ$ comparing we get $ω = \sqrt{\frac{p E}{I}}$
Thus frequency of oscillations of dipole $n = \frac{ω}{2 π} = \frac{1}{2 π} \sqrt{(\frac{p E}{I})}$

Updated on:21/07/2023

The frequency of oscillation of an electric dipole moment having dipole moment p and rotational inertia l, oscillating in a uniform electric field E is given
A $(1 / 2 π) \sqrt{I / p E}$
B $(1 / 2 π) \sqrt{p E / I}$
C $(2 π) \sqrt{p E / I}$
D $(2 π) \sqrt{I / p E}$
The work in rotating electric dipole of dipole moment p in an electric field E through an angle $θ$ from the direction of electric field, is:
A $p E (1 - cos θ)$
B $p E$
Czero
D $- p E cos θ$
An electric dipole of dipole moment p is placed in a uniform external electric field E. Then, the
Atoeque experienced by the dipole is $E \times p$
Btorque is zero, if p is perpendicular to E
Ctorque is maximum, if p is perpendicular to E
Dpotential energy is maximum, if p is parallel to E