The momentum of a moving particle given by p=tlnt. Net force acting on this particle is defined by equation F=dpdt. The net force acting on the particle is zero at time
A
t=0
B
t=1e
C
t=1e2
D
None of these
Video Solution
Text Solution
The correct Answer is:B
∵p=tlnt
∴F=dpdt=ddt(tlnt)=(1)lnt+(t)(1t)=1+lnt
F=0⇒1+lnt=0⇒lnt=−1⇒t=e−1=1e
|
Topper's Solved these Questions
- MISCELLANEOUSBOOK - ALLENCHAPTER - MISCELLANEOUSEXERCISE - Exersice-03
7 Videos
- MISCELLANEOUSBOOK - ALLENCHAPTER - MISCELLANEOUSEXERCISE - ASSERTION-REASON
18 Videos
- MISCELLANEOUSBOOK - ALLENCHAPTER - MISCELLANEOUSEXERCISE - Part -II Example Some worked out Examples
1 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLENCHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-IV
8 Videos
Similar Questions
Knowledge Check
If net external force acting on the system is zero then
AVelocity of the individual particle of the system will remain constantBVelocity of the center of mass of the system will remain constantCVelocity of the center of mass of the system will be zeroDVelocity of the individual particle of the system will be zeroOne of the forces acting on the particle is conservative, then
A(a) Its work is zero when the particle moves exactly once around any closed path.B(b) Its work equals the change in the kinetic energy of the particle.C(c) It does not obey Newton's second law.D(d) Its work depends on the end points of the motion, not on the path in between.If the net magnetic force acting on a loop is zero then
Ano torque acts on loopBloop performs translational motion.Cboth A and BDtorque
The potential energy of a particle varies with distance x as shown in the graph. The force acting on the particle is zero at
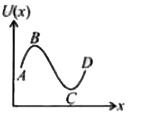
View SolutionThe net force acting is not zero on
View SolutionThe displacement time curve of a particle is shown in the figure. The external force acting on the particle is :
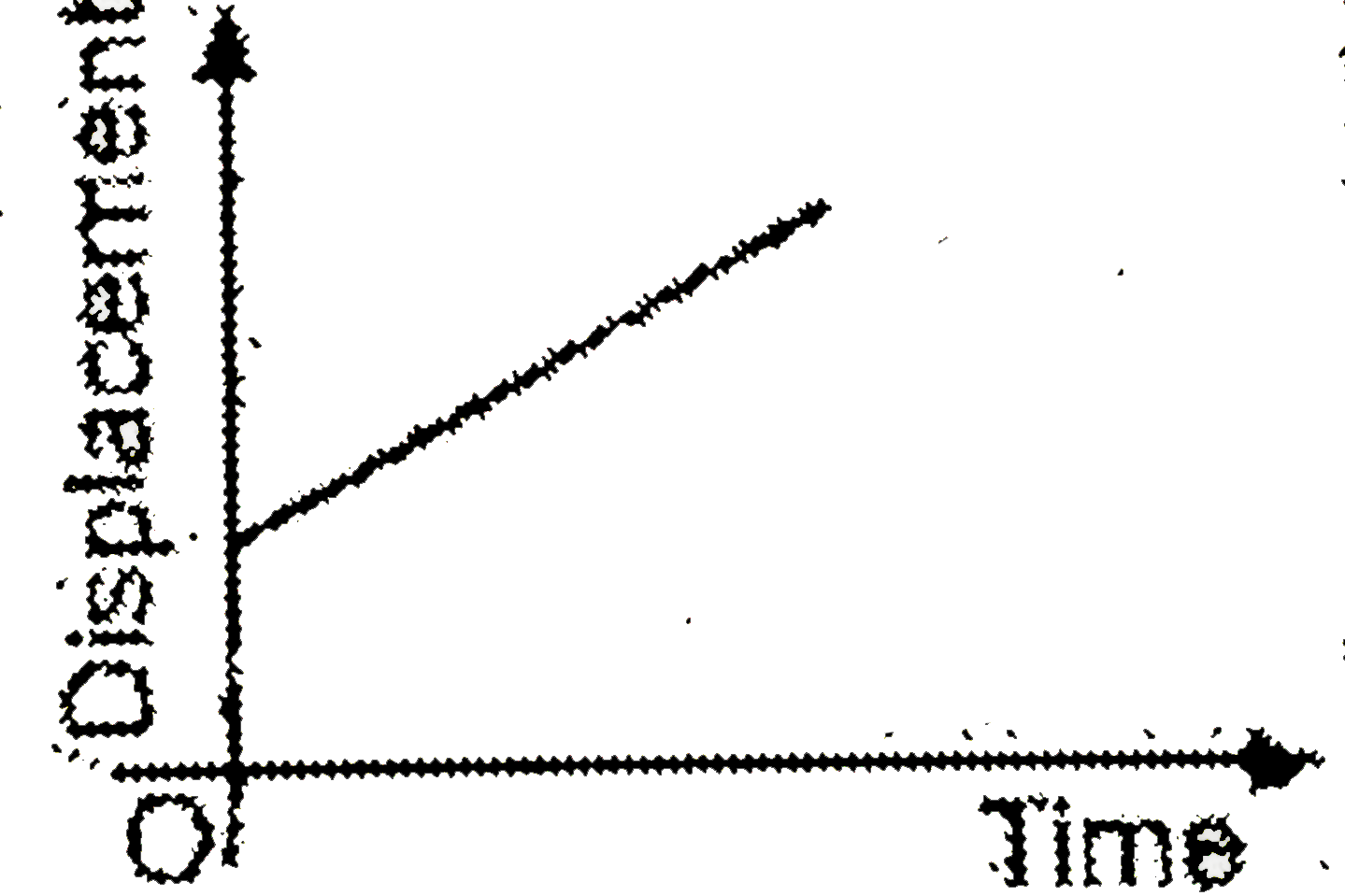
View SolutionA particle is simultaneously acted by two forces equal to 4N and 3N. The net force on the particle is
View SolutionFigure shows the displacement of a particle going along the X-axis as a function of time. The force acting on the particle is zero in the region

View Solution
